Prove that a diameter AB of a circle bisects all those chords which are parallel to the tangent at the point A.
Given, AB is a diameter of the circle.
A tangent is drawn from point A. Draw a chord CD parallel to the tangent MAN.
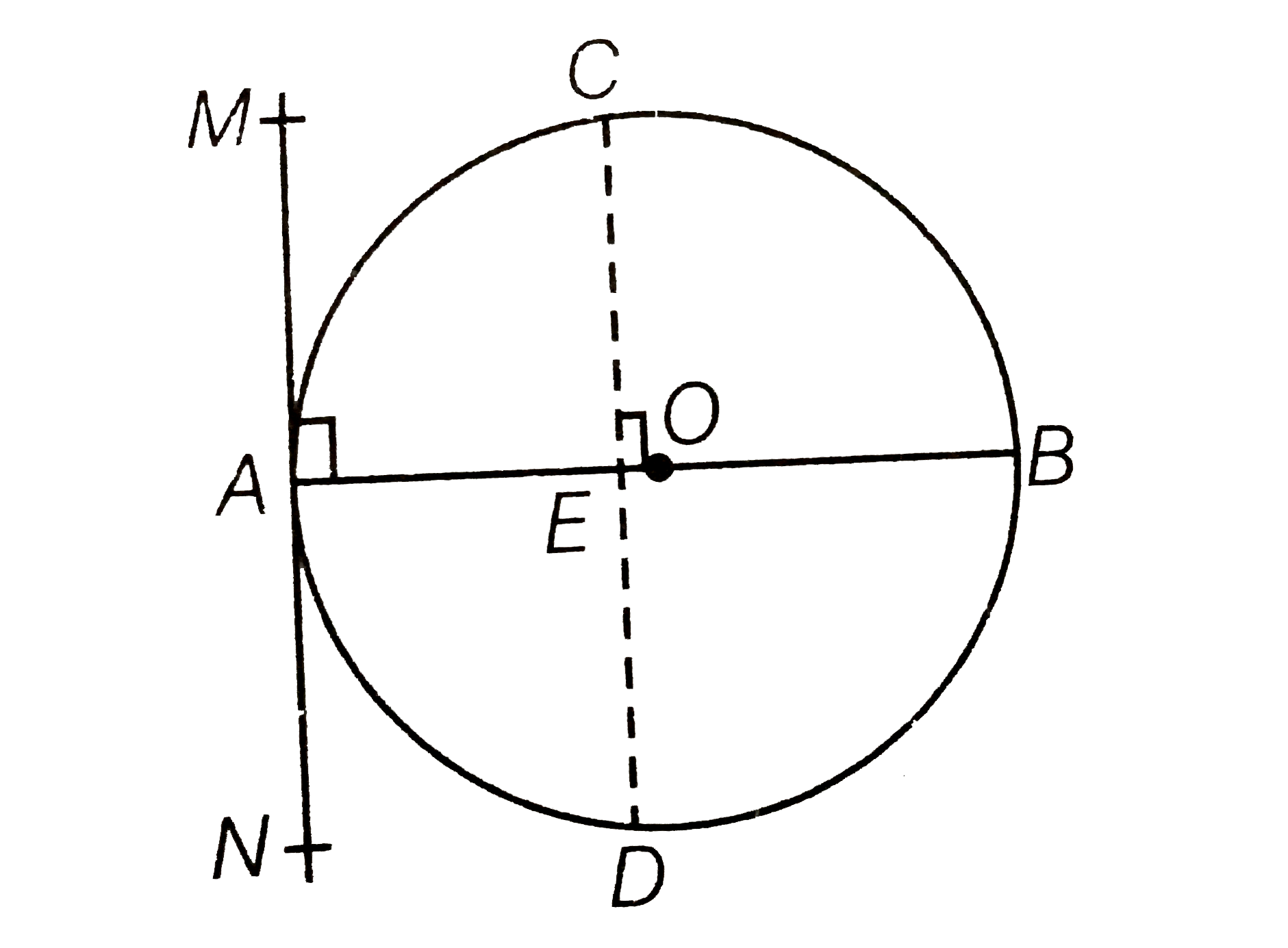
So, CD is a chord of the circle and OA is a radius of the circle.
∠MAO=90∘
[tangent at any point of a circle is perpendicular to the radius through the point of contact]
∠CEO=∠MAO [corresponding angles]
∴∠CEO=90∘
Thus, OE bisects CD, [perpendicular from centre of circle to the radius through the point of contact]
Similarly, the diameter AB bisects all. Chords which are parallel to the tangent at the point A.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The tangents are drawn at the extremities of diameter AB of a circle with centre P. If a tangent to the circle at the point C Intersects the other two tangents at Q and R, then the measure of the ∠QPR is
A45∘B60∘C90∘D180∘
If a diameter of a circle bisects each of the two chords of a circle, prove that the chords are parallel.
View SolutionIf a diameter of a circle bisects each of the two chords of a circle, prove that the chords are parallel.
View SolutionAB is a diameter of a circle. CD is a chord parallel to AB and 2CD=AB. The tangent at B meets the line AC produced at E then AE is equal to -
View SolutionAB is a diameter of a circle. CD is a chord parallel to AB and 2CD = AB. The tangent at B meets the line AC produced at E then AE is equal to
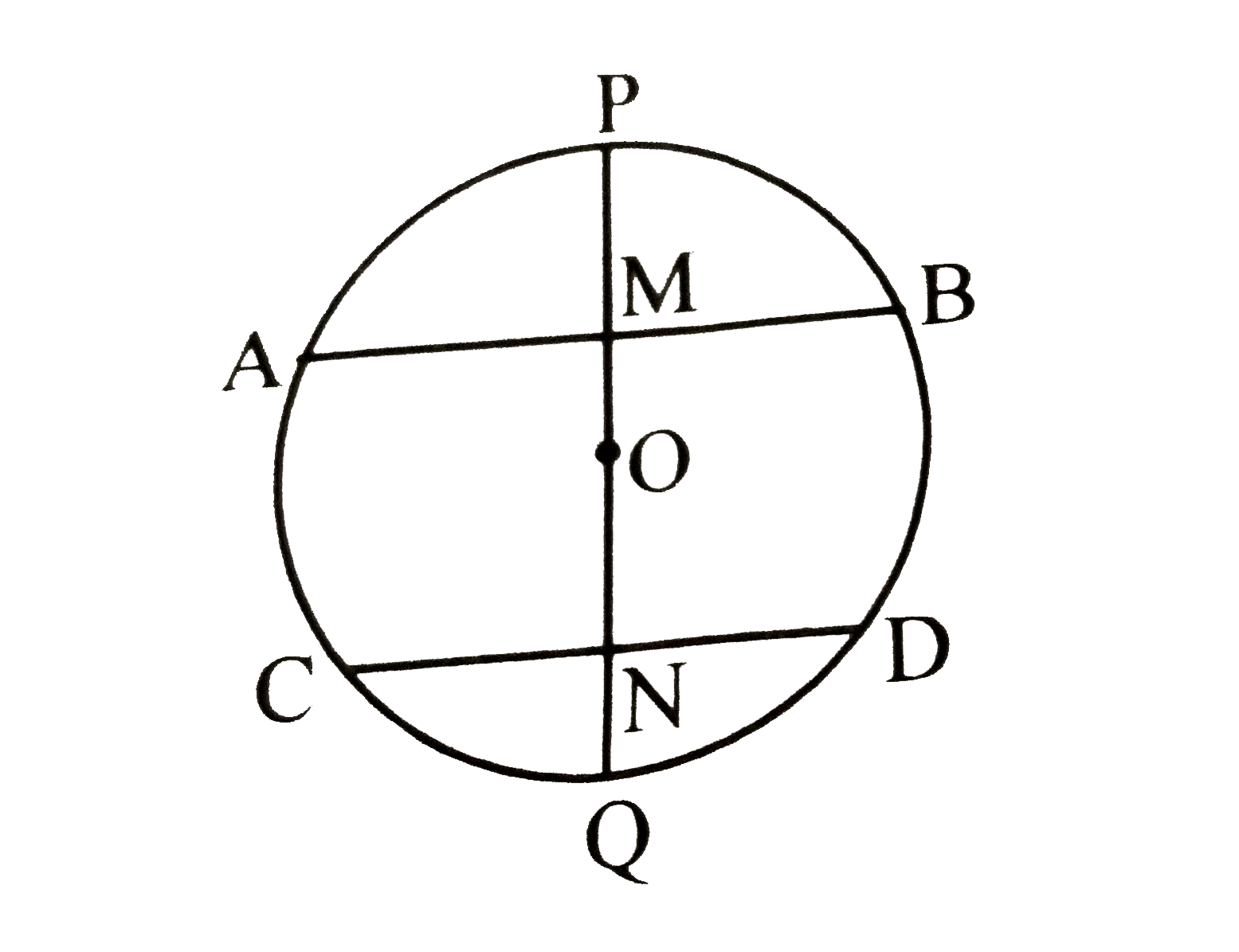
View SolutionIn the given figure, a diameter PQ of a circle bisects the chord RS at the point O. If PS is parallel to RQ, prove that RS is also a diameter of the circle.
View SolutionCircle described on the focal length as diameter touches the tangent at the vertex
View SolutionProve that the right bisector of a chord of a circle, bisects the corresponding arc or the circle.
View Solution