At what minimum acceleration should a monkey slide a rope whose breaking strength is 23rd of its weight?
2g3
g
g3
zero
The correct Answer is:C
To find the minimum acceleration at which a monkey can slide a rope whose breaking strength is 23 of its weight, we can follow these steps:
Step 1: Define the variables
Let:
- m = mass of the monkey
- g = acceleration due to gravity
- Weight of the monkey, W=mg
- Maximum tension in the rope, Tmax=23W=23mg
Step 2: Write the equation of motion for the monkey
When the monkey is sliding down the rope, the forces acting on it are:
- Weight acting downward: mg
- Tension acting upward: T
Using Newton's second law, we can write the equation of motion:
mg−T=ma
where a is the acceleration of the monkey.
Step 3: Substitute the maximum tension
For the minimum acceleration, we will consider the maximum tension in the rope:
T=Tmax=23mg
Substituting this into the equation of motion gives:
mg−23mg=ma
Step 4: Simplify the equation
Now, simplify the left-hand side:
mg−23mg=13mg
Thus, we have:
13mg=ma
Step 5: Solve for acceleration a
Now, divide both sides by m (assuming m≠0):
13g=a
Conclusion
The minimum acceleration a at which the monkey can slide down the rope is:
a=g3
Final Answer
The minimum acceleration required for the monkey to slide down the rope is g3.
---
Let:
- m = mass of the monkey
- g = acceleration due to gravity
- Weight of the monkey, W=mg
- Maximum tension in the rope, Tmax=23W=23mg
When the monkey is sliding down the rope, the forces acting on it are:
- Weight acting downward: mg
- Tension acting upward: T
mg−T=ma
where a is the acceleration of the monkey.
For the minimum acceleration, we will consider the maximum tension in the rope:
T=Tmax=23mg
mg−23mg=ma
Now, simplify the left-hand side:
mg−23mg=13mg
Thus, we have:
13mg=ma
Now, divide both sides by m (assuming m≠0):
13g=a
The minimum acceleration a at which the monkey can slide down the rope is:
a=g3
The minimum acceleration required for the monkey to slide down the rope is g3.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Objective Question
32 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Comprehension
9 Videos
- LAWS OF MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF MOTIONEXERCISE - Assertion And Reason
28 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEY ENGLISHCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
Knowledge Check
With what minimum acceleration can a fireman slide down a rope whose breaking strength is (2/3) of his weight?
Ag2B23gC32gDg3
A sphere is accelerated upwards with the help of a cord whose braking strength is five times its weight. The maximum acceleration with which the sphere can move up without cord breaking is :-
View SolutionA monkey is descending from the branch of a tree with a constant acceleration. If the breaking strength of the branch is 75% of the weight of the monkey, the minimum acceleration with which the monkey can slide down without breaking the branch is
View SolutionA stone is accelerated upwards by a cord whose breaking strength is three times the weight of the stone. The maximum acceleration with which the stone can be moved up without breaking the cord is
View SolutionA fireman wants to slide down a rope. The breaking load for the rope is 3/4th of the weight of the man. With what minimum acceleration sholud the fireman slide down? Acceleration due to gravity is g.
View SolutionA body of mass 60 kg is holding a vertical rope . The rope can break when a mass of 75 kg is suspended from it. The maximum acceleration with which the boy can climb the rope without breaking it is :(g=10m/s2)
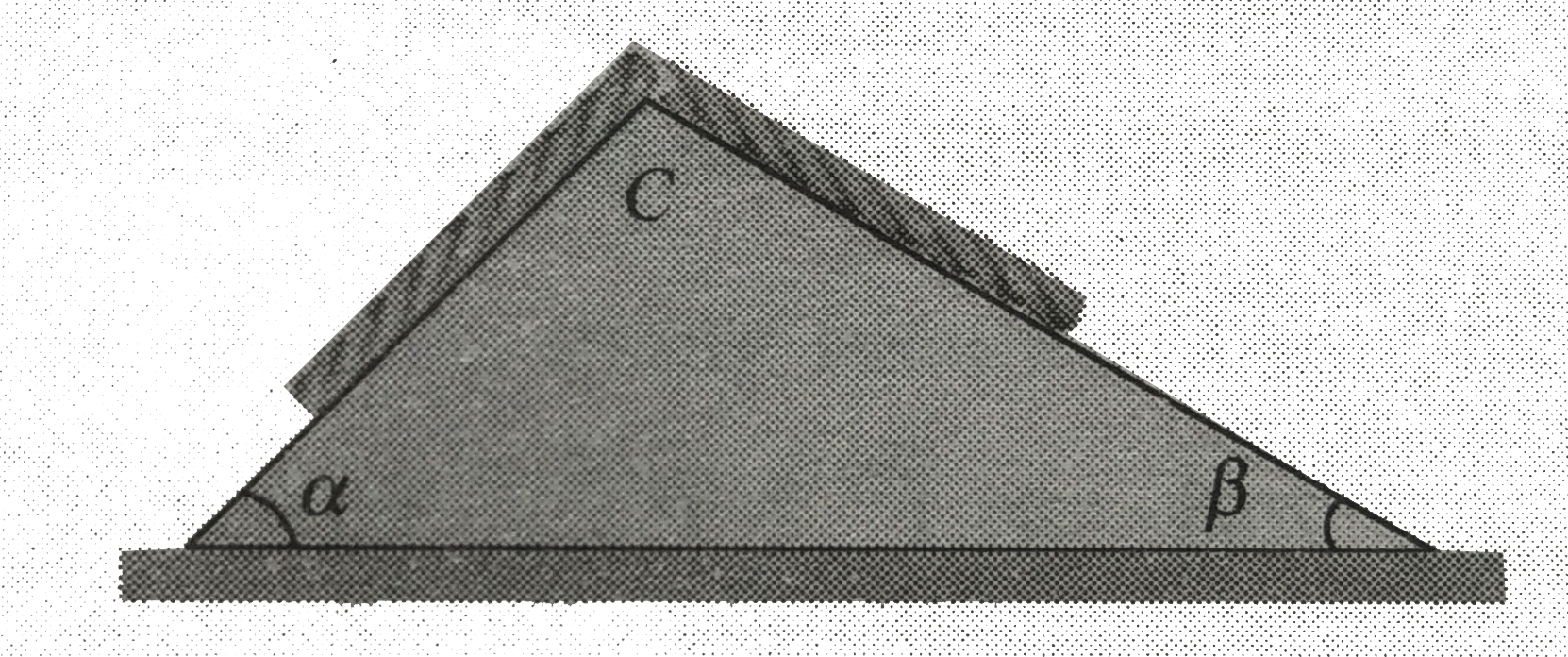
View SolutionA homogeneous flexible rope rests on a wedge whose side edges make angles α and β with the horizontal. The central part of the rope lies on the upper rib C of the wedge. With what acceleration should the wedge be pulled to the left along the horizontal plane in order to prevent the displacement of the rope with respect to the wedge? [Consider all surfaces to be smooth]
View SolutionA man slides down a light rope whose breaking strength in η times his weight (η<1). What should be his maximum acceleration so that the rope just breaks ?
View Solution
