Question

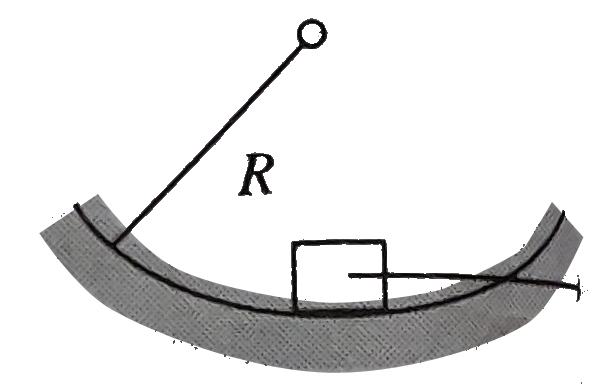
A particle is placed at rest inside a hollow hemisphere of radius $R$. The coefficient of friction between the particle and the hemisphere is $\mu =\frac{1}{\sqrt{3}}$. The maximum height up to which the particle can remain stationary is

Options

A. `(R )/(2)`
B. `(1 - sqrt(3)/(2)) R`
C. `sqrt(3)/(2) R`
D. `(3 R)/(8)`

Answer

B

Explanation

To solve the problem, we need to find the maximum height $$H$$ up to which a particle can remain stationary inside a hollow hemisphere of radius $$R$$ with a coefficient of friction $$\mu =\frac{1}{\sqrt{3}}$$.

1. Understanding the Geometry:
- The particle is placed inside a hollow hemisphere of radius \( R \).
- The maximum height \( H \) is the vertical distance from the base of the hemisphere to the point where the particle can remain stationary.

2. Identifying the Angle of Friction:
- The coefficient of friction \( \mu \) is given as \( \frac{1}{\sqrt{3}} \).
- The angle of friction \( \theta \) can be calculated using the relation:
\( \tan \theta = \mu = \frac{1}{\sqrt{3}} \)
- This gives us:
\( \theta = 30^\circ \)

3. Setting Up the Geometry:
- When the particle is at height \( H \), it makes an angle \( \theta \) with the vertical.
- The angle between the radius at the point of contact and the vertical line is \( 30^\circ \).

4. Using Trigonometry:
- We can denote the horizontal distance from the center of the hemisphere to the particle as \( a \).
- From the geometry of the triangle formed, we can express the relationship between \( H \), \( a \), and \( R \):
\( R = H + a \)
- The angle at the point of contact with the hemisphere is \( 60^\circ \) (since \( 90^\circ - 30^\circ = 60^\circ \)).

5. Finding the Relationship Using Sine:
- In the triangle formed, we can use the sine function:
\( \sin(60^\circ) = \frac{a}{R} \)
- Therefore, we can express \( a \) as:
\( a = R \sin(60^\circ) = R \cdot \frac{\sqrt{3}}{2} \)

6. Substituting Back to Find Height:
- Now substitute \( a \) back into the equation \( R = H + a \):
\( R = H + R \cdot \frac{\sqrt{3}}{2} \)
- Rearranging gives us:
\( H = R - R \cdot \frac{\sqrt{3}}{2} = R \left(1 - \frac{\sqrt{3}}{2}\right) \)

7. Final Expression for Maximum Height:
- Thus, the maximum height \( H \) up to which the particle can remain stationary is:
\( H = R \left(1 - \frac{\sqrt{3}}{2}\right) \)