A block of mass is placed on a rough horizontal force plane. A time dependent horizontal force acts on the block. Here . The frictional force between the block and plane at time is a) 4 N b) 8 N c) 12 N d) 10 N
The correct Answer is:A
To solve the problem, we will follow these steps:
Step 1: Identify the given values
- Mass of the block, m=4kg
- Coefficient of friction, μ=0.2
- Time, t=2s
- Constant k=2N/s
Step 2: Calculate the normal force
The normal force N acting on the block is equal to the weight of the block, which can be calculated using the formula:
N=mg
Where g (acceleration due to gravity) is approximately 10m/s2.
Substituting the values:
N=4kg×10m/s2=40N
Step 3: Calculate the maximum frictional force
The maximum frictional force fmax can be calculated using the formula:
fmax=μN
Substituting the values:
fmax=0.2×40N=8N
Step 4: Calculate the applied force at t=2s
The applied force F is given by the equation:
F=kt
Substituting the values:
F=2N/s×2s=4N
Step 5: Compare the applied force with the maximum frictional force
Now we compare the applied force with the maximum frictional force:
- Applied force F=4N
- Maximum frictional force fmax=8N
Since the applied force (4 N) is less than the maximum frictional force (8 N), the block will not move.
Step 6: Determine the frictional force
In this case, the frictional force f will be equal to the applied force because the block does not move:
f=F=4N
Final Answer
The frictional force between the block and the plane at time t=2s is 4N.
- Mass of the block, m=4kg
- Coefficient of friction, μ=0.2
- Time, t=2s
- Constant k=2N/s
The normal force N acting on the block is equal to the weight of the block, which can be calculated using the formula:
N=mg
Where g (acceleration due to gravity) is approximately 10m/s2.
N=4kg×10m/s2=40N
The maximum frictional force fmax can be calculated using the formula:
fmax=μN
Substituting the values:
fmax=0.2×40N=8N
The applied force F is given by the equation:
F=kt
Substituting the values:
F=2N/s×2s=4N
Now we compare the applied force with the maximum frictional force:
- Applied force F=4N
- Maximum frictional force fmax=8N
In this case, the frictional force f will be equal to the applied force because the block does not move:
f=F=4N
The frictional force between the block and the plane at time t=2s is 4N.
Topper's Solved these Questions
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Objective Question
Explore 32 Videos
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Comprehension
Explore 9 Videos
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Assertion And Reason
Explore 28 Videos
- KINEMATICS 1Book:DC PANDEY ENGLISHChapter:KINEMATICS 1Exercise:INTEGER_TYPE
Explore 15 Videos
- LAWS OF THERMODYNAMICSBook:DC PANDEY ENGLISHChapter:LAWS OF THERMODYNAMICSExercise:Level 2 Subjective
Explore 18 Videos
Similar Questions
A block of mass 4kg is placed on a rough horizontal plane A time dependent force F=kt2 acts on the block where k=2N/s2 Coefficient of friction μ=0.8 force of friction between the block and the plane at t=2s is
View SolutionA block is placed on a rough horizontal plane. A time dependent horizontal force F=K t acts on the block K is a postive constant. Accelration-time graph of the block is
View Solution
Knowledge Check
A block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the block will be.
A10ms−2B5ms−2C15ms−2D0.5ms−2

When F = 2N, the frictional force between 10 kg block 5 kg block is
View SolutionA block of mass 20kg is placed on a rough horizontal plane and a horizontal force of 12N is applied. If coefficient of friction is 0.1 the frictional force acting on it is,
View SolutionA block is placed on a rough horizontal plane. Three horizontalforces are applied on the block as shown in the figure. If the block is in equilibrium, find the friction force acting on the block.
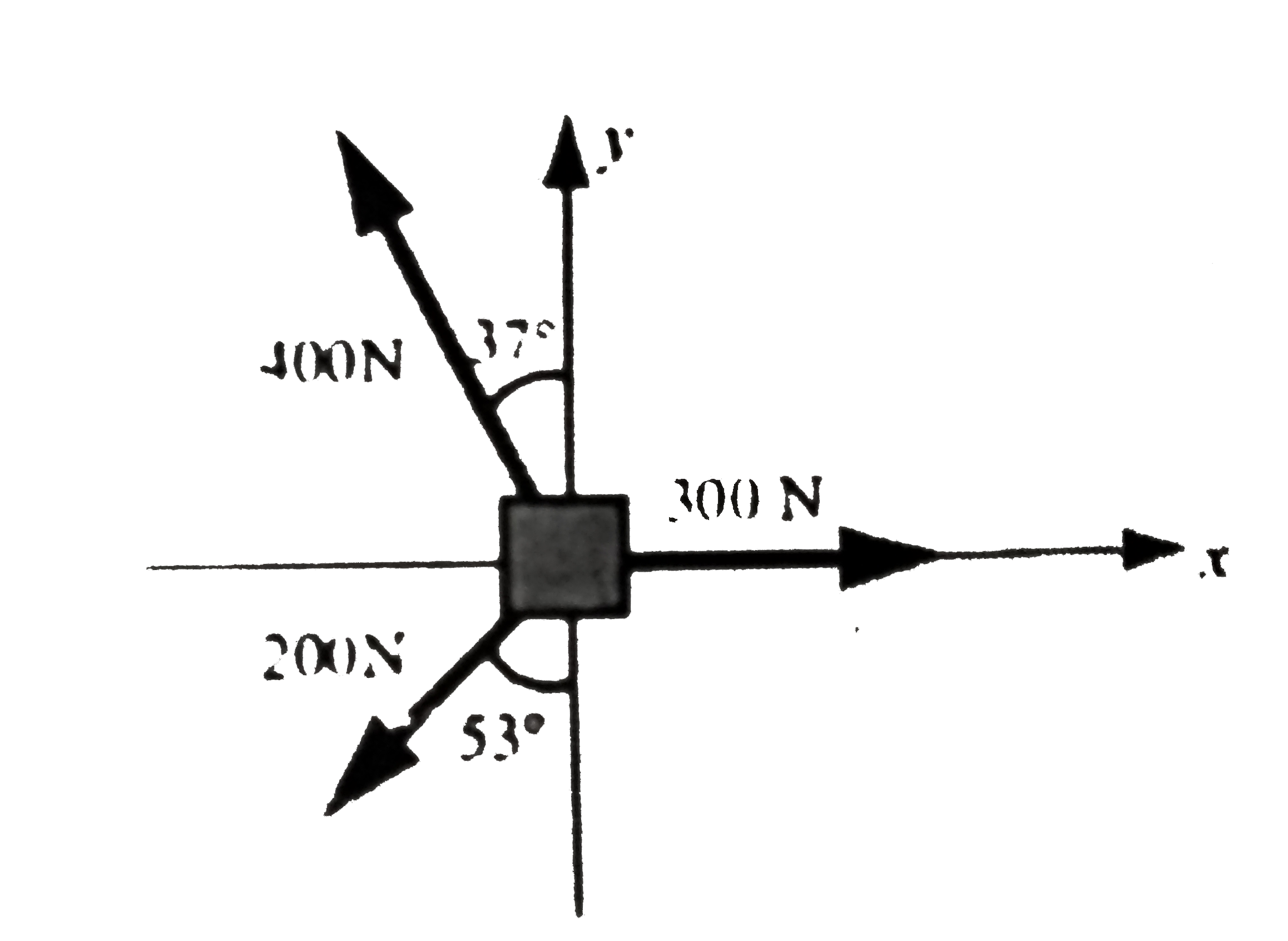
View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the will be.
View SolutionA block of mass 10 kg is placed on a rough horizontal surface of coefficient of friction 0.1. A pulling force of 100 N acts on it at 37∘ with the horizontal. The frictional force acting on the body is ..... N.
View SolutionA block of mass 10kg is placed on a rough horizontal surface having coefficient of friction μ=0.5 . If a horizontal force of 100N is acting on it, then acceleration of the block will be.
View Solution