A block A of mass 4kg is kept on ground. The coefficient of friction between the block and the ground is 0.8. The external force of magnitude 30N is applied parallel to the ground. The resultant force exerted by the ground on the block is (g=10m/s2) a) 40 N b) 30 N c) zero d) 50 N
40N
30N
zero
50N
The correct Answer is:D
To solve the problem, we will follow these steps:
Step 1: Identify the given values
- Mass of the block, m=4kg
- Coefficient of friction, μ=0.8
- External force applied, F=30N
- Acceleration due to gravity, g=10m/s2
Step 2: Calculate the weight of the block
The weight W of the block can be calculated using the formula:
W=m⋅g
Substituting the values:
W=4kg⋅10m/s2=40N
Step 3: Calculate the normal force
The normal force N acting on the block is equal to its weight when the block is on a horizontal surface:
N=W=40N
Step 4: Calculate the frictional force
The frictional force f can be calculated using the formula:
f=μ⋅N
Substituting the values:
f=0.8⋅40N=32N
Step 5: Compare the applied force and frictional force
The applied force F is 30N, which is less than the frictional force f=32N. Since the applied force is less than the maximum frictional force, the block will not move, and the frictional force will equal the applied force.
Step 6: Determine the resultant force exerted by the ground
The resultant force exerted by the ground on the block consists of two components: the normal force N acting vertically and the frictional force f acting horizontally. Since these two forces are perpendicular to each other, we can find the resultant force R using the Pythagorean theorem:
R=√N2+f2
Substituting the values:
R=√(40N)2+(32N)2
Calculating:
R=√1600+1024=√2624≈51.2N
Step 7: Conclusion
The resultant force exerted by the ground on the block is approximately 51.2N. However, since the options provided are discrete, we round it to 50N, which corresponds to option D.
Final Answer
The resultant force exerted by the ground on the block is 50N (Option D).
---
- Mass of the block, m=4kg
- Coefficient of friction, μ=0.8
- External force applied, F=30N
- Acceleration due to gravity, g=10m/s2
The weight W of the block can be calculated using the formula:
W=m⋅g
Substituting the values:
W=4kg⋅10m/s2=40N
The normal force N acting on the block is equal to its weight when the block is on a horizontal surface:
N=W=40N
The frictional force f can be calculated using the formula:
f=μ⋅N
Substituting the values:
f=0.8⋅40N=32N
The applied force F is 30N, which is less than the frictional force f=32N. Since the applied force is less than the maximum frictional force, the block will not move, and the frictional force will equal the applied force.
The resultant force exerted by the ground on the block consists of two components: the normal force N acting vertically and the frictional force f acting horizontally. Since these two forces are perpendicular to each other, we can find the resultant force R using the Pythagorean theorem:
R=√N2+f2
Substituting the values:
R=√(40N)2+(32N)2
Calculating:
R=√1600+1024=√2624≈51.2N
The resultant force exerted by the ground on the block is approximately 51.2N. However, since the options provided are discrete, we round it to 50N, which corresponds to option D.
The resultant force exerted by the ground on the block is 50N (Option D).
Topper's Solved these Questions
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Objective Question
Explore 32 Videos
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Comprehension
Explore 9 Videos
- LAWS OF MOTIONBook:DC PANDEY ENGLISHChapter:LAWS OF MOTIONExercise:Assertion And Reason
Explore 28 Videos
- KINEMATICS 1Book:DC PANDEY ENGLISHChapter:KINEMATICS 1Exercise:INTEGER_TYPE
Explore 15 Videos
- LAWS OF THERMODYNAMICSBook:DC PANDEY ENGLISHChapter:LAWS OF THERMODYNAMICSExercise:Level 2 Subjective
Explore 18 Videos
Similar Questions
A block of mass 2 kg is at rest on a floor . The coefficient of static friction between block and the floor is 0.54. A horizonatl force of 2.8 N is applied to the block . What should be the frictional force between the block and the floor ? ( take , g=10m/s2)
View SolutionIn the previous problem. The friction force applied by ground on block B is
View Solution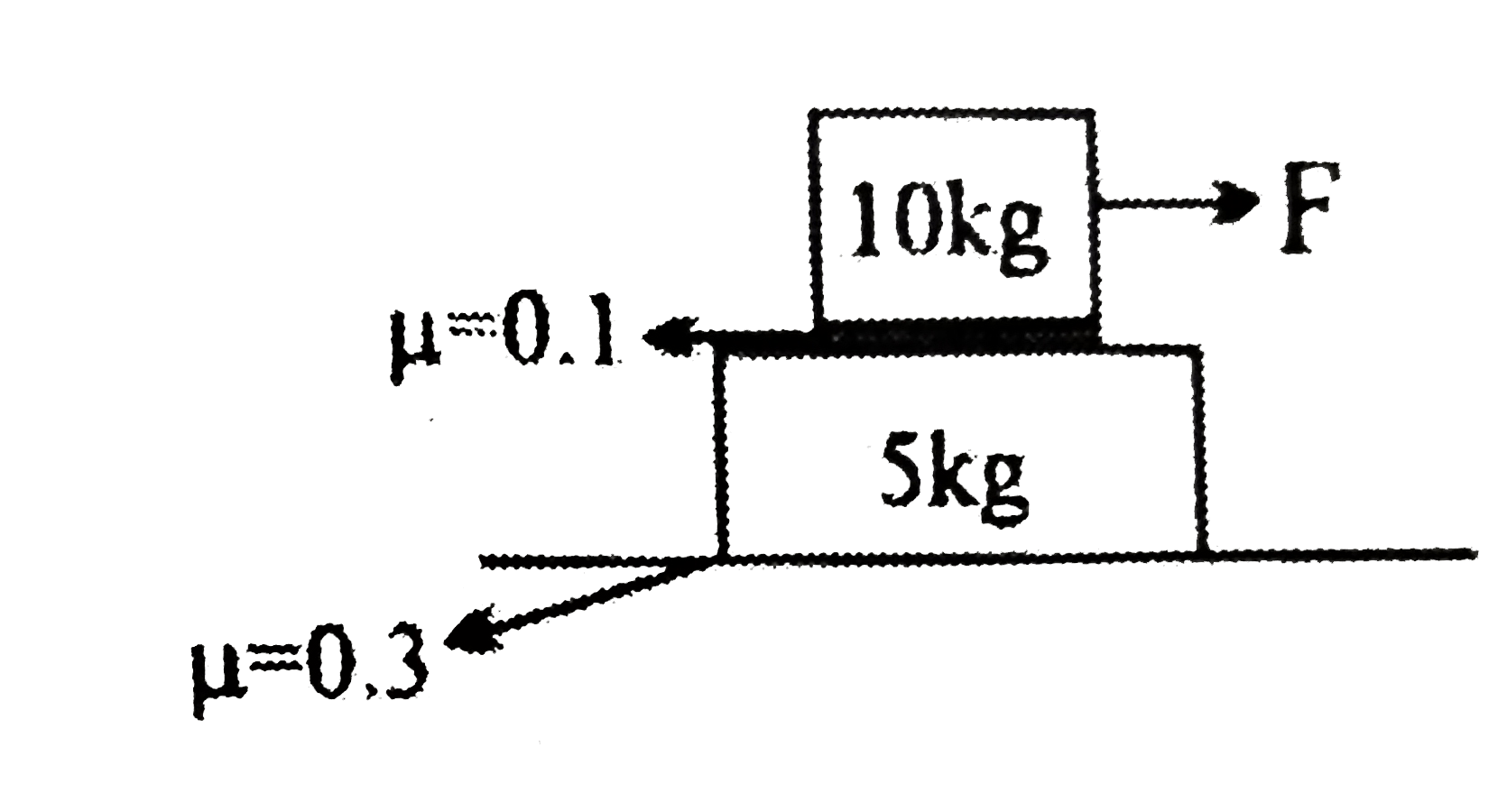
When F = 2N, the frictional force between 5 kg block and ground is
View SolutionA block of mass 4 kg is kept on a rough horizontal surface. The coefficient of static friction is 0.8. If a forace of 19 N is applied on the block parallel to the floor, then the force of friction between the block and floor is
View SolutionTwo blocks of mass 10kg and 30kg are kept as shown in the figure. The surface between the block is fricitional while the ground is smooth. A horizontal force of magnitude 60N is applied horizontally on the upper block. Find the frictional force on block of mass 30kg ?

View SolutionA block of mass m=4kg is placed over a rough inclined plane as shown in fig . The coefficient of friction between the block and the plane μ=0.5 A force F=10N is applied on the block at an angle of 30∘. Find the contact force between the block and the plane.
k

View SolutionA force of 100N is applied on a block of mass 3kg as shown below. The coefficient of friction between wall and the block is 1/4. The friction force acting on the block is :
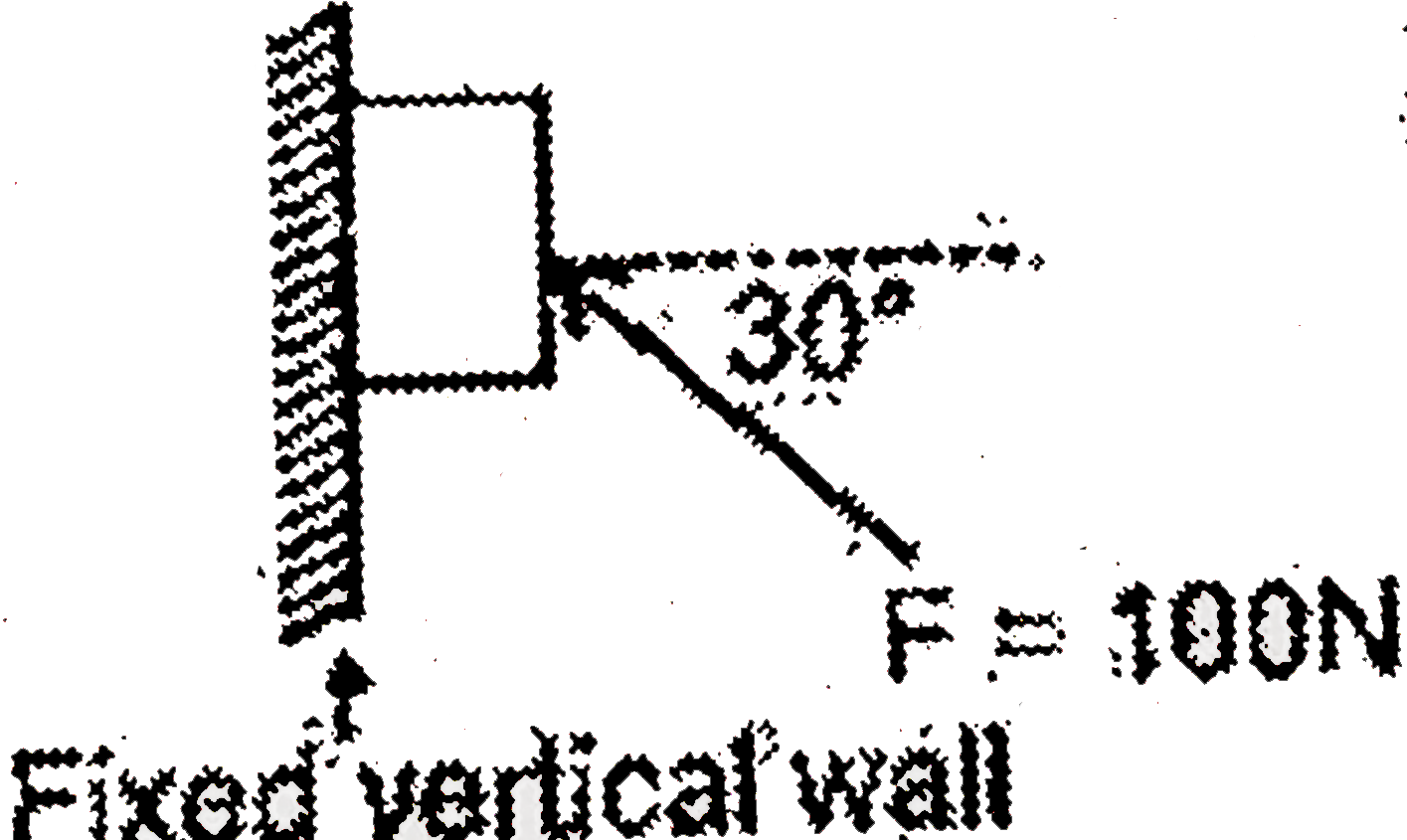
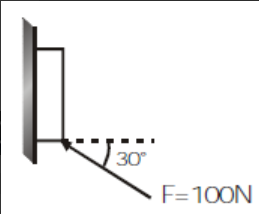
View SolutionA force of 100 N is applied on a block of mass 3 kg as shown in figure. The coefficient of friction between the surface and the block is 14 .Then the friction force acting on the block is
View SolutionA block of mass 20 kg is acted upon by a force F=30N at an angle 53∘ with horizontal in downward direction as shown. The coefficient of friction between the block and the forizontal surface is 0.2. The friction force acting on the block by the ground is (g=10ms2)
View SolutionA block of mass 20 kg is acted upon by a force F=30N at an angle 53∘ with horizontal in downward direction as shown. The coefficient of friction between the block and the forizontal surface is 0.2. The friction force acting on the block by the ground is (g=10ms2)
View Solution