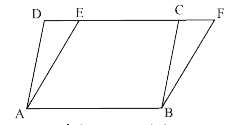
ABCD is a parallelogram and X is the mid-point of AB. $(A X C D) = 24 c m^{2}$ , then $a r (A B C) = 24 c m^{2}$ .

Video Solution

Text Solution

Verified by Experts

False
Given, ABCD is a parallelogram and $a r (A X C D) = 24 c m^{2}$
Let area of parallelogram ABCD is $2 y c m^{2}$ and join AC.

We know that, diagonal divides the area of parallelogram in two equal areas.
$∴$ $a r (Δ A B C) = a r (A C D) = y$ [say]
Also, X is the mid-point of AB.
So, $a r (Δ A C X) = a r (B C X)$ [since, X is median in $Δ A B C$ ]
$= \frac{1}{2} a r (A B C) = \frac{1}{2} y$
Now, ar $(A X C D) = a r (Δ A D C) + a r (A C X)$
$24 = y + \frac{y}{2}$ [given]
$\Rightarrow$ $24 \frac{3 y}{2}$
$\Rightarrow$ $y = \frac{24 \times 2}{3} = 16 c m^{2}$
Hence, $a r (Δ A B C) = 16 c m^{2}$
Therefore, given statement is false.

Updated on:21/07/2023

In parallelogram ABCD, if P is the mid-point of side AB, then
ADP bisects diagonal AC
BDP bisects diagonal BD
CDP trisects diagonal BD
DDP trisects diagonal AC and trisected by AC
In the figure, ABCD and EFGD are two parallelograms and G is the mid-point of CD. Then, $a r (Δ D P C) = a r (E F G D)$ .

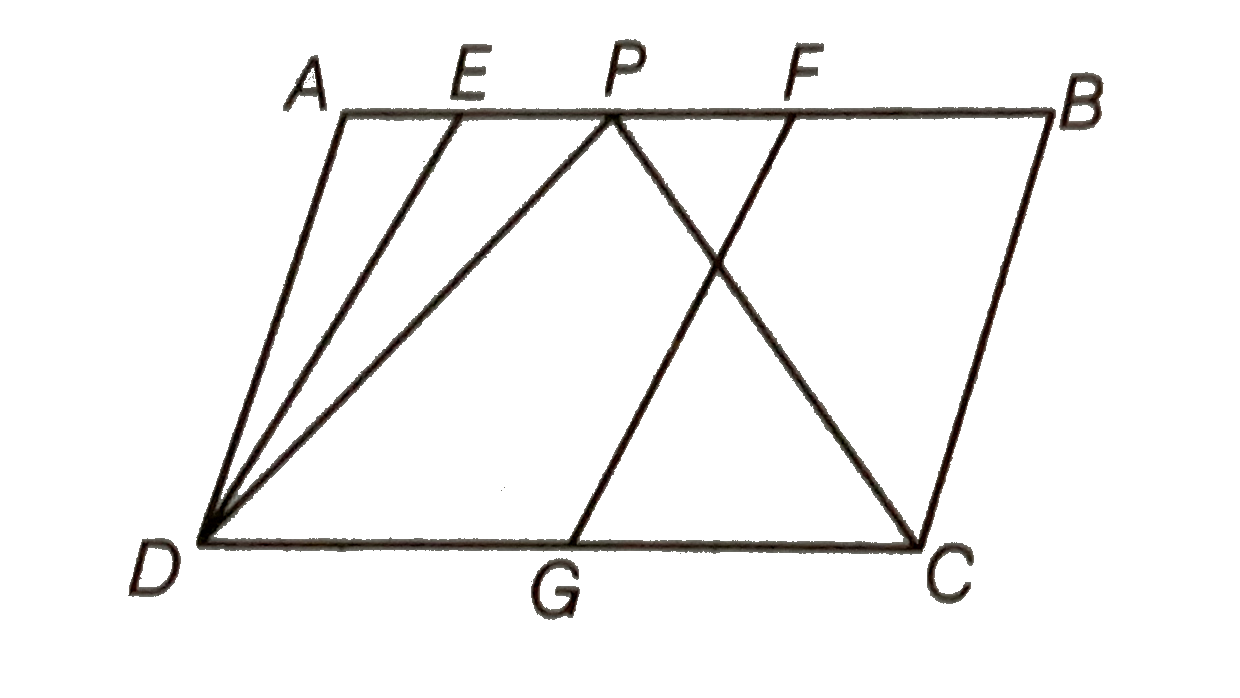ATrue
BFalse
CPartially true
DNone of these
In the given figure, ABCD and ABEF are parallelograms such that ar(quad. EABC) $17 c m^{2}$ and ar(||gm ABCD) $= 25 c m^{2}$ . Then $a r (△ B C F)$ = ?

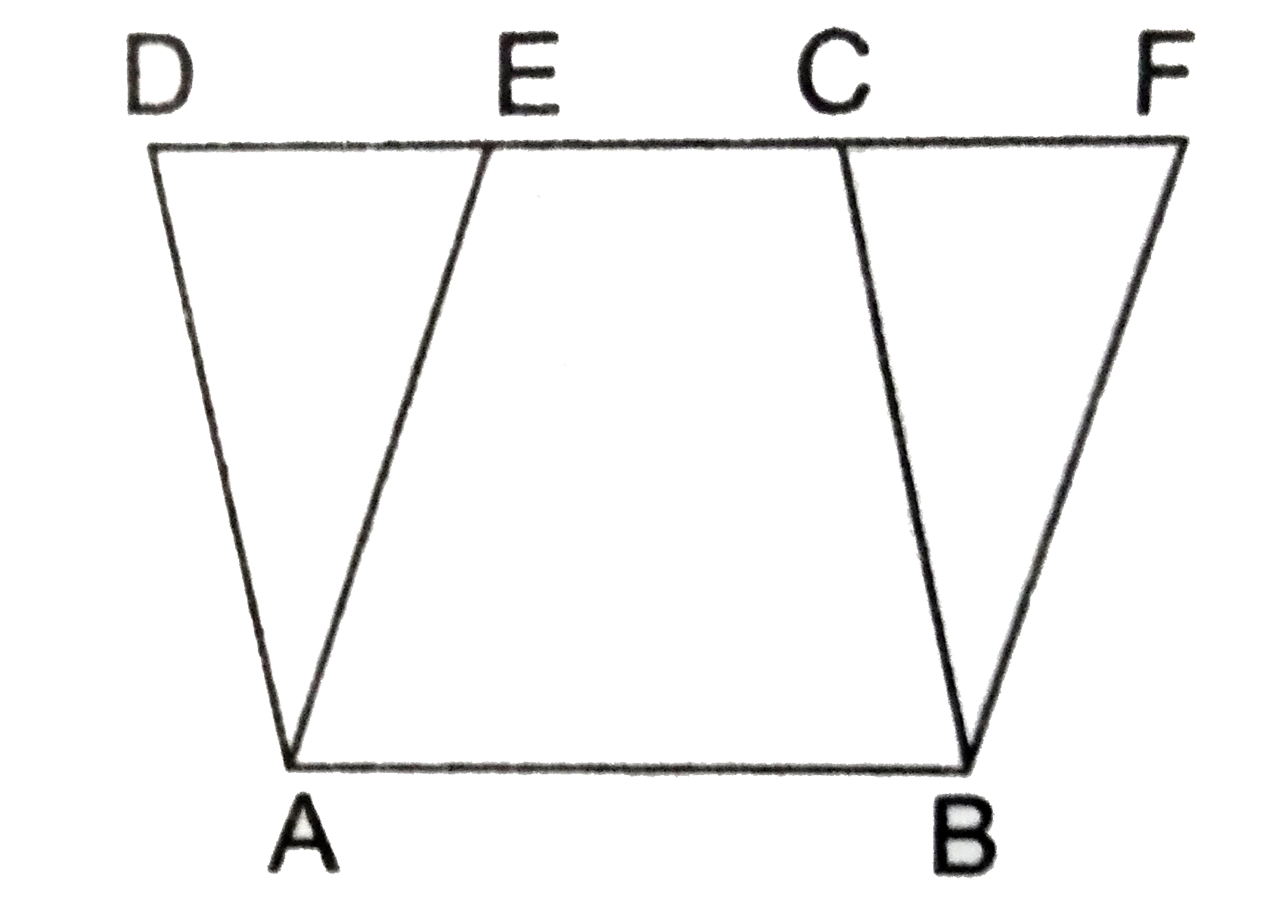A $4 c m^{2}$
B $4.8 c m^{2}$
C $6 c m^{2}$
D $8 c m^{2}$