In a trapezium ABCD, AB || DC, AB = a cm, and DC = b cm. If M and N are the midpoints of the nonparallel sides, AD and BC respectively then find the ratio of ar(DCNM) and ar(MNBA).
a:b
(3a+b):(a+3b)
(a+3b):(3a+b)
(2a+b):(3a+b)
The correct Answer is:B
Given, AB = a cm, DC = b cm and AB|| DC.
Also, E and F are mid-points of AD and BC, respectively.
So, distance between CD, EF and AB, EF will be same say h.
Join BD which intersect EF at M.
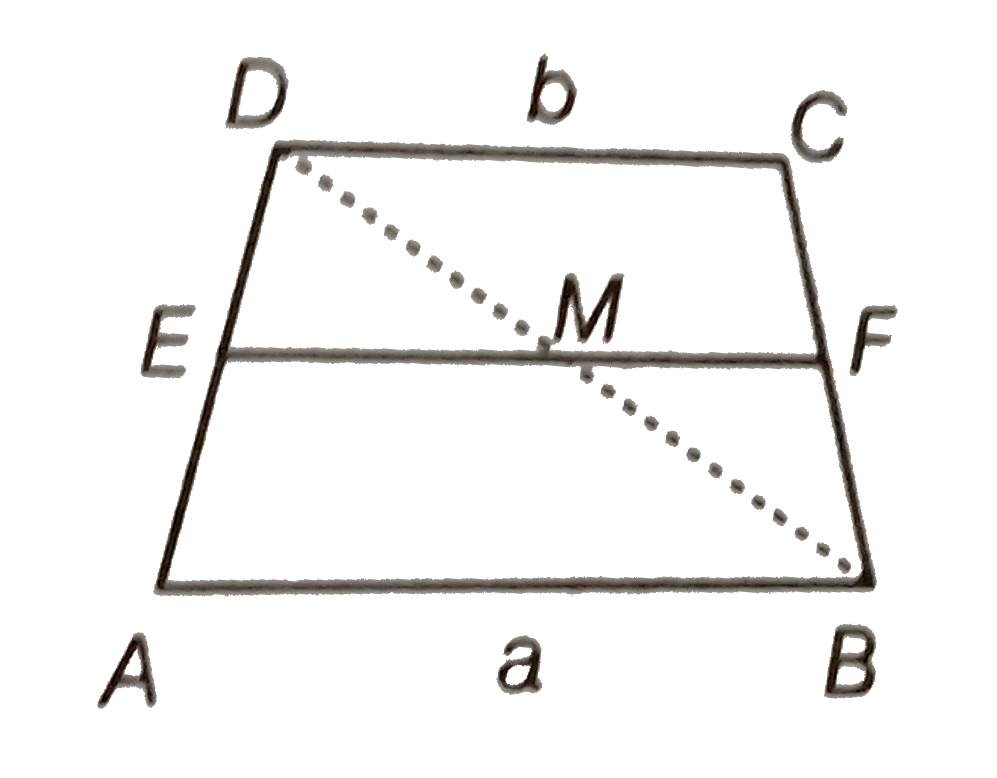
Now, in ΔABD,E is the mid-point of AD and EM || AB
So, M is the mid-point of BD
and EM=12AB [by mid-point theorem] ...(i)
Similarly in ΔCBD , MF=12CD ...(ii)
On adding, Eqs. (i) and (ii), we get
EM+MF=12AB+12CD
⇒ EF=12(AB+CD)=12(a+b)
Now, area of trapezium ABFE=12(sum of parallel sides)×(distance between parallel sides)
=12(a+12(a+b))×h=14(3a+b)h
Now, area of trapezium EFCD =12[b+12(a+b)]×h=14(3b+a)h
∴ Required ratio=Area of ABFEArea of EFCD=14(3a+b)H14(3b+a)H
=(3a+b)(a+3b)or (3a+b):(a+3b)
Topper's Solved these Questions
Similar Questions
Knowledge Check
In figure, if DE||BC, then find the ratio of ar (ΔADE) and ar(DECB).
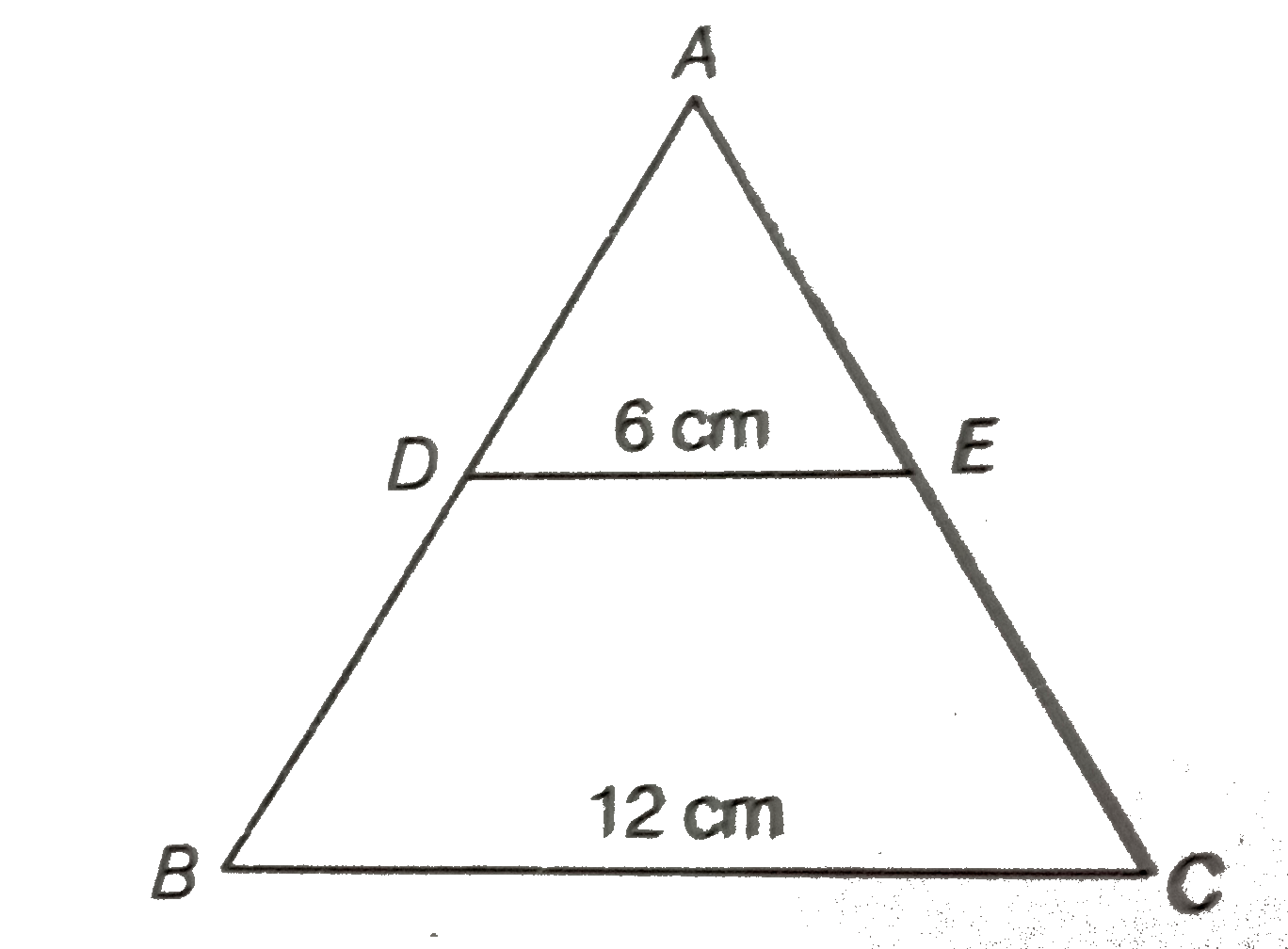 A1:3B1:2C2:5D3:5
A1:3B1:2C2:5D3:5ABCD is a trapezium in which AB || DC a and DC= 40 cm and AB = 60 cm. If X and Y a re respectively, the midpoints of AD and BC, then

XY=A40 cmB50 cmC60 cmD30 cmIn a trapezium ABCD, AB||DC, AB = AD, ∠ADC=64∘and∠BCD=54∘. Find ∠DBC.
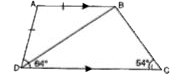 A64∘B72∘C94∘D116∘
A64∘B72∘C94∘D116∘
ABCD is a trapezium with AB || DC. E and F are points on non-parallel sides AD and BC respectively such that EF is parallel to AB. Show thatAEED=BFFC.
View SolutionABCD is a trapezium in which AB || DC, AB = 16 cm and DC = 24 cm. If E and Fare respectively the midpoints of AD and BC, prove that ar(ABFE) = 911 ar(EFCD).
View SolutionABCD is trapezium in which AB∣∣DC, DC = 30 cm and AB = 50 cm. If X and Y are, respectively the mid-points of AD and BC, prove that
ar(DCYX)=79ar(XYBA).
View SolutionIn a trapezium ABCD, DC || AB , AB = 12 cm and DC = 7.2 cm. What is the length of the line segment joining the mid- points of its diagonals?
View SolutionP and Q are any two points lying on the sides DC and AD respectively of a parallelogramABCD. Show that ar (APB) = ar (BQC).
View Solution
