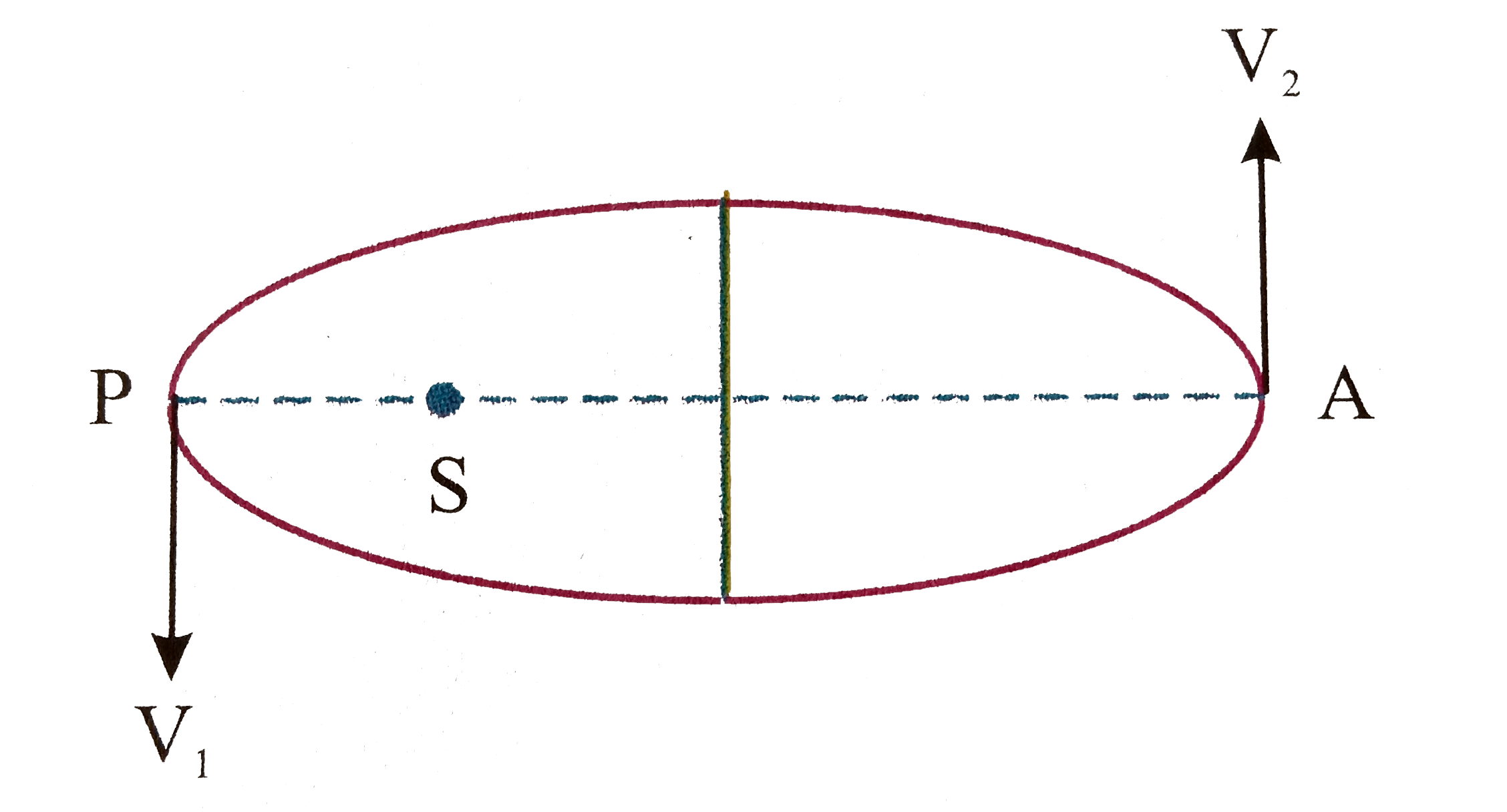
A planet of mass $m$ is moving in an elliptical orbit around the sun of mass $M$ . The semi major axis of its orbit is a, eccentricity is $e$ .
Find total energy of planet interms of given parameters.

A

$- \frac{G M m}{4 a}$

B

$- \frac{G M m^{2}}{2 a}$

C

$- \frac{G M m}{8 a}$

D

$- \frac{G M m}{2 a}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

|

Answer

Step by step video, text & image solution for A planet of mass m is moving in an elliptical orbit around the sun of mass M. The semi major axis of its orbit is a, eccentricity is e. Find total energy of planet interms of given parameters. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS GRAVITATION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One

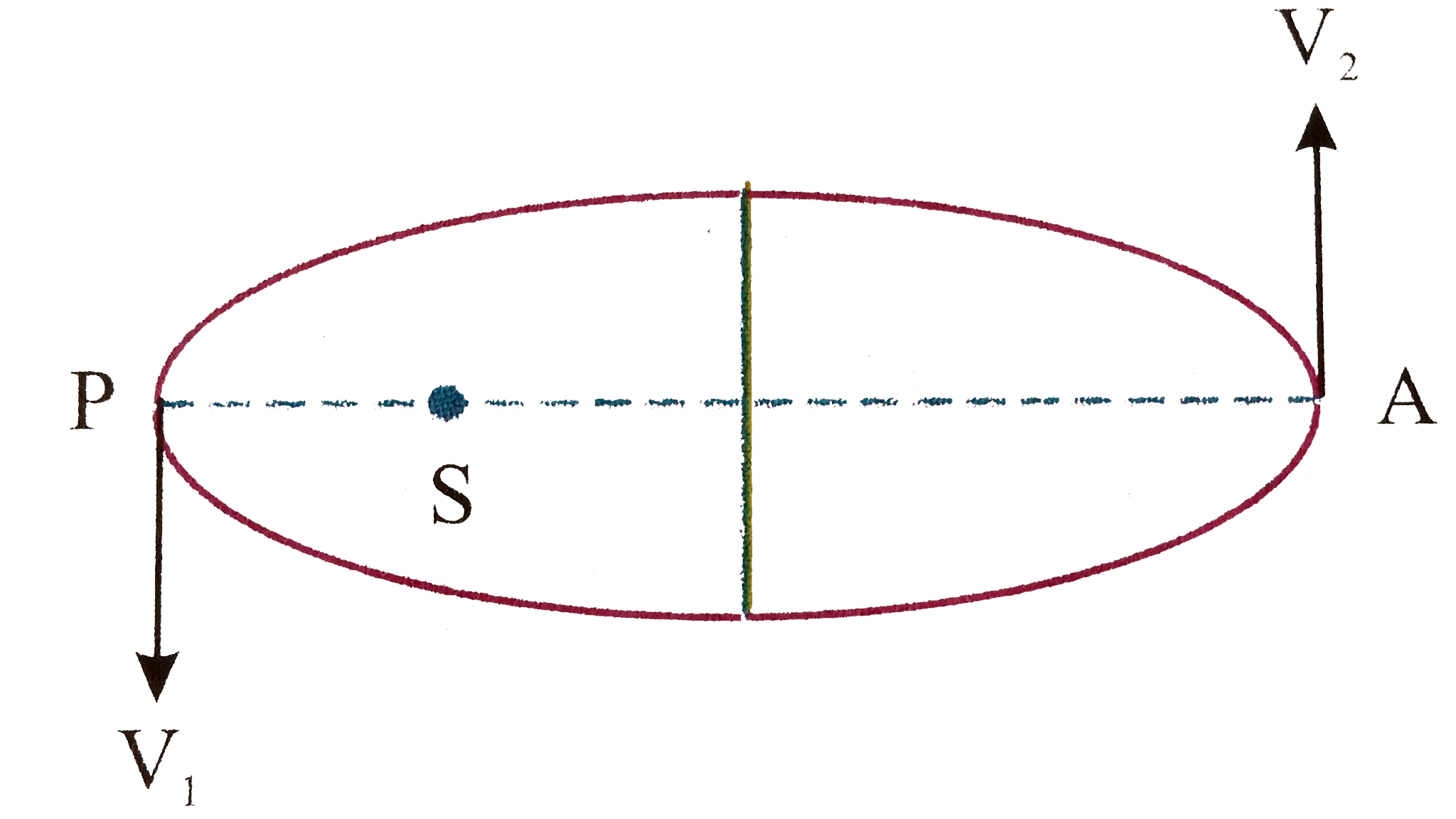
A planet of mass $m$ is moving in an elliptical orbit around the sun of mass $M$ . The semi major axis of its orbit is a, eccentricity is $e$ .
Find speed of planet $V_{1}$ at perihelion $P$
A $\sqrt{\frac{G M}{a} \frac{(1 + e)}{(1 - e)}}$
B $\frac{1 + e}{1 - e} \sqrt{\frac{G M}{a}}$
C $\sqrt{\frac{G M}{a^{3}} \frac{(1 + e)}{(1 - e)}}$
D $\sqrt{\frac{G M}{a^{3}} \frac{(1 + e^{2})}{(1 - e^{2})}}$
Question 2 - Select One

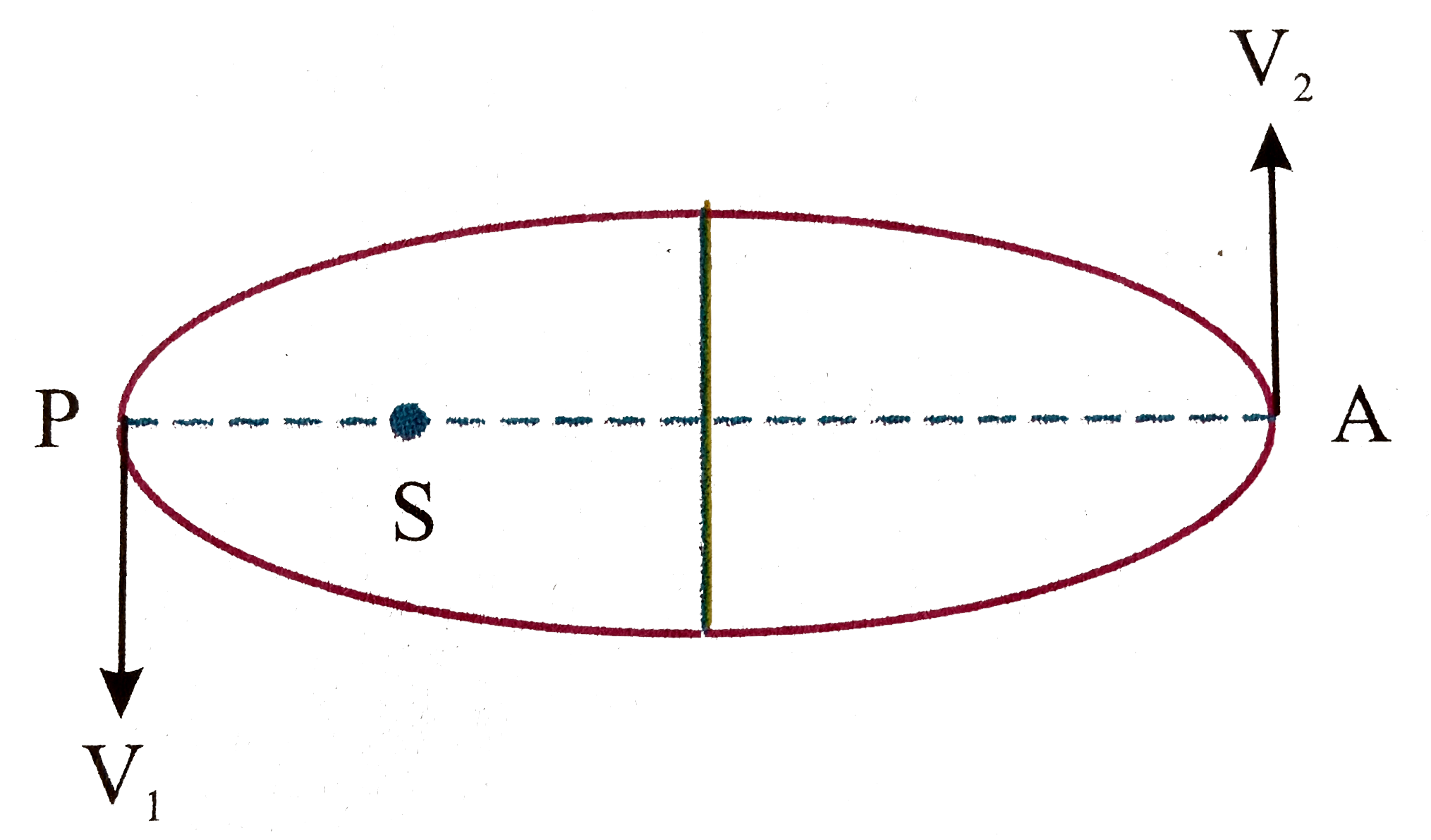
A planet of mass $m$ is moving in an elliptical orbit around the sun of mass $M$ . The semi major axis of its orbit is a, eccentricity is $e$ .
Find speed of planet $V_{2}$ at aphelion $A$ .
A $\sqrt{\frac{G M}{a} \frac{(1 + e)}{(1 - e)}}$
B $\sqrt{\frac{G M}{a} \frac{(1 - e)}{(1 + e)}}$
C $\sqrt{\frac{G M}{a^{3}} \frac{(1 + e^{2})}{(1 - e^{2})}}$
D $\sqrt{\frac{G M}{a^{3}} \frac{(1 - e^{2})}{(1 + e^{2})}}$
Question 3 - Select One
A planet of mass m is moving around the sun in an elliptical orbit of semi-major axis a :
AThe total mechanical energy of the planet is varying periodically with time
BThe total energ of the planet is constant and equals $- \frac{G m M_{s}}{2 a}, N_{s}$ is mass of sun
CTotal mechanical energy of the planet is constant and equals $- \frac{G m M_{s}}{a}, M_{2}$ is mass of sun
DDate is insufficient of arrice at a conclusion

NARAYNA-GRAVITATION-LEVEL-VI

Home
Ask Doubt
Profile