Consider a thin spherical shell of uniform density of mass M and radius R:
The gravitational field inside the shell will be zero
The gravitational self energy of shell is GM22R
Attractive force experience by unit area of the shell pull the other half is GM22R2
Net gravitational force with which one hemisphere of the shell arracts other, is GM28R2
The correct Answer is:A, B, D
F=−GMmR3x
a=−GMR3x
a=−ω2x
ω=√GMR3
V=Rω=R×√GMR3=√GMR
After collision, velocity of ball towards 'A'
VC=e√GMR=0.2√GMR
VC=15√GMR
A1=Vcω=15√GMR√GMR3=R5
∴ Net distance =R+R5+R5
Topper's Solved these Questions
- GRAVITATIONBook:NARAYNAChapter:GRAVITATIONExercise:ILLUSTRATION
Explore 49 Videos
- GRAVITATIONBook:NARAYNAChapter:GRAVITATIONExercise:EVALUATE YOURSELF -1
Explore 3 Videos
- GRAVITATIONBook:NARAYNAChapter:GRAVITATIONExercise:LEVEL-V
Explore 54 Videos
- FRICTIONBook:NARAYNAChapter:FRICTIONExercise:Passage type of questions I
Explore 6 Videos
- KINETIC THEORY OF GASESBook:NARAYNAChapter:KINETIC THEORY OF GASESExercise:LEVEL-III(C.W)
Explore 52 Videos
Similar Questions
A uniform metal sphere of radius R and mass m is surrounded by a thin uniform spherical shell of same mass and radius 4R. The centre of the shell C falls on the surface of the inner sphere. Find the gravitational fields at points A and B.

View SolutionA solid sphere of mass m and radius r is placed inside a hollow thin spherical shell of mass M and radius R as shown in figure−6.30. A particle of mass m' is placed on the line joining the two centres at a distance x from the point of contact of the sphere and the shell. Find the magnitude of the resultant gravitational force on this - particle due to the sphere and the shell if (a) r<x<2r,(b)2r<x<2Rand(c)x>2R.
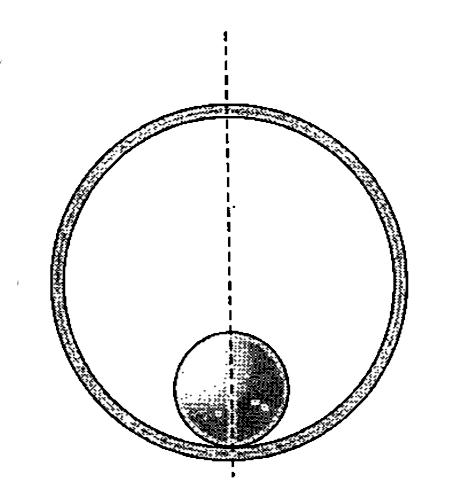
View Solution
Knowledge Check
Consider two solid spherical asteriod of uniform density of mass M and radius R. In one asteroid a tunnel tof very small size of depth R is bored to the centre and in other asteroid a spherical into the cavities of both asteroids from the top most point P. If force experienced by particle is FI and FII
 AThe ratio of FI/FII is equal to 2x/RBThe ratio of FI/FII is equal to x/RCThe ratio of TI/TII is equal to π/4DThe ratio of TI/TII is equal to π/2
AThe ratio of FI/FII is equal to 2x/RBThe ratio of FI/FII is equal to x/RCThe ratio of TI/TII is equal to π/4DThe ratio of TI/TII is equal to π/2Consider a thin uniform spherical layer of mass M and radius R. The potential energy of gravitational interaction of matter forming this shell is :
A−GM2RB−12GM2RC−35GM2RD−23GM2RA small section of area ΔA is removed from a uniform spherical shell with surface mass density σ and radius R as shown in the figure . Find the magnitude of gravitational field intensity at point P due to the remaining mass .
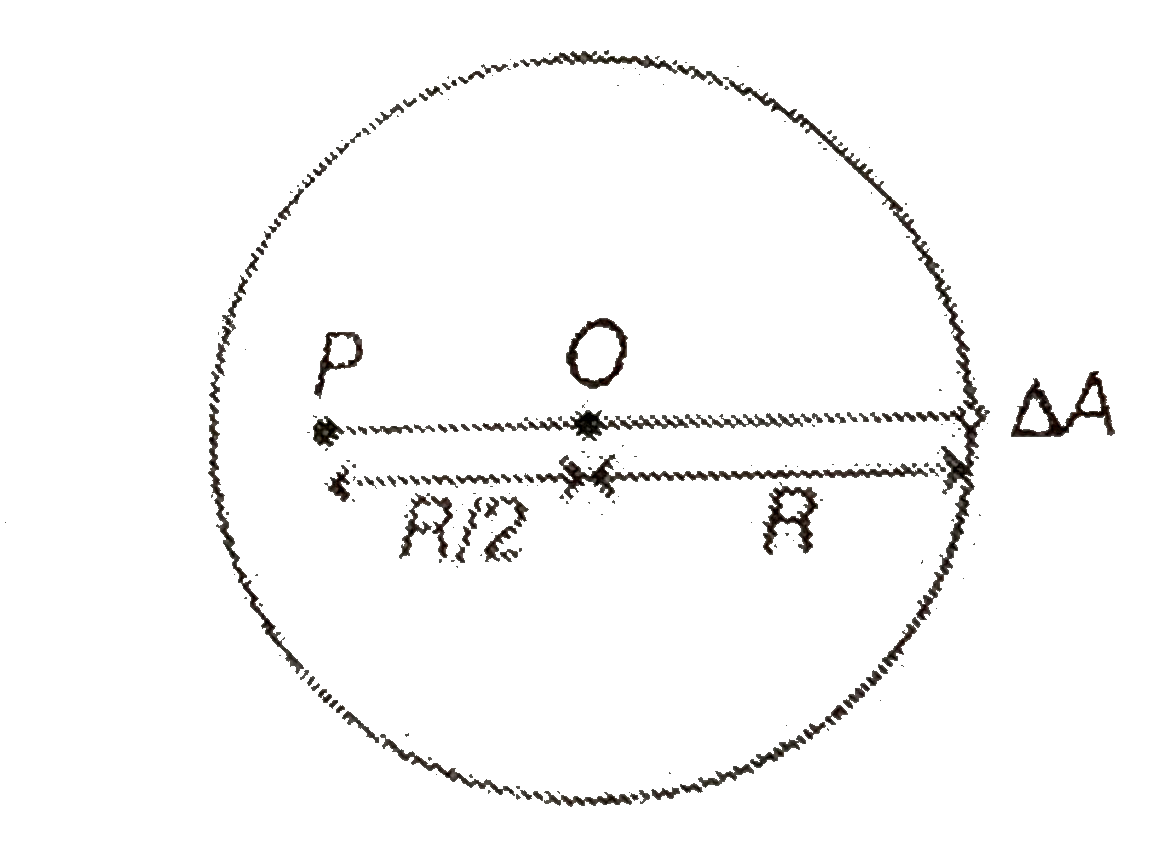 A4σΔAG9R2B4σΔAGR2CσΔAGR2Dzero
A4σΔAG9R2B4σΔAGR2CσΔAGR2Dzero
a solid sphere of mass m and radius r is plced nside as hollow thin spherical shell of mass and M and radius R as shown in figure. A particle of mas m is placed on tehline joining the tow centres ast a distance x from the point of contct of th sphere and teh shell. Findn teh mgnitude fo the resultant gravitational force on this particle due to the sphere and the shell if a. r<x<2r,b.2r<x<2Randc.x>2R.
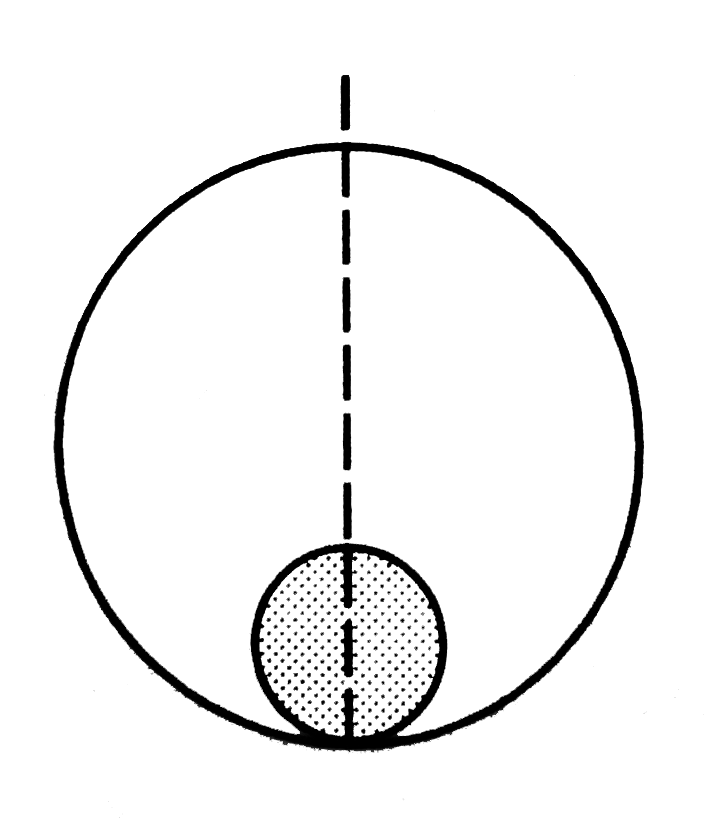
View SolutionTwo uniformly charged non-conducting hemispherical sheels each having uniform charge density σ and radius R form a complete sphere (not stuck together) and surround a concentric spherical conducting shell of radius R/2. If hemispherical parts are in equilibrium then minimum surface charge density of inner conducting shell is :
View SolutionA solid sphere of mass m and radius r is placed inside a hollow thin spherical shell of mass M and radius R as shown in the figure. A particle of mass m' is placed on the line joining the two centres at a distance x from the point of contact of the sphere and the shell. Find the magnitude of the resultant gravitational force on this particle due to the sphere and the shell if

r<x<2r
View SolutionConsider a thin spherical shell of radius R consisting of uniform surface charge density σ. The electric field at a point of distance x from its centre and outside the shell is
View SolutionThe moment of inertia of thin spherical shell of mass M and radius R about a diameter is 23MR. Its radius of gyration K about a tangent will be
View Solution