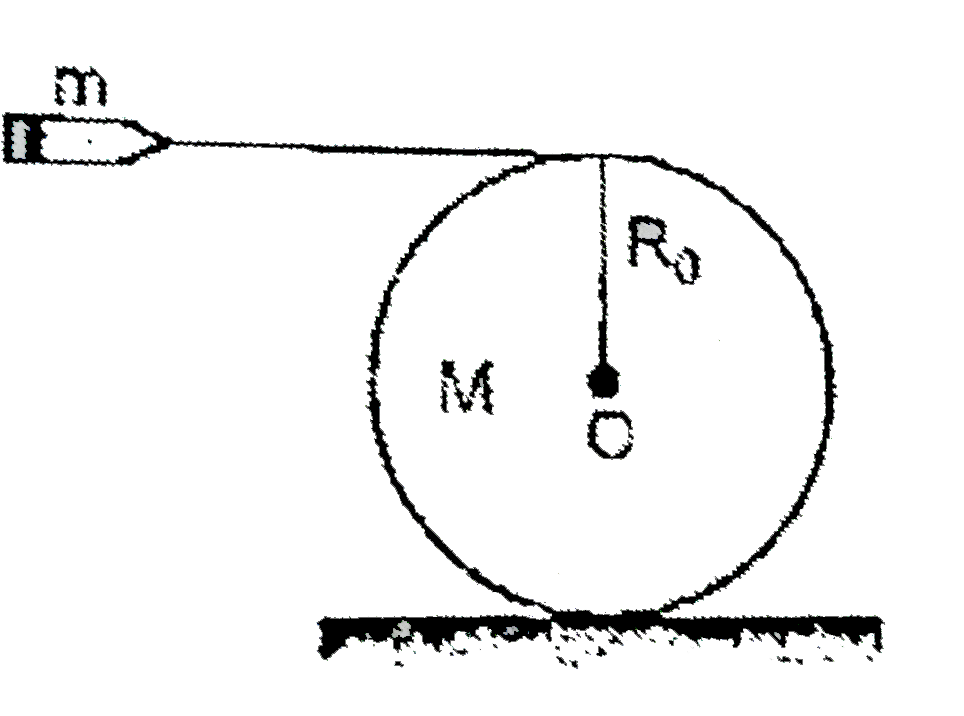
A bullet of mass $m$ moving with a velocity of $u$ just grazes the top of a solid cylinder of mass $M$ and radius $R$ resting on a rough horizontal surface as shown and is embedded in the cylinder after impact. Assuming that the cylinder rolls without slipping, find the angular velocity of the cylinder and the final velocity of the bullet.

Video Solution

Text Solution

Verified by Experts

Let $v$ and $ω$ be the velocity of the bullet and angular velocity of the cylinder, respectively. Applying onservation of angular momentum about the point of contact of the cylinder with the floor we get
$μ 2 R = m v 2 R + (I_{C M} + M R^{2}) ω$

here, $v = 2 R ω$ and $I_{C M} = 1 / 2 M R^{2}$
Substituting and solving, we get
$v = \frac{8 μ}{8 m + 3 M}$
and $ω = \frac{4 μ}{(8 m + 3 M) R}$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
A solid cylinder of mass M and radius R pure rolls on a rough surface as shown in the figure. Choose the correct alternative (s).

AThe acceleration of the centre of mass is $\frac{F}{M}$
BThe acceleration of the centre of mass is $\frac{2}{3} \frac{F}{M}$
CThe friction force on the cylinder acts backward
DThe magnitude of the friction force is $\frac{F}{3}$
Question 2 - Select One
A solid cylinder of mass M and radius R rolls without slipping on a flat horizontal surface. Its moment of inertia about the line of contact is ?
A $\frac{M R^{2}}{2}$
B $M R^{2}$
C $\frac{3}{2} M R^{2}$
D $2 M R^{2}$
Question 3 - Select One
A homogeneous cylinder of mass Mand radius r is pulled on a horizontal plane by a horizontal force F acting through its centre of mass. Assuming rolling without slipping, find the angular acceleration of the cylinder,
A $\frac{2 F}{3 M r}$
B $\frac{3 F}{2 M r}$
C $\frac{F}{3 M r}$
D $\frac{F}{2 M r}$

CENGAGE PHYSICS-RIGID BODY DYNAMICS 2-Exercise 3.3

Home
Ask Doubt
Profile