A small solid marble of mass $M$ and radius $r$ rolls down along the loop track, without slipping. Find the height $h$ above the base, from where it has to start rolling down the incline such that the sphere just completes the vertical circular loop of radius $R$ .

Video Solution

Text Solution

Verified by Experts

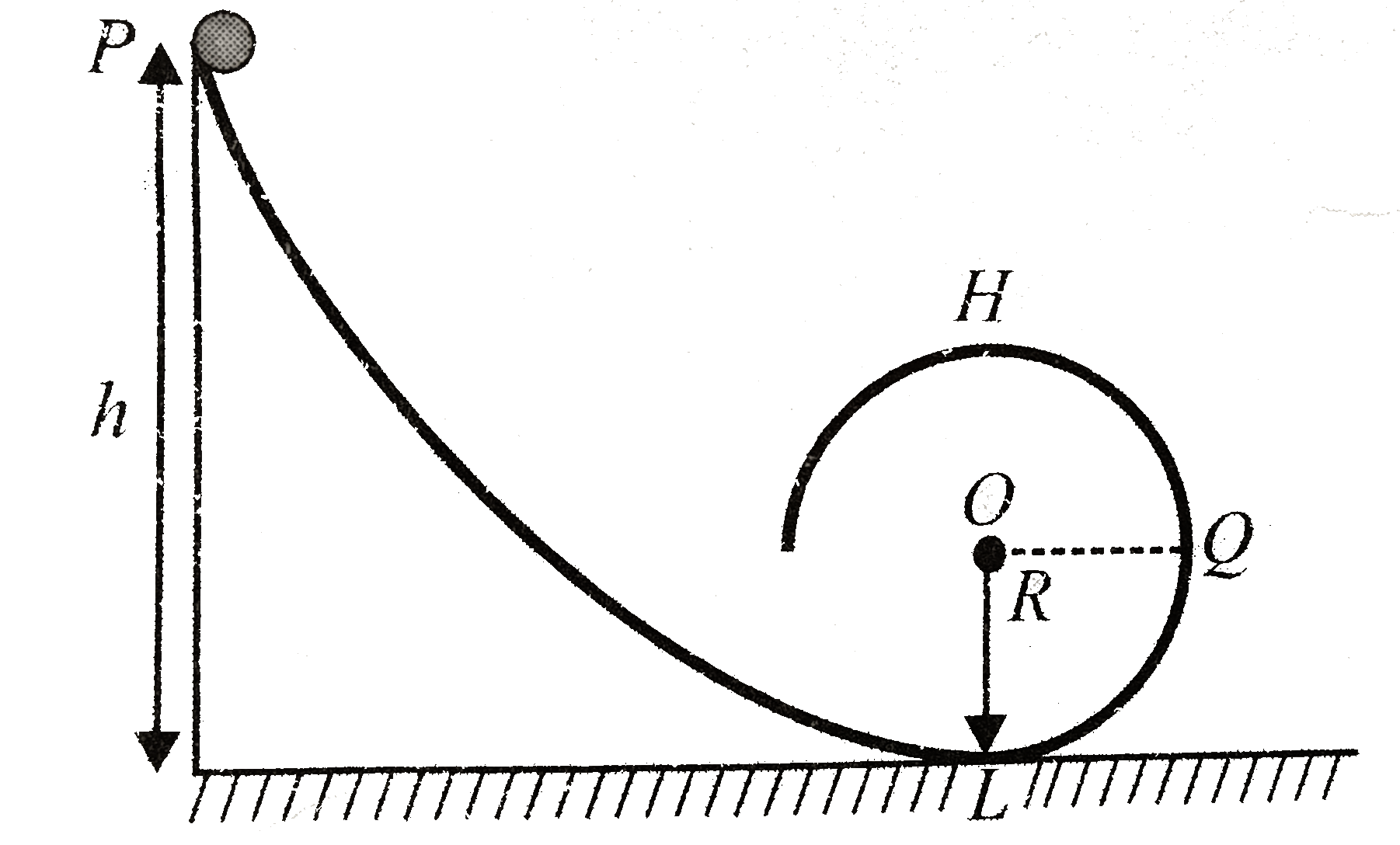
Here the centre of mass of the marble will move in a circle of radius $(R - r)$ so for just looping the loop, at $H$
$v = \sqrt{g (R - r)}$ ……i
Now as in roling
$K = K_{T} + K_{R} = \frac{1}{2} M v^{2} + \frac{1}{2} ω^{2}$
and here
$I = (\frac{2}{5}) M r^{2}$ with $v = r ω$
so $K = \frac{1}{2} M v^{2} + \frac{1}{2} [\frac{2}{5} M r^{2}] {[\frac{v}{r}]}^{2}$
i.e., $= \frac{1}{2} M v^{2} + \frac{1}{5} M v^{2} = \frac{7}{10} M v^{2}$ .........ii
So in the light of eqn i eqn ii becomes
$K = \frac{7}{10} M g (R - r)$ .....iii
As this kinetic energy is provided by loss in $P E$ , applying conservation of mechanical energy between $P$ and $H$ .
$0 + M g h = \frac{7}{10} m g (R - r) + M g (2 R_{r})$
$h = \frac{1}{10} (27 R - 17 r)$

Updated on:21/07/2023

A small solid sphere of radius $r$ rolls down an incline without slipping which ends into a vertical loop of radius $R$ . Find the height above the base so that it just loops the loop
A $\frac{5}{2} R$
B $\frac{5}{2} (R - r)$
C $\frac{25}{10} (R - r)$
D $\frac{27}{10} R - \frac{17 r}{10}$
A solid cylinder of mass $M$ and radius $R$ rolls down an inclined plane of height $h$ without slipping. The speed of its centre when it reaches the bottom is.
A $\sqrt{\frac{3}{4} g h}$
B $\sqrt{\frac{4}{3} g h}$
C $\sqrt{4 g h}$
D $\sqrt{2 g h}$
A solid cylinder of mass $M$ and radius $R$ rolls down an inclined plane of height $h$ without slipping. The speed of its centre when it reaches the bottom is.
A $\sqrt{2 g h}$
B $\sqrt{\frac{4}{3} g h}$
C $\sqrt{\frac{3}{4}} g h$
D $\sqrt{\frac{4 g}{h}}$