The bob of a pendulum at rest is given a sharp hit to impart a horizontal velocity √10gl where l is the length of the pendulum. Find the tension in the string when a. the string is horizontal. B. The bob is at its highest point and c. the string makes an angle of 600 with the upward vertical.Solution in Tamil
The correct Answer is:A, B, C
a.Let the velocity at B be v2
12mv21=12mv22+mgl
→12m(10gl)=12mv22+mgl
v22=8gl
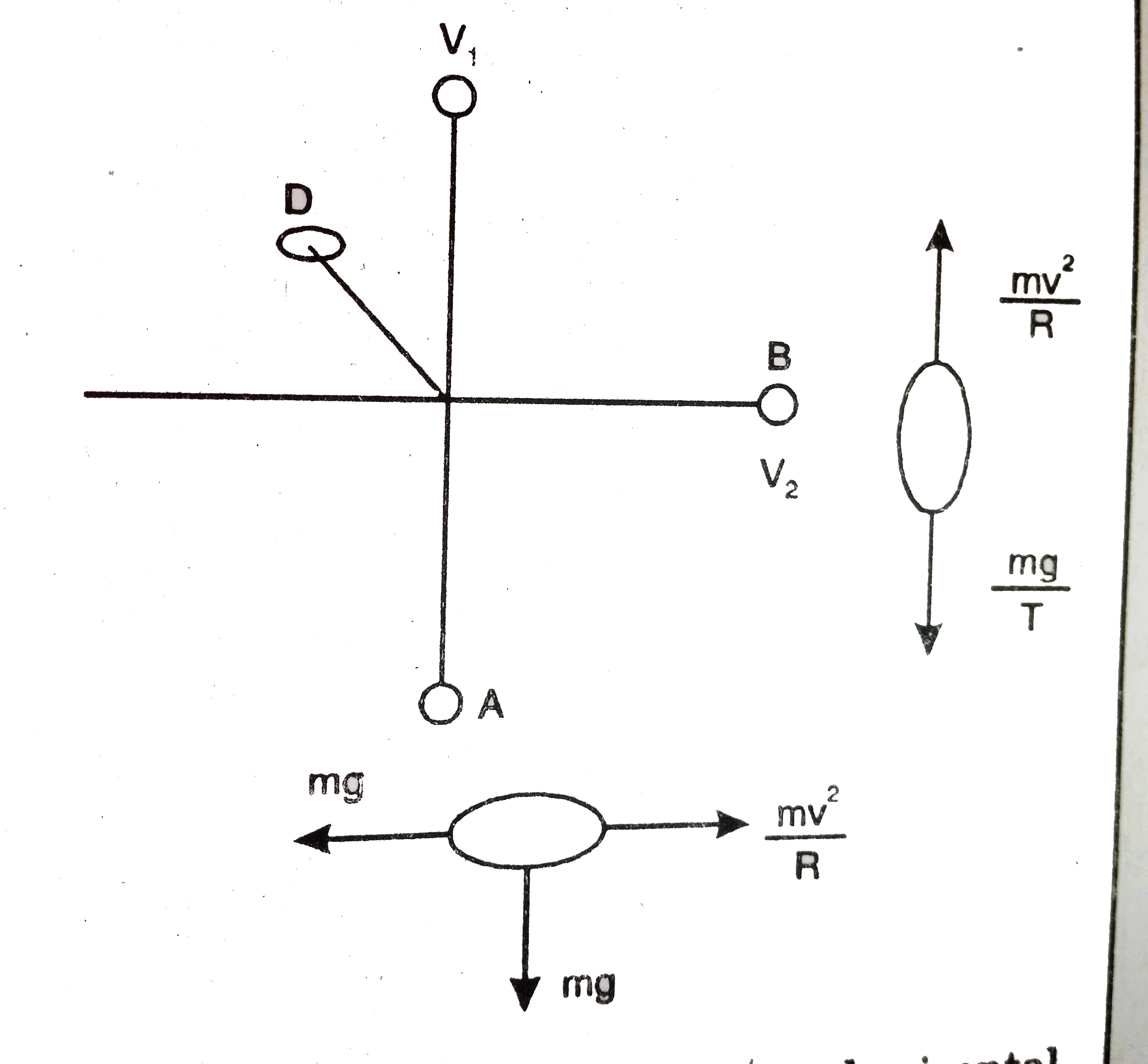
so the tension in the string at horizontal position
T=mv2R=m8gll
=8mg
b. Let the velocity at C be v3
12mv21=12mv23+mg2l
→12m10gl=12mv23+2mgl
→v23=6gl
So, the tension in the string is given by
TC=mv23l−mg=5mg
c. Let the velocity at point D be v4<br>Aga∈1/2 mv_1^2=1/2 mv_4^2+mgl(1+cos60^0)<br>rarr v_4^2=7gl<br>so,thetension∈thestr∈gis<br>T_D=(mv^2)/l-mgcos 60^0<br>=m(7gl)/l-0.5 mg<br>=7mg=0.5mg<br>6.5 mg
Topper's Solved these Questions
Similar Questions
A heavy stone is thrown from a cliff of height h with a speed v. The stone will hit the ground with maximum speed if it is thrown
07:20View SolutionA wave pulse passing on a string with speed of 40cms−1 in the negative x direction has its maximum at x=0 at t=0. Where will this maximum be located at t=5s?
02:10View SolutionThe bob of a stationary pendulum is given a sharp hit to impart it a horizontal speed of √3gl. Find the angle rotated by the string before it becomes slack.
06:15View SolutionA pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
04:43View SolutionThe bobo of a pendulum at rest is given a sharp hit to impart a horizontal velocity u where l is the length of the pendulum. Find the angle rotated by the string before it becomes slack.
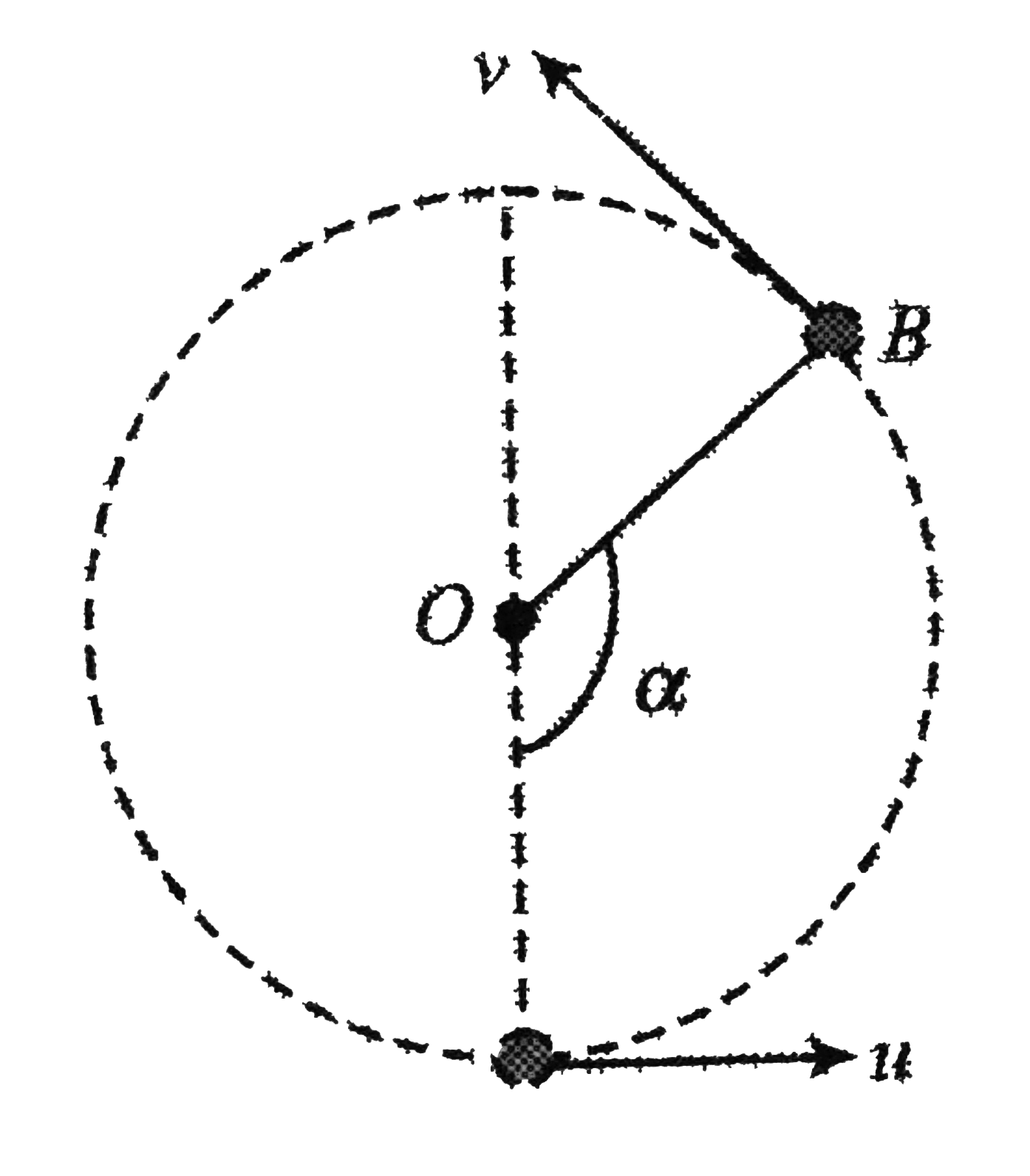 06:45View Solution
06:45View SolutionMass of the bob of a simple pendulum of length L is m . If the bob is projected horizontally from its mean position with velocity sqrt(4 gL) , then the tension in the string becomes zero after a vertical displacement of
11:13View Solutionएक दोलक की लंबाई l और इसके बॉब का द्रव्यमान m हैं । यह विरामावस्था में लटक रहा हैं । इसके बॉब को एक क्षैतिज वेग √10glदिया जाता हैं । डोरी में तनाव ज्ञात कीजिए जब बॉब उच्चतम बिंदु पर पहुँच जाए ।
05:07View SolutionEffective length of a pendulum is 50 cm and the mass of the bob is 4g . The bob is drawn to one side until the string is horizontal , and is then released. When the string makes an angle 60∘ with the vertical , what is the velocity and the kinetic energy of the bob ?
09:34View SolutionMass of the bob of a pendulum is 100g and the length of the string is 1m . The bob is initially held is such a way that the string is horizontal . The bob is then released. Find the kinetic energy of the bob when the string makes an angle of (i) 0^@ with vertical
04:34View SolutionMass of the bob of a pendulum is 100g and the length of the string is 1m . The bob is initially held is such a way that the string is horizontal . The bob is then released. Find the kinetic energy of the bob when the string makes an angle of (ii) 30^@ with the vertical .
View SolutionThe bob of a stationary pendulum is given a sharp hit to impart it a horizontal speed of √3gl. Find the angle rotated by the string before it becomes slack.
View SolutionA simple pendulum bob is given a horizontal velocity √72gl at the bottom. The string slackens after swinging through an angle θ with the vertical. The θ is
View SolutionA simple pendulum of length . l . has a bob of mass .m.. If it is released when the string makes an angle 60° with the vertical, the tension in the string at the lowest position is,
06:41View SolutionA simple pendulum bob is given a horizontal velocity √72gl at the bottom. The string slackens after swinging through an angle θ with the vertical. The θ is:
View SolutionA pendulum bob of mass .m. is held out in the horizontal position and then released from rest. If the string is of length l(I) what is the velocity of the bob and tension in the string when the bob reaches the lowest position (2) If the breaking of the string.
View SolutionA simple pendulum of length 'l' has a bob of mass 'm'. If it is released when the string makes an angle 60^(@) with the vertical, the tension in the string at the lowest position is
View Solution
