A particle of mass m is kept on a fixed, smooth sphere of radius R at a position, where the radius through the particle makes an angle of 30 ∘ with the vertical. The particle is released from this position. (a) What is the force exerted by the sphere on the particle just after the release? (b) Find the distance traveled by the particle before it leaves contact with the sphere.Solution in Tamil
The correct Answer is:B, C, D
a. When the particle is released from rest ure the centrifugal force is zero.
N force =mgcosθ=mgcos300
=√32mg
b. When the particle leaves contact with the surface ure N=θ
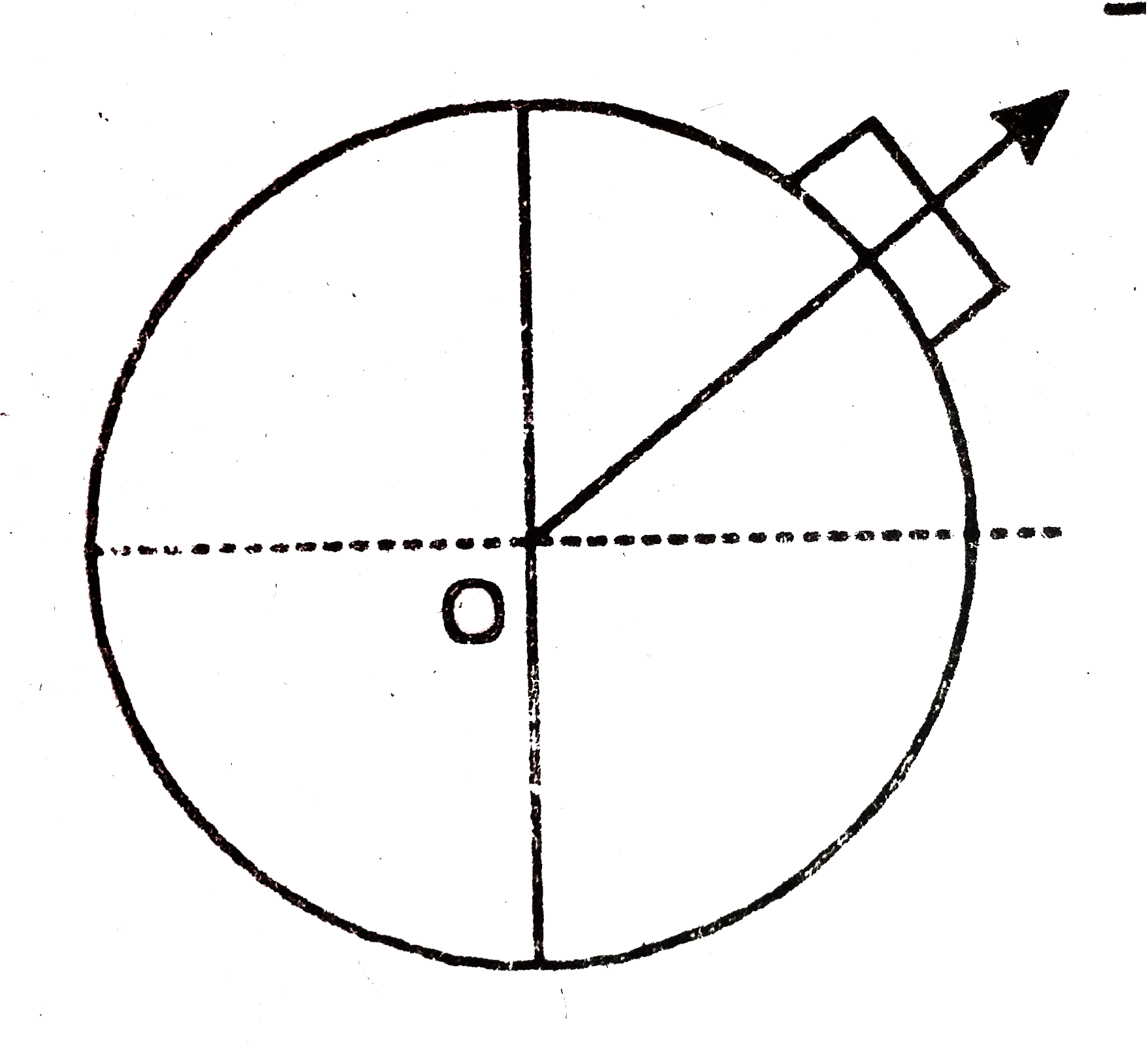
So,mv2R=mgcosθ
→v2=Rcosθ ........i
Aga∈<br>(12)mv2=mgR(cos300−cosθ)
→v2=2rg(√32−cosθ)........ii
omequationiandequationii<br>Rgcostheta=2Rg[sqrt3/2-costheta]<br>rarr 3costheta=sqrt(3)<br>rarr costheta=1/sqrt3<br>or theta=cos^-1 1/sqrt3<br>so,thedistancetravelldbythepartic≤before≤av∈gcontact<br>L=R(theta-pi/6)<br>[∵30^0=(pi/6)]<br>putting the value of theta we get<br>L=0.43R
Topper's Solved these Questions
Similar Questions
A heavy stone is thrown from a cliff of height h with a speed v. The stone will hit the ground with maximum speed if it is thrown
View SolutionA wave pulse passing on a string with speed of 40cms−1 in the negative x direction has its maximum at x=0 at t=0. Where will this maximum be located at t=5s?
View SolutionA particle slides on the surface of a fixed smooth sphere starting from the topmost pont. Find the angle rotated by the radius through the particle, when it leaves contact with the sphere.
View SolutionA particle of mass m is kept on the top of a smooth sphere of radius LR. It is given a sharp impulse whichimparts it a horizontal speed v. a. find thenormal force between the sphere and the particle just after the impulse. B. What should be the minimum value of v for which the particle does not slip on the sphere? c. Assuming the velocity v to be half the minimum calculated in part, d. find teh angle made by the radius through the particle with the vertical when it leaves the sphere.

View SolutionA particle rests on the top of a smooth hemisphere of radius r . It is imparted a horizontal velocity of sqrt(etagr) . Find the angle made by the radius vector joining the particle with the vertical at the instant the particle losses contact with the sphere.
View SolutionA particle of mass m is located outside a uniform sphere of mass M at a distance r from its centre. Find: (a) the potential energy of gravitational interaction of the particle and the sphere, (b) the gravitational force which the sphere exerts on the particle.
View SolutionA particle of mass m is released on a fixed. Smooth sphere of radius R at a position, where the radius through the particle makes an angle of alpha with the verticle. What is the angle made by radius through the particle when the particle loses contact with sphere? (cos alpha=3//4)
View SolutionA particle is released from the top of the smooth hemisphere R as shown. the normal contact between the particle and the hemisphere in position theta is
View SolutionA small box of mass m is kept on a fixed, smooth sphere of radius R at a position where the radius through the box makes an angle of 30^(@) with the vertical. The box is released from this position (a) What is the force exerted by the sphere on the box just after the release ? (b) Find the distance travelled by the box before it leaves contact with the sphere.
View SolutionA small box of mass m is placed on the outer surface of a smooth fixed sphere of radius R at a point where the radius makes a angle Pse with the vertical. The box is released from this position. Find the distance travelled by the box before it leaves contact with the sphere.
View SolutionA smooth sphere of radius R is moving in a straight line with an acceleration a. A particle is released from top of the sphere from rest. Find the speed of the particle when it is at an angular position θ from the initial position relative to the sphere.
View Solutionएक स्थिर एंव चिकने गोले के उच्चतम बिंदु से एक कण इसकी सतह पर फिसलना प्रारंभ करता हैं । जब इस कण का गोले की सतह से संपर्क छूट जाता हैं , उस समय कण से गुजरती हुई त्रिज्या का ऊपर की ओर के ऊर्ध्वाधर से बनाया गया कोण ज्ञात कीजिए ।
View SolutionR त्रिज्या के एक स्थिर एंव चिकने गोले पर m द्रव्यमान का एक कण पकड़कर रखा गया हैं । कण से गुजरनेवाली त्रिज्या ऊर्ध्वाधर 30∘ कोण बनाती हैं । कण को इस स्थिति से छोड़ दिया जाता हैं । छोड़ने के तुरंत बाद गोले व्दारा कण पर लगाया गया बल ज्ञात करें ।
View SolutionR त्रिज्या के एक स्थिर एंव चिकने गोले पर m द्रव्यमान का एक कण पकड़कर रखा गया हैं । कण से गुजरनेवाली त्रिज्या ऊर्ध्वाधर 30∘ कोण बनाती हैं । कण को इस स्थिति से छोड़ दिया जाता हैं । कण का गोले से संपर्क छूटने तक में कण व्दारा गोले पर तय की गई दूरी ज्ञात करें ।
View SolutionR त्रिज्या का एक चिकना गोला एक सरल रेखा के अनुदिश a त्वरण से बिना घूमे चल रहा हैं । गोले के उच्चतम बिंदु पर रखे हुए एक कण को गोले के सापेक्ष शून्य वेग से छोड़ दिया जाता हैं । जिस समय कण से गुजरती हुई त्रिज्या ऊर्ध्वाधर से θ कोण बनाए उस समय गोले के सापेक्ष कण की चाल निकालें ।
View SolutionA uniformly charged sphere of charge Q and radius R is placed at some height from ground surface and sphere is fixed. Now a charged particle of mass m and charge q is released form rest just below to sphere what will be speed of particle after travelling Y-direction
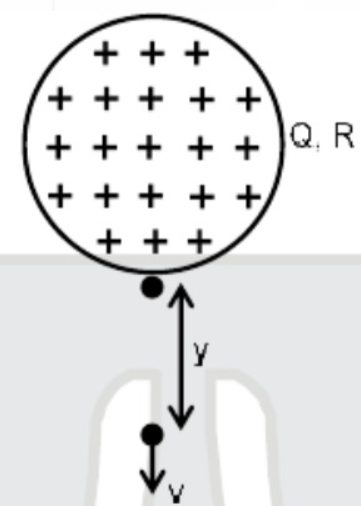
View Solution
