A horizontal platform with a small block of mass 1 kg kept on it performs vertical SHM of amplitude 1 cm. The block does not lose contact with the platform anywhere. The minimum possible time period of the platform
(a)0.1 s
(b)0.2 s
(c)0.3 s
(d)0.4 s
The correct Answer is:B
To solve the problem of finding the minimum possible time period of a platform performing vertical simple harmonic motion (SHM) with a block on it, we can follow these steps:
1. Understanding the Problem:
- We have a block of mass m=1kg on a platform that performs vertical SHM.
- The amplitude of the SHM is A=1cm=0.01m.
- The block must not lose contact with the platform at any point during the motion.
2. Condition for Maintaining Contact:
- For the block to remain in contact with the platform, the acceleration of the platform must be greater than or equal to the acceleration due to gravity g at the lowest point of the motion.
- The maximum acceleration amax in SHM is given by:
amax=ω2A
- Here, ω is the angular frequency of the SHM.
3. Setting Up the Equation:
- For the block to stay in contact, we need:
amax≥g
- Substituting the expression for maximum acceleration:
ω2A≥g
4. Solving for Angular Frequency:
- Rearranging the inequality gives:
ω2≥gA
- Taking the square root:
ω≥√gA
5. Finding the Time Period:
- The time period T of SHM is related to the angular frequency by:
T=2πω
- Substituting our expression for ω:
T≥2π√gA
6. Calculating the Minimum Time Period:
- Substituting g=10m/s2 and A=0.01m:
T≥2π√100.01=2π√1000=2π31.62≈0.199s
7. Final Result:
- The minimum possible time period T is approximately 0.199s.
- We have a block of mass m=1kg on a platform that performs vertical SHM.
- The amplitude of the SHM is A=1cm=0.01m.
- The block must not lose contact with the platform at any point during the motion.
- For the block to remain in contact with the platform, the acceleration of the platform must be greater than or equal to the acceleration due to gravity g at the lowest point of the motion.
- The maximum acceleration amax in SHM is given by:
amax=ω2A
- Here, ω is the angular frequency of the SHM.
- For the block to stay in contact, we need:
amax≥g
- Substituting the expression for maximum acceleration:
ω2A≥g
- Rearranging the inequality gives:
ω2≥gA
- Taking the square root:
ω≥√gA
- The time period T of SHM is related to the angular frequency by:
T=2πω
- Substituting our expression for ω:
T≥2π√gA
- Substituting g=10m/s2 and A=0.01m:
T≥2π√100.01=2π√1000=2π31.62≈0.199s
- The minimum possible time period T is approximately 0.199s.
Topper's Solved these Questions
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - LEVEL (2)
40 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 6-previous year question
56 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - LEVEL 0 LONG ANSWER TYPE
2 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (True/False Type)
3 Videos
- SYSTEM OF A PARTICLES & ROTATIONAL MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F
10 Videos
Similar Questions
A horizontal plank has a rectangular block placed on it. The plank starts oscillating vertically and simple harmonically with an amplitude of 40 cm. The block just loses contact with the plank when the latter is at momentary rest Then.
View SolutionA horizontal plank has a rectangular block placed on it. The plank starts oscillating vertically and simple harmonically with an amplitude of 40 cm. The block just loses contact with the plank when the latter is at momentary rest Then.
View SolutionA body of mass 8 kg performs S.H.M. of amplitude 60 cm. The restoring force is 120 N, when the displacement is 60 cm. The time period is
View SolutionA plank with a small block on top of it is under going vertical SHM. Its period is 2sec. The minium amplitude at which the block will separate from plank is :
View Solution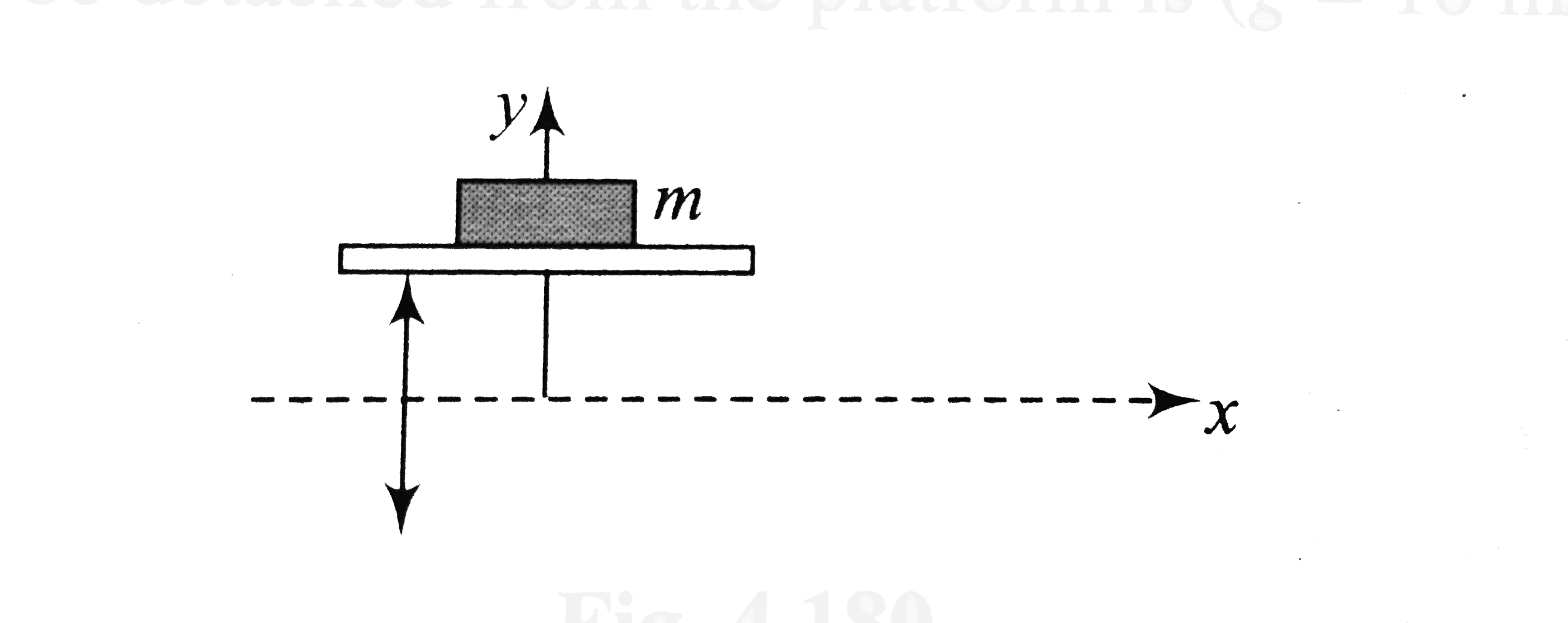
A horizontal platform with a mass m placed on it is executing SHM slong y-axis. If the amplitude of oscillation is 2.5 cm, the minimum period of the motion for the mass not to be detached from the platform (g=10ms2)
View Solution
A horizontal platform with a mass m placed on it is executing SHM slong y-axis. If the amplitude of oscillation is 2.5 cm, the minimum period of the motion for the mass not to be detached from the platform (g=10ms2)
View SolutionBlock A of mass m is performing SHM of amplitude a. Another block B of mas m is gently placed on A when it passes through mean position and B sticks to A. Find the time period and amplitude of new SHM.
View SolutionA block of mass 2 kg is kept on a rough horizontal floor an pulled with a force F. If the coefficient of friction is 0.5. then the minimum force required to move the block is :-

View SolutionA coin is placed on a horizontal platform which undergoes vertical simple harmonic motion of angular frequency ω. The amplitude of oscillation is gradually increased. The coin will leave contact with the platform for the first time
View SolutionThe system shown in the figure can move on a smooth surface. They are initially compressed by 6cm and then released, then choose the correct options.
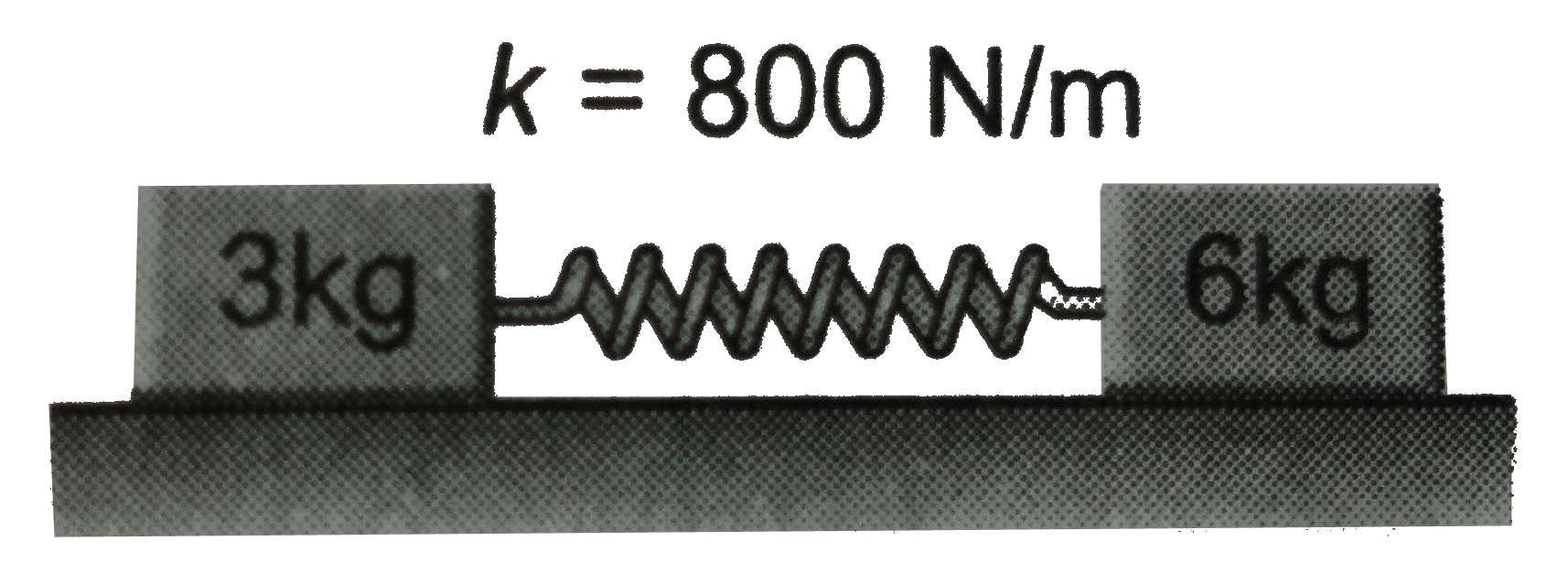
(a) The system performs, SHM with time period π10s
(b) The block of mass 3kg perform SHM with amplitude 4cm
( c) The block of mass 6kg will have maximum momentum of 2.40kg−m/s
(d) The time periods of two blocks are in the ratio of 1:√2
View Solution
