A horizontal plank has a rectangular block placed on it. The plank starts oscillating vertically and simple harmonically with an amplitude of 40 cm. The block just loses contact with the plank when the latter is at momentary rest Then.
the period of oscillation is (2π5)
the block weighs double its actual weght, then the plank is at one of the positions of momentary rest.
the block weighs 1.5 times its weight on the plank halfway down
the block weghs its true weight on the plank when the later moves fastest.
The correct Answer is:A, B, C, D
To solve the problem step by step, we will analyze the situation where a rectangular block is placed on a plank that is oscillating vertically in simple harmonic motion (SHM). The block loses contact with the plank when the plank is at its momentary rest position.
1. Understanding the System:
- The plank oscillates vertically with an amplitude A=40cm=0.4m.
- The block loses contact with the plank when the plank is at its momentary rest position.
2. Identify Forces at the Moment of Losing Contact:
- At the moment the block loses contact, the normal force N acting on the block becomes zero.
- The forces acting on the block are its weight mg (downward) and the net acceleration due to the plank's motion.
3. Acceleration of the Plank:
- The maximum acceleration amax of the plank in SHM is given by:
amax=Aω2
- Here, ω is the angular frequency of the oscillation.
4. Condition for Losing Contact:
- At the moment of losing contact, we have:
mg=mamax
- This simplifies to:
g=amax=Aω2
5. Substituting Known Values:
- We know A=0.4m and g≈10m/s2.
- Therefore:
10=0.4ω2
- Solving for ω2:
ω2=100.4=25
- Hence:
ω=5rad/s
6. Finding the Period of Oscillation:
- The period T of the oscillation is given by:
T=2πω
- Substituting ω=5:
T=2π5
7. Final Result:
- The period of oscillation is:
T=2π5s
- The plank oscillates vertically with an amplitude A=40cm=0.4m.
- The block loses contact with the plank when the plank is at its momentary rest position.
- At the moment the block loses contact, the normal force N acting on the block becomes zero.
- The forces acting on the block are its weight mg (downward) and the net acceleration due to the plank's motion.
- The maximum acceleration amax of the plank in SHM is given by:
amax=Aω2
- Here, ω is the angular frequency of the oscillation.
- At the moment of losing contact, we have:
mg=mamax
- This simplifies to:
g=amax=Aω2
- We know A=0.4m and g≈10m/s2.
- Therefore:
10=0.4ω2
- Solving for ω2:
ω2=100.4=25
- Hence:
ω=5rad/s
- The period T of the oscillation is given by:
T=2πω
- Substituting ω=5:
T=2π5
- The period of oscillation is:
T=2π5s
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Assertion Reasoning6videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Comprehension33videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single Correct107videos
LINEAR AND ANGULAR SIMPLE HARMONIC MOTION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger11videos
KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type3videos
MISCELLANEOUS KINEMATICS
Similar Questions
A horizontal platform with a small block of mass 1 kg kept on it performs vertical SHM of amplitude 1 cm. The block does not lose contact with the platform anywhere. The minimum possible time period of the platform
View SolutionPassage III A block of mass m =1/2 kg is attached with two springs each of force contant k=10N/m as shown. The block is oscillating vertically up and down with amplitude A=25cm. When the block is at its mean position one of the spring breaks without changing momentum of block.
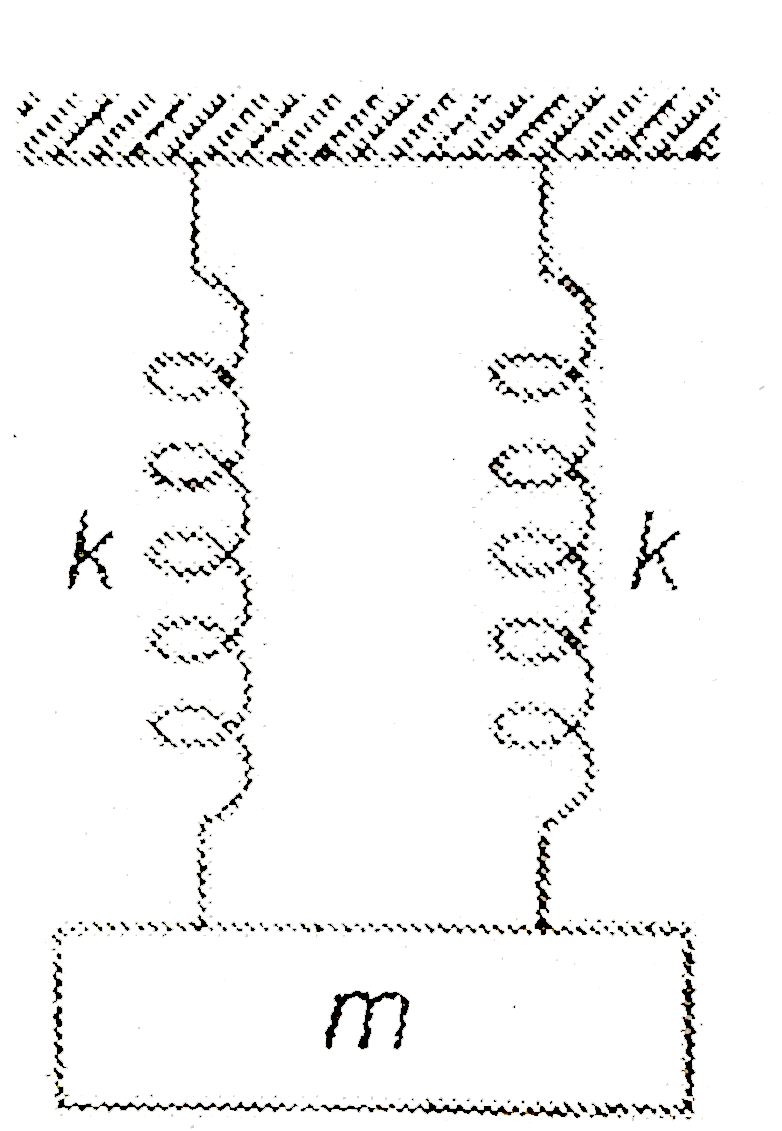
What is the new amplitude of osciallation of the block.View SolutionPassage III A block of mass m =1/2 kg is attached with two springs each of force contant k=10N/m as shown. The block is oscillating vertically up and down with amplitude A=25cm. When the block is at its mean position one of the spring breaks without changing momentum of block.
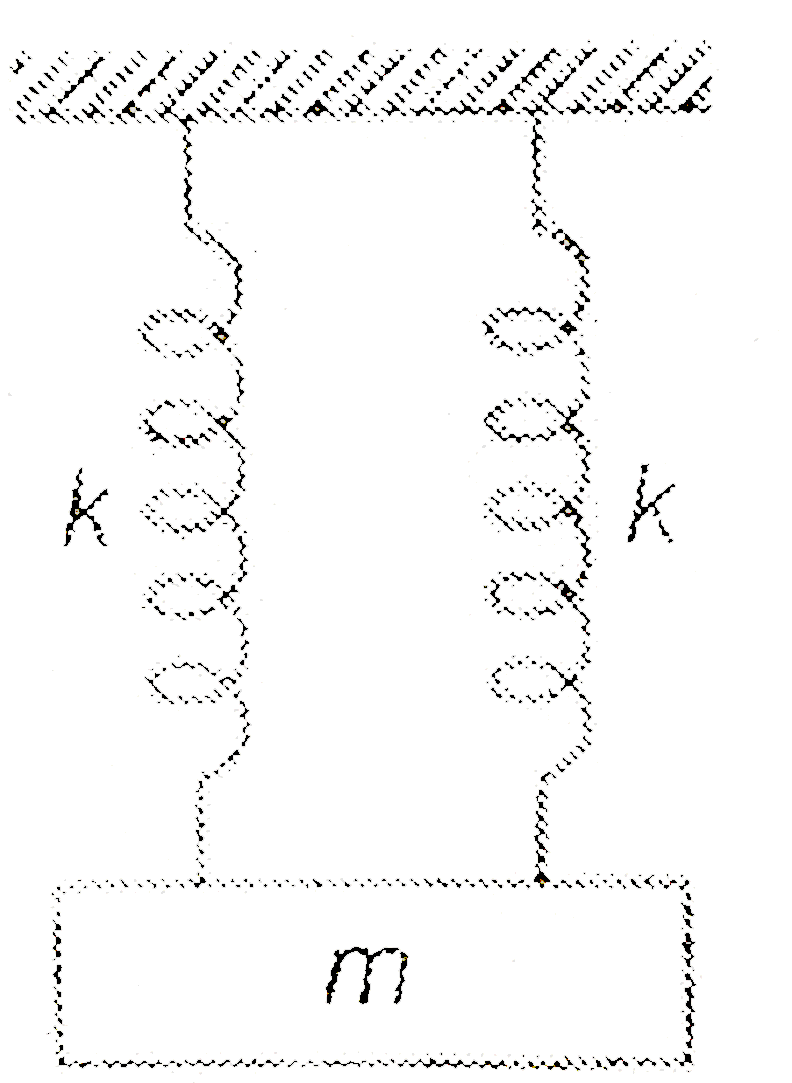
If instead of breaking one spring m/2 mass would have fallen from the block, then what will be the new amplitude?View SolutionA plank with a small block on top of it is under going vertical SHM. Its period is 2sec. The minium amplitude at which the block will separate from plank is :
View Solution
A plank of mass 12 kg is supported by two identical springs as shown is Fig. The plank always remains horizontal. When the plank is pressed down and released it performs simple harmonic motion with time period 3 s. When a block of m is attached to the plank the time priod changes to 6 s. The mass of the block isView SolutionA block is kept is kept over a horizontal platform, executing vertical SHM of angular frequency ω. Find maximum amplitude of oscillations, so that the block does not leave contact with the platform.
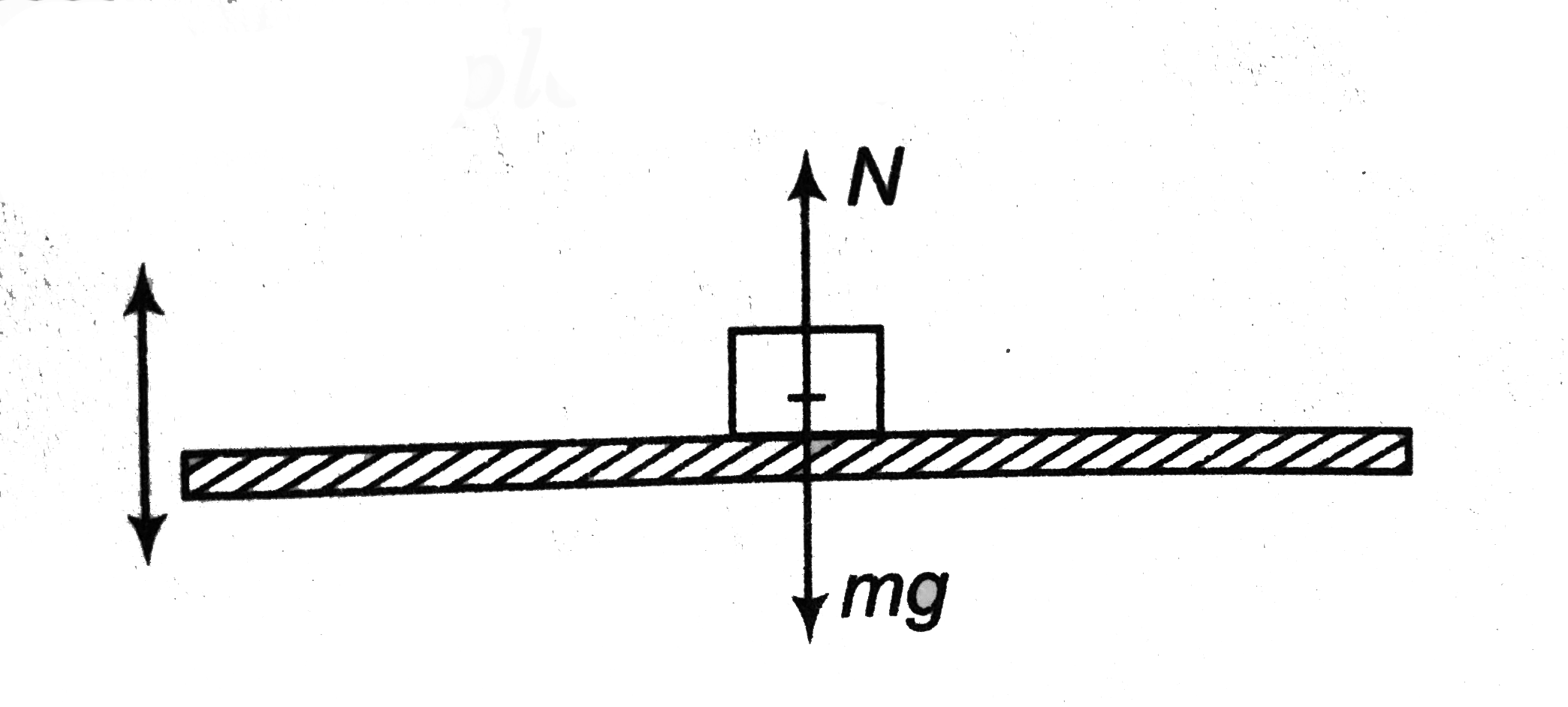 View Solution
View SolutionA block is kept on a rough horizontal plank. The coefficient of friction between the block and the plank is 12. The plank is undergoing SHM of angular frequency 10 rad/s. The maximum amplitude of plank in which the block does not slip over the plank is (g= 10 m/s2)
View SolutionA block of mass m is kept on a plank . The coefficient of friction between the plank and block is 1. The plank is slowly raised from one end so that it makes angle θ with horizontal . The forces of friction acting on the plank , when θ=30∘andθ60∘ are respectively,
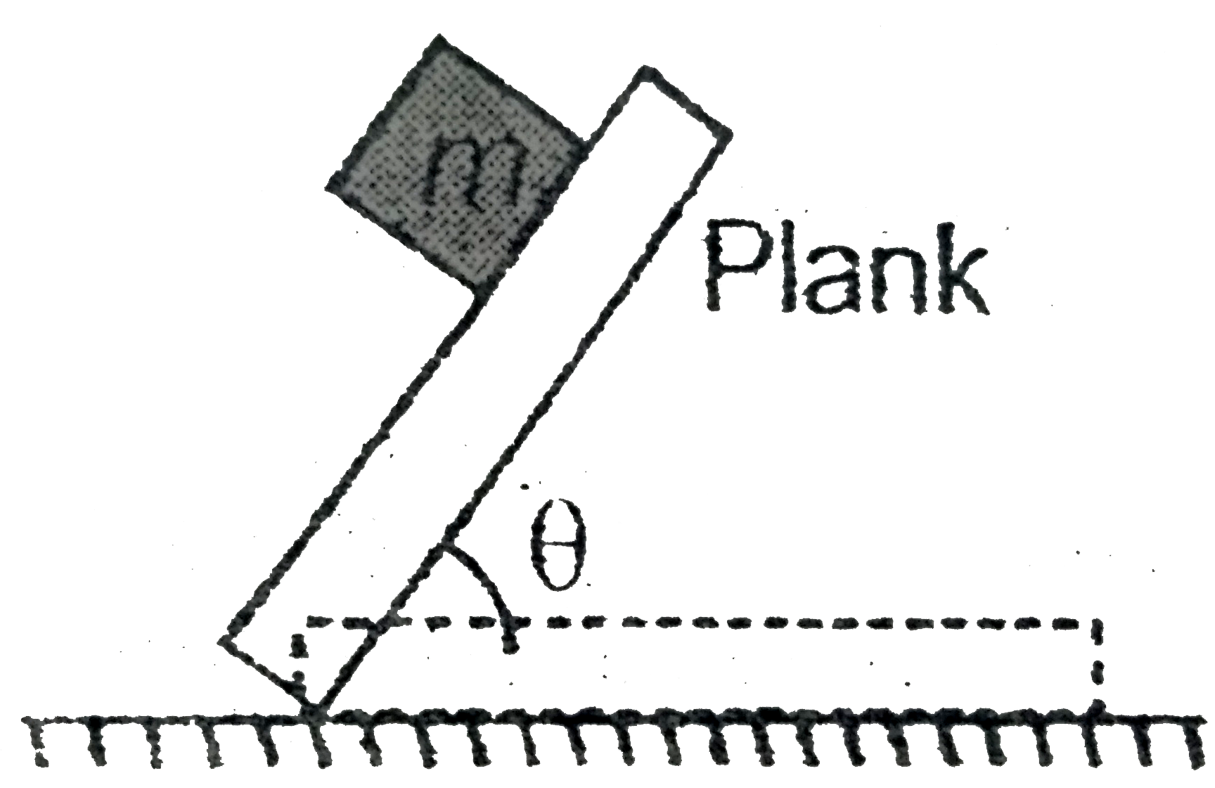 View Solution
View SolutionA horizontal plane supports a plank with a block placed on it. A light elastic string is attached to the block, which is attached to a fixed point O. Initially, the cord is unstretched and vertical. The plank is slowly shifted to right until the block starts sliding over it. It occurs at the moment when the cord deviates from vertical by an angle θ=0∘. Work done by the force F equals
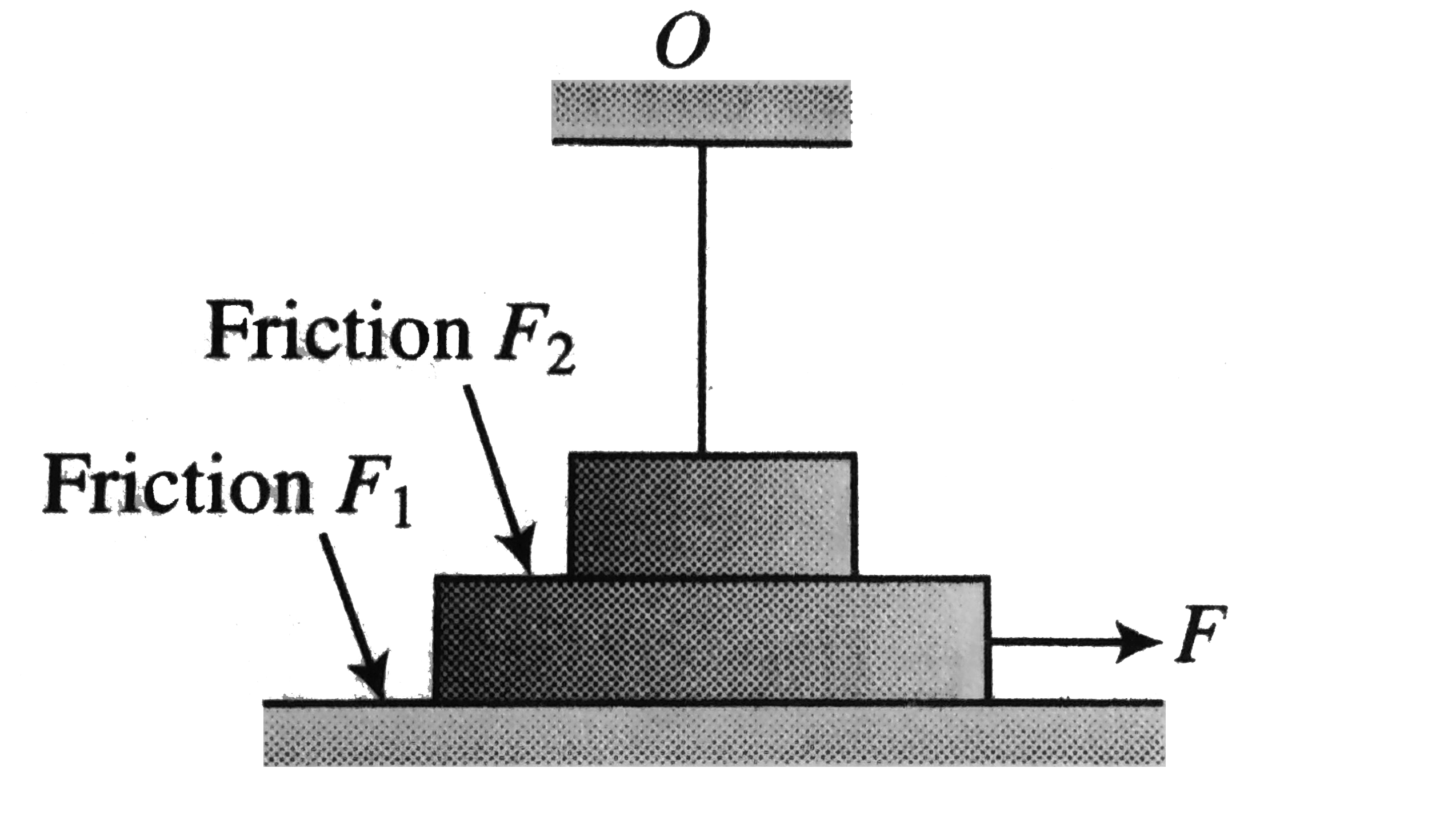 View Solution
View SolutionA horizontal plane supports a plank with a bar of mass m placed on it and attached by a light elastic non-deformed cord of length l0, to a point O. The coefficient of friciton between the bar and the plank is equal to m. The plank is slowly shifted to the right until the bar starts sliding over it. It occurs at the moment when the cord derivates from the vertical by an angle θ.
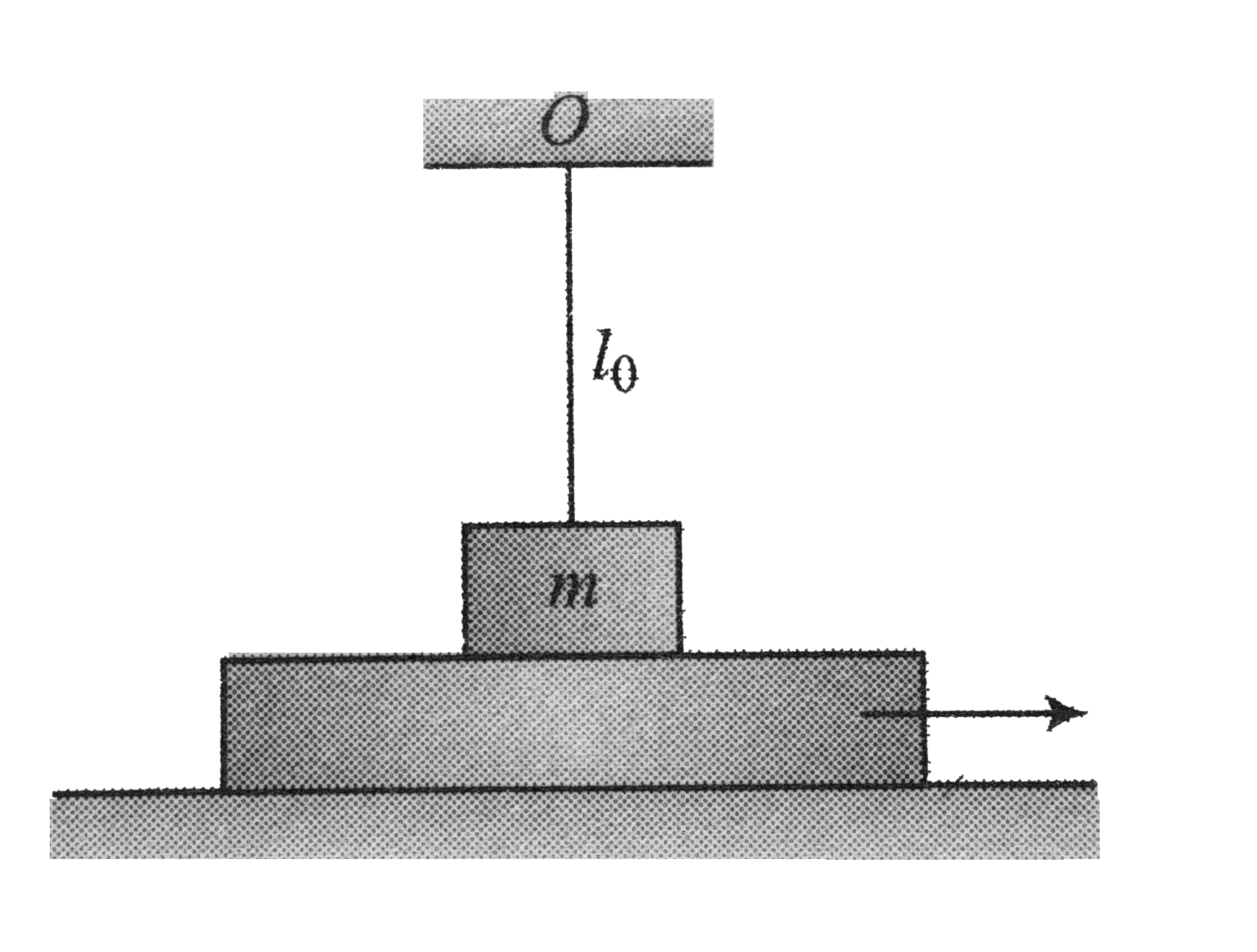
Find the work that has been performed by the moment by the frictional force acting on the bar in the reference frame fixed to the plane.View Solution
