Question

While a particle executes linear simple harmonic motion

Options

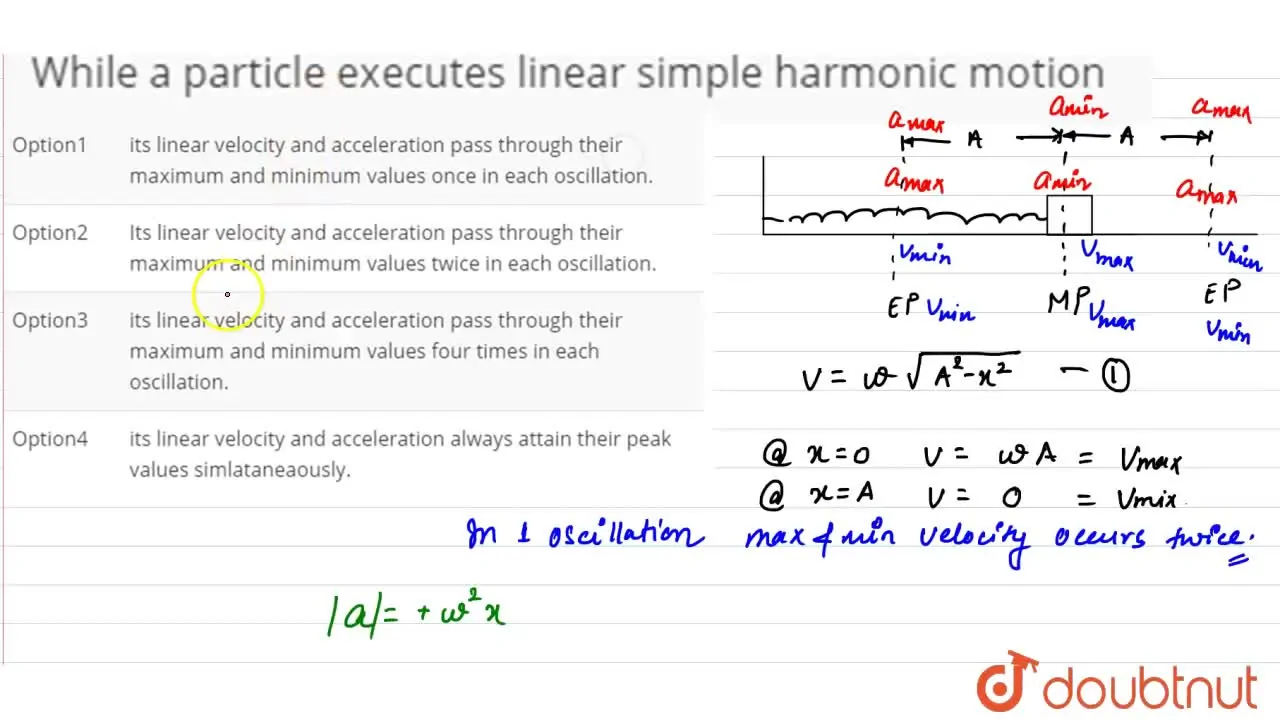
A. its linear velocity and acceleration pass through their maximum and minimum values once in each oscillation.
B. Its linear velocity and acceleration pass through their maximum and minimum values twice in each oscillation.
C. its linear velocity and acceleration pass through their maximum and minimum values four times in each oscillation.
D. its linear velocity and acceleration always attain their peak values simlataneaously.

Answer

B

Explanation

To solve the question regarding the behavior of a particle executing linear simple harmonic motion (SHM), we need to analyze the linear velocity and acceleration of the particle throughout one complete oscillation.

1. Understanding Simple Harmonic Motion (SHM):
- In SHM, a particle moves back and forth around a mean position. The maximum displacement from the mean position is called the amplitude (A).

2. Velocity in SHM:
- The velocity \( V \) of a particle in SHM can be expressed as:
\( V = \omega \sqrt{A^2 - x^2} \)
where \( \omega \) is the angular frequency and \( x \) is the displacement from the mean position.

3. Maximum and Minimum Velocity:
- At the mean position (\( x = 0 \)):
\( V_{\text{max}} = \omega A \)
- At the extreme positions (\( x = A \) or \( x = -A \)):
\( V_{\text{min}} = 0 \)
- As the particle moves from the mean position to the extreme position, it reaches maximum velocity at the mean position and minimum velocity at the extremes.

4. Counting Occurrences of Maximum and Minimum Velocity:
- Starting from the mean position, the particle reaches maximum velocity, moves to the extreme position (minimum velocity), returns to the mean position (maximum velocity again), and then goes to the opposite extreme (minimum velocity).
- Therefore, in one complete oscillation, the maximum and minimum velocities occur twice.

5. Acceleration in SHM:
- The acceleration \( a \) of a particle in SHM is given by:
\( a = -\omega^2 x \)
- The maximum acceleration occurs at the extreme positions:
\( a_{\text{max}} = \omega^2 A \)
- At the mean position (\( x = 0 \)):
\( a_{\text{min}} = 0 \)

6. Counting Occurrences of Maximum and Minimum Acceleration:
- Similar to velocity, as the particle moves:
- It reaches maximum acceleration at the extreme positions (twice: once for each extreme).
- It reaches minimum acceleration (zero) at the mean position (twice: once for each return to the mean).
- Thus, in one complete oscillation, the maximum and minimum accelerations also occur twice.

7. Conclusion:
- Both linear velocity and acceleration pass through their maximum and minimum values twice in each oscillation.

Final Answer:
The correct option is that the linear velocity and acceleration pass through their maximum and minimum values twice in each oscillation.

---