A coin is placed on a horizontal platform which undergoes vertical simple harmonic motion of angular frequency ω. The amplitude of oscillation is gradually increased. The coin will leave contact with the platform for the first time
at the mean position of platform
for an amplitude gω2
for an amplitude g2ω2
at the lowest position of the platform
The correct Answer is:B
To solve the problem of when a coin will leave contact with a platform undergoing vertical simple harmonic motion, we can follow these steps:
1. Understanding the System:
- We have a coin placed on a horizontal platform that is oscillating vertically with an angular frequency ω and an increasing amplitude A.
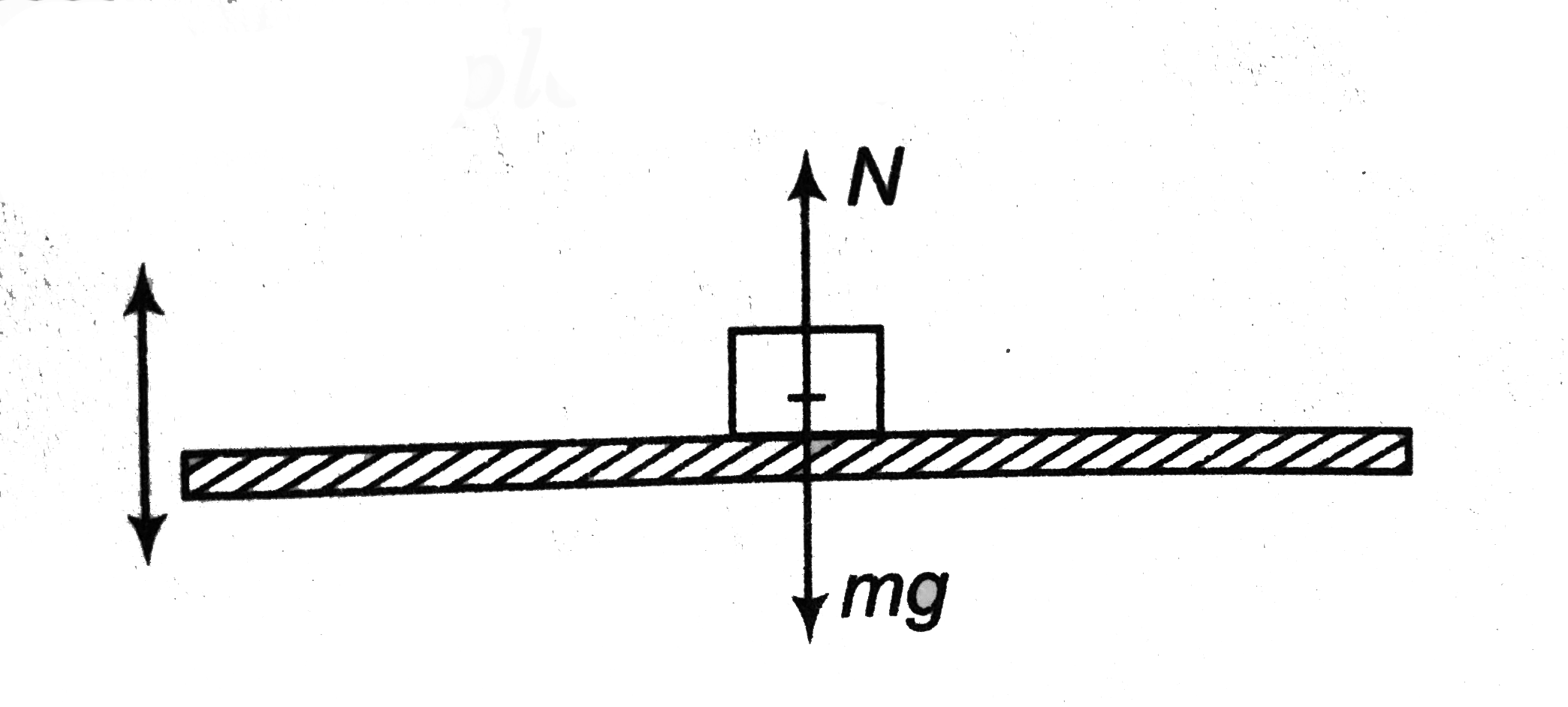
- The forces acting on the coin are its weight mg (acting downwards) and the normal force N (acting upwards).
2. Condition for Losing Contact:
- The coin will lose contact with the platform when the normal force N becomes zero. This occurs when the downward acceleration of the platform equals the acceleration due to gravity g.
3. Acceleration of the Platform:
- For a simple harmonic motion, the maximum acceleration a of the platform can be expressed as:
a=ω2A
- Here, A is the amplitude of the oscillation.
4. Setting Up the Equation:
- At the lowest point of the oscillation, the forces acting on the coin can be described by the equation:
mg−N=ma
- When the coin just loses contact, N=0, thus the equation simplifies to:
mg=ma
5. Substituting for Acceleration:
- Substituting a=ω2A into the equation gives:
mg=m(ω2A)
6. Solving for Amplitude:
- We can cancel m from both sides (assuming m≠0):
g=ω2A
- Rearranging this equation to solve for the amplitude A:
A=gω2
7. Conclusion:
- The coin will leave contact with the platform for the first time when the amplitude of oscillation reaches A=gω2.
Final Answer:
The amplitude at which the coin will leave contact with the platform for the first time is given by:
A=gω2
- We have a coin placed on a horizontal platform that is oscillating vertically with an angular frequency ω and an increasing amplitude A.
- The forces acting on the coin are its weight mg (acting downwards) and the normal force N (acting upwards).
- The coin will lose contact with the platform when the normal force N becomes zero. This occurs when the downward acceleration of the platform equals the acceleration due to gravity g.
- For a simple harmonic motion, the maximum acceleration a of the platform can be expressed as:
a=ω2A
- Here, A is the amplitude of the oscillation.
- At the lowest point of the oscillation, the forces acting on the coin can be described by the equation:
mg−N=ma
- When the coin just loses contact, N=0, thus the equation simplifies to:
mg=ma
- Substituting a=ω2A into the equation gives:
mg=m(ω2A)
- We can cancel m from both sides (assuming m≠0):
g=ω2A
- Rearranging this equation to solve for the amplitude A:
A=gω2
- The coin will leave contact with the platform for the first time when the amplitude of oscillation reaches A=gω2.
The amplitude at which the coin will leave contact with the platform for the first time is given by:
A=gω2
Topper's Solved these Questions
- BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question46videos
SIMPLE HARMONIC MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - LEVEL (2)40videos
SIMPLE HARMONIC MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (True/False Type)3videos
ROTATIONAL MOTION
- BOOK - VMC MODULES ENGLISHCHAPTER - SYSTEM OF A PARTICLES & ROTATIONAL MOTIONEXERCISE - IN-CHAPTER EXERCISE F10videos
SYSTEM OF A PARTICLES & ROTATIONAL MOTION
Similar Questions
A block is kept is kept over a horizontal platform, executing vertical SHM of angular frequency ω. Find maximum amplitude of oscillations, so that the block does not leave contact with the platform.
 View Solution
View SolutionA coin is placed on a horizontal platform, which undergoes horizontal simple harmonic motion about a mean position O. The coin does not slip on the platform. The force of friction acting on the coin is F.
(i) F is always directed towards O
(ii) F is directed towards O when the coin is moving away from O, and away from O when the coin moves towards O
(iii) F=0 when the coin and platform come to rest momentarily at the extreme position of the harmonic motion
(iv) F is maximum when the coin and platform come to rest momentarily at the extreme position of the harmonic motionView Solution
Knowledge Check
- Question 1 - Select One
A particle executing simple harmonic motion with an amplitude A and angularr frequency ω. The ratio of maximum acceleration to the maximum velocity of the particle is
AωABω2ACωDω2A
A body is executing simple harmonic motion with an angular frequency 2 rad/s . The velocity of the body at 20 mm displacement, when the amplitude of motion is 60 mm is -
View SolutionA particle executes simple harmonic motion with a frequency. (f). The frequency with which its kinetic energy oscillates is.
View SolutionA particle executing simple harmonic motion of amplitude 5cm has maximum speed of 31.4cm/s. The frequency of its oscillation is
View SolutionA horizontal platform with an object placed on it is executing SHM in the vertical direction . The amplitude of oscillation is 2.5 cm what must be the least period of these oscillations so that the object is not detached ?
View SolutionA particle executing simple harmonic motion of amplitude 5 cm has maximum speed of 31.4 cm / s . The frequency of its oscillation is
View SolutionTwo simple harmonic motions of angular frequency 100rads−1 and 1000rads−1 have the same displacement amplitude. The ratio of their maximum accelerations is
View Solution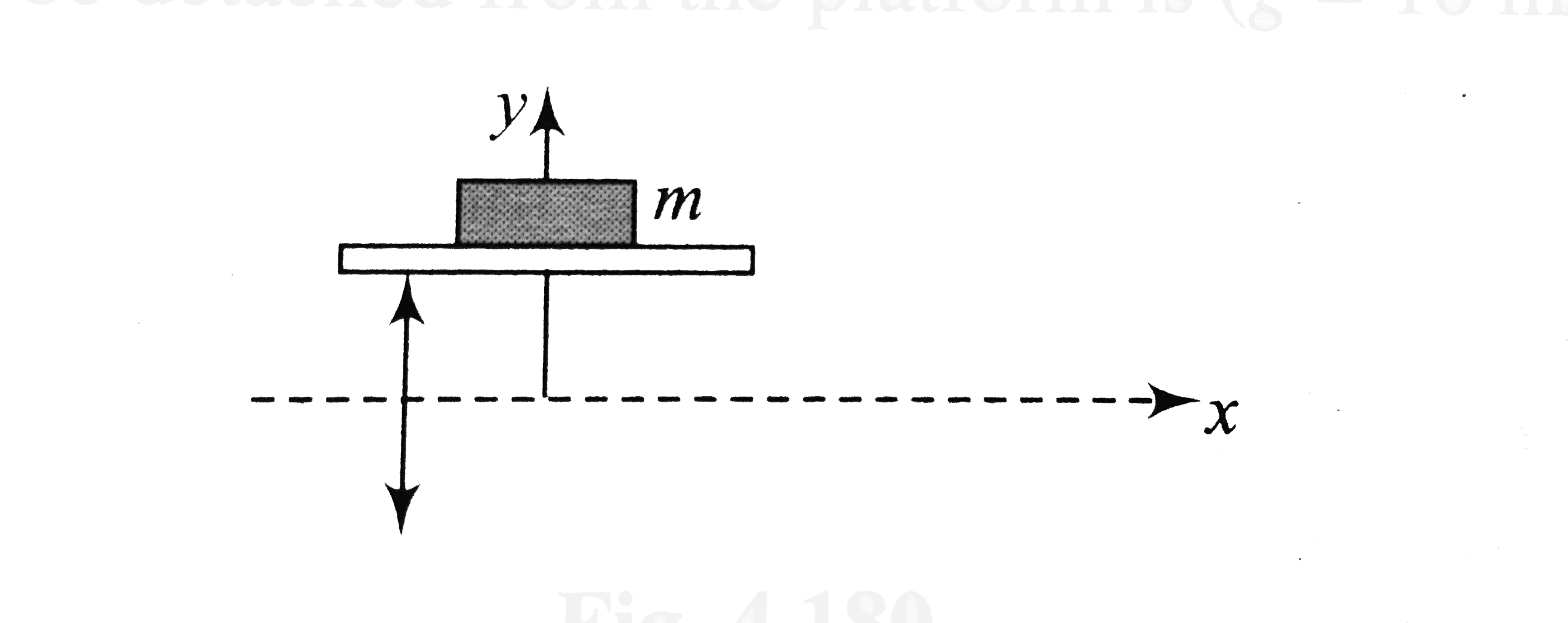
A horizontal platform with a mass m placed on it is executing SHM slong y-axis. If the amplitude of oscillation is 2.5 cm, the minimum period of the motion for the mass not to be detached from the platform (g=10ms2)View Solution
