A uniform stick of length l is mounted so as to rotate about a horizontal axis perpendicular to the stick and at a distance d from the centre of mass. The time period of small oscillation has a minimum value when d/l is
(a)1√2
(b)1√12
(c)1√3
(d)1√6
The correct Answer is:B
To solve the problem of finding the ratio dl for the minimum time period of small oscillations of a uniform stick, we will follow these steps:
Step 1: Understand the Problem
We have a uniform stick of length l that is rotating about a horizontal axis perpendicular to the stick, at a distance d from its center of mass. We need to find the ratio dl when the time period of small oscillations is minimized.
Step 2: Write the Formula for Time Period
The time period T of a physical pendulum is given by the formula:
T=2π√Imgd
where:
- I is the moment of inertia about the suspension point,
- m is the mass of the stick,
- g is the acceleration due to gravity,
- d is the distance from the suspension point to the center of mass.
Step 3: Calculate the Moment of Inertia
The moment of inertia I of a uniform stick about its center of mass is:
Icm=ml212
When the stick is rotated about a point d distance away from the center of mass, we use the parallel axis theorem:
I=Icm+md2=ml212+md2
Step 4: Substitute into the Time Period Formula
Substituting I into the time period formula, we get:
T=2π
⎷ml212+md2mgd
This simplifies to:
T=2π√l212gd+dg
Step 5: Simplify the Expression
We can factor out d from the square root:
T=2π√1g(l212d+1)
Step 6: Find the Minimum Time Period
To find the minimum time period, we need to minimize the expression inside the square root:
l212d+1
To do this, we can differentiate with respect to d and set the derivative to zero:
ddd(l212d+1)=−l212d2=0
This gives us:
l2=12d
Thus, we can express d in terms of l:
d=l212
Step 7: Calculate the Ratio dl
Now we can find the ratio:
dl=l212l=l12
Step 8: Final Result
To express this in terms of l:
dl=112
Conclusion
The minimum value of dl when the time period of small oscillation is minimized is:
dl=1√12
We have a uniform stick of length l that is rotating about a horizontal axis perpendicular to the stick, at a distance d from its center of mass. We need to find the ratio dl when the time period of small oscillations is minimized.
The time period T of a physical pendulum is given by the formula:
T=2π√Imgd
where:
- I is the moment of inertia about the suspension point,
- m is the mass of the stick,
- g is the acceleration due to gravity,
- d is the distance from the suspension point to the center of mass.
The moment of inertia I of a uniform stick about its center of mass is:
Icm=ml212
When the stick is rotated about a point d distance away from the center of mass, we use the parallel axis theorem:
I=Icm+md2=ml212+md2
Substituting I into the time period formula, we get:
T=2π
⎷ml212+md2mgd
This simplifies to:
T=2π√l212gd+dg
We can factor out d from the square root:
T=2π√1g(l212d+1)
To find the minimum time period, we need to minimize the expression inside the square root:
l212d+1
To do this, we can differentiate with respect to d and set the derivative to zero:
ddd(l212d+1)=−l212d2=0
This gives us:
l2=12d
Thus, we can express d in terms of l:
d=l212
Now we can find the ratio:
dl=l212l=l12
To express this in terms of l:
dl=112
The minimum value of dl when the time period of small oscillation is minimized is:
dl=1√12
Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 More Than One Correct
8 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 2 Comprehension
2 Videos
- SIMPLE HARMONIC MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Level 1 Subjective
39 Videos
- SEMICONDUCTORS AND ELECTRONIC DEVICESBOOK - DC PANDEY ENGLISHCHAPTER - SEMICONDUCTORS AND ELECTRONIC DEVICESEXERCISE - More than One Option is Correct
3 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
Similar Questions
A uniform stick of length l is hinged so as to rotated about a harmonic axis perpendicular to the stick, at a distance from the center . Find the value of x, for which the time period is minimum.
View SolutionThe radius of gyration of a uniform rod of mass m and length l about an axis perpendicular to its length and at distance l4 from its centre will be
View SolutionA uniform rod of mass m, length l rests on a smooth horizontal surface. Rod is given a sharp horizontal impulse p perpendicular to the rod at a distance l/4 from the centre. The angular velocity of the rod will be
View SolutionTwo uniform solid of masses m1 and m2 and radii r1 and r2 respectively, are connected at the ends of a uniform rod of length l and mass m. Find the moment of inertia of the system about an axis perpendicular to the rod and passing through a point at a distance of a from the centre of mass of the rod as shown in figure.

View Solution
A uniform rod of length 4l and mass m is free to rotate about a horizontal axis passing through a point distant l from its one end. When the rod is horizontal its angular velocity is ω as shown in figure. calculate
(a). reaction of axis at this instant,
(b). Acceleration of centre of mass of the rod at this instant.
(c). reaction of axis and acceleration of centre mass of the rod when rod becomes vertical for the first time.
(d). minimum value of ω, so that centre of rod can complete circular motion.
View SolutionAn uniform disc is rotating at a constant speed in a vertical plane about a fixed horizontal axis passing through the centre of the disc. A piece of the disc from its rim detaches itself from the disc at the instant when it is at horizontal level with the centre of the disc and moving upwards, then about the fixed axis.
STATEMENT-1 : Angular speed of the disc about the aixs of rotation will increase.
and
STATEMENT-2 : Moment of inertia of the disc is decreased about the axis of rotation.
View SolutionA uniform stick of length l and mass m lies on a smooth table. It rotates with angular velocity ω about an axis perpendicular to the table and through one end of the stick. The angular momentum of the stick about the end is
View SolutionA uniform rod of mass m and length l is suspended about its end. Time period of small angular oscillations is

View SolutionA uniform rod ofmass M and length L is hanging from its one end free to rotate in a veritcal plane.A small ball of equal mass is attached of the lowe end as shown. Time period of small oscillations of the rod is

View Solution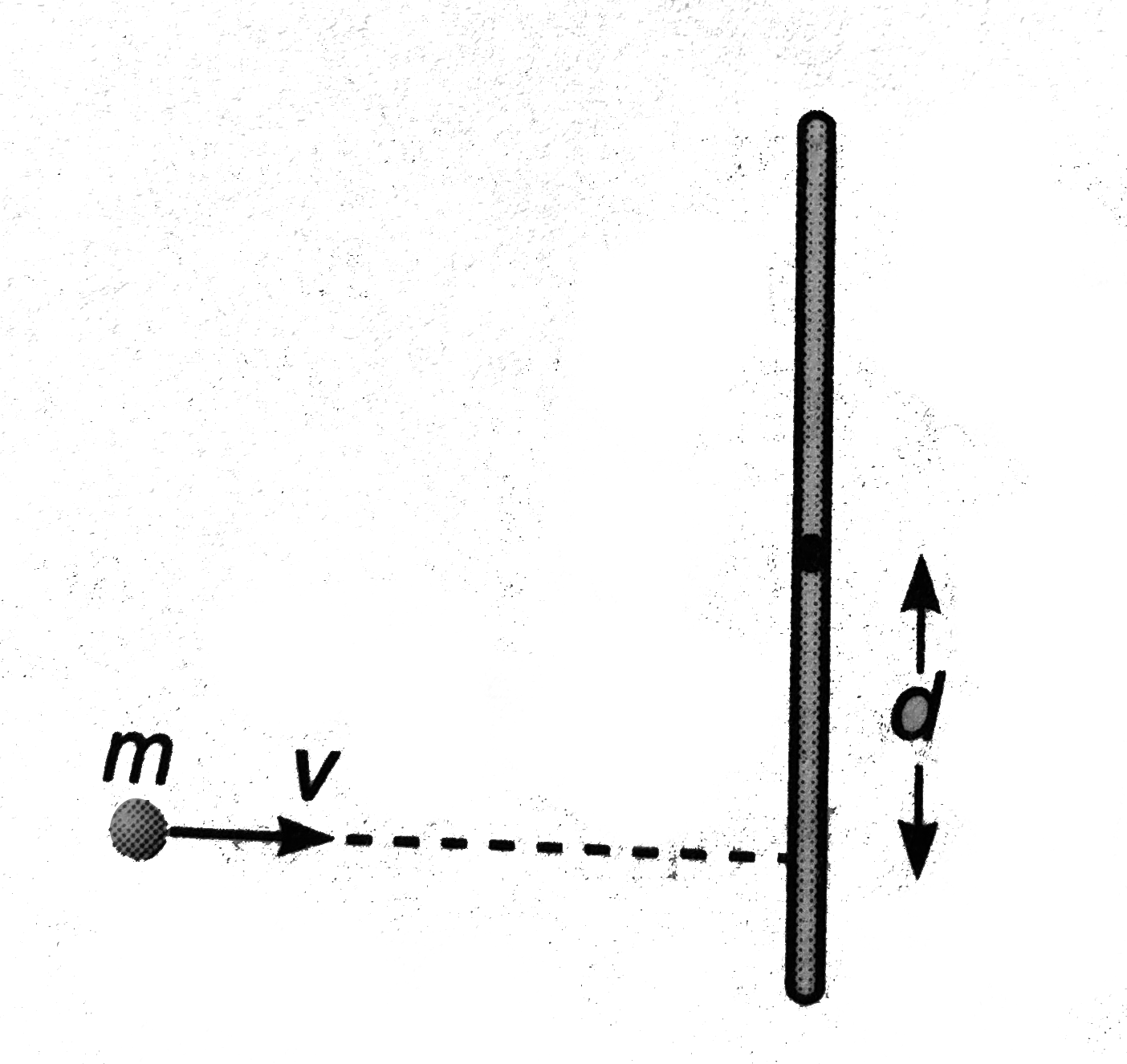
A stick of length l lies on horizontal table. It has a mass M and is free to move in any way on the table. A bal of mass m moving perpendicularly to the stick at a distance d from its centre with speed v collides elastically with it as shown in figure. what quantities are conserved in the collision ? what must be the mass of the ball, so that it remains at rest immediately after collision?
View Solution
