Question

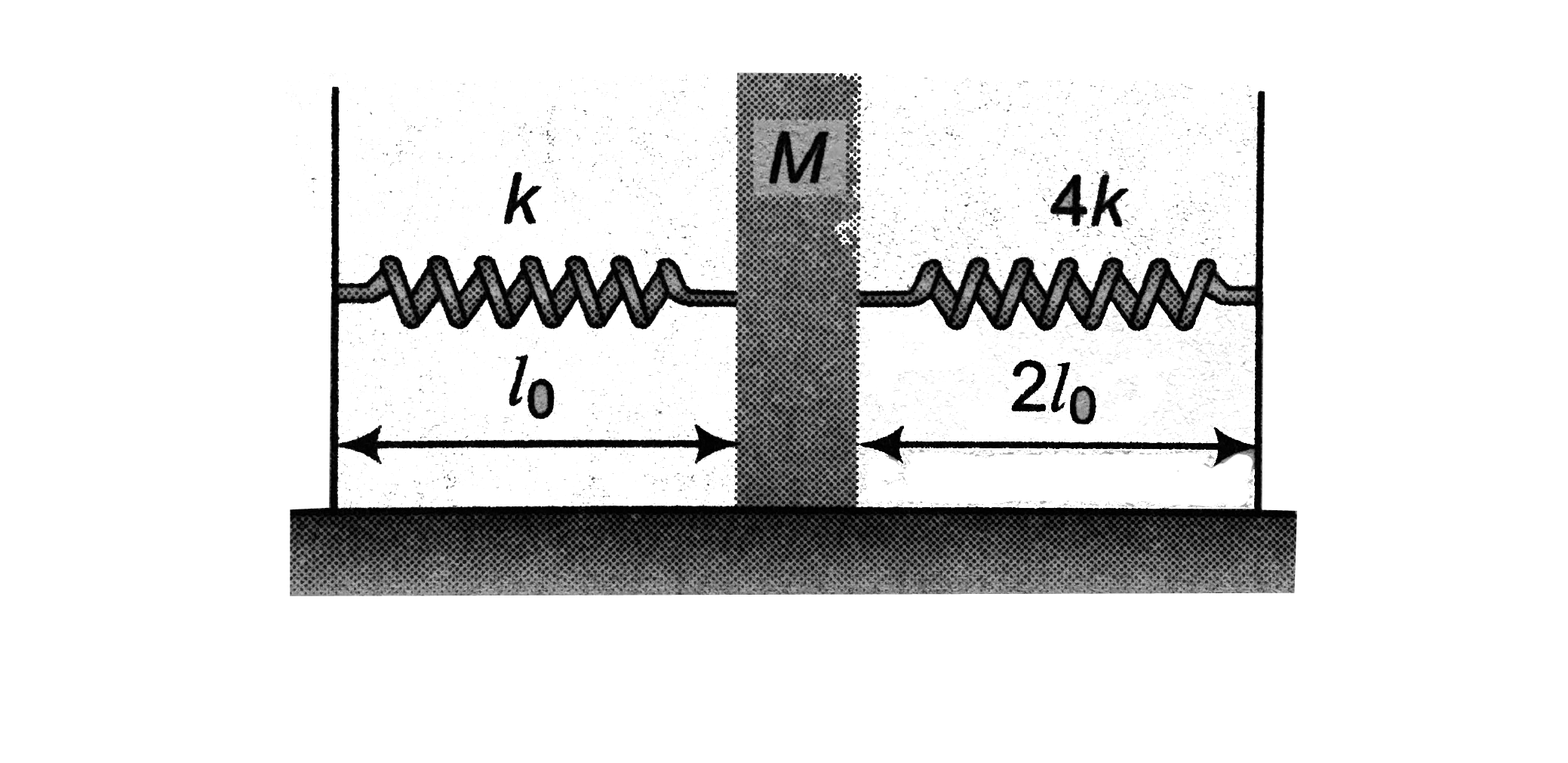
A block of mass $M$ is kept on a smooth surface and touches the two springs as shown in the figure but not attached to the springs. Initially springs are in their natural length. Now, the block is shifted $(l_0/2)$ from the given position in such a way that it compresses a spring and released. The time - period of oscillation of mass will be

Options

A. `(pi)/(2)sqrt((M)/(k))`
B. `2pi sqrt ((M)/(5k))`
C. `(3pi)/(2) sqrt ((M)/(k))`
D. `pi sqrt ((M)/(2k))`

Answer

C

Explanation

Half the oscillation is completed with one spring and the other half with other spring. Hence,
`T = (T_(1))/(2) + (T_(2))/(2) = (2pi sqrt(((M))/(k)))/(2) + (2pi sqrt(((M))/(4k)))/(2)`
`= pi sqrt ((M)/(k))[1 + 1/2] = (3pi)/(2) sqrt((M)/(k))`