A ball is projected from a given point with velocity u at some angle with the horizontal and after hitting a vertical wall returns to the same point. Show that the distance of the point from the wall must be less than eu2(1+e)g, where e is the coefficient of restitution.
To solve the problem, we will follow a systematic approach to derive the required expression for the distance from the wall.
1. Understanding the Problem:
A ball is projected with an initial velocity u at an angle θ with the horizontal. After hitting a vertical wall, it returns to the same point. We need to show that the distance D from the point of projection to the wall must be less than eu2(1+e)g, where e is the coefficient of restitution.
2. Break Down the Initial Velocity:
The initial velocity can be resolved into horizontal and vertical components:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
3. Time of Flight Until Collision:
The time T taken for the ball to reach the wall can be calculated using the horizontal motion:
T=Ducosθ
4. Total Time of Flight:
The total time of flight for the projectile motion (up and down) until it returns to the same height is given by:
Ttotal=2usinθg
5. Time After Collision:
Let T1 be the time taken to reach the wall, then the time taken after the collision to return to the same point is:
TR=Ttotal−T1=2usinθg−Ducosθ
6. Velocity After Collision:
After the collision, the horizontal component of the velocity becomes eucosθ (due to the coefficient of restitution).
7. Distance After Collision:
The distance traveled after the collision back to the original point can be expressed as:
D=eucosθ⋅TR
8. Substituting for TR:
Substitute TR into the distance equation:
D=eucosθ(2usinθg−Ducosθ)
9. Rearranging the Equation:
Rearranging gives:
D+eDucosθ=2eu2sinθcosθg
D(1+eucosθ)=2eu2sinθcosθg
10. Solving for D:
Solving for D:
D=2eu2sinθcosθg(1+eucosθ)
Using the identity sin2θ=2sinθcosθ:
D=eu2sin2θg(1+e)
11. Maximizing D:
The maximum value of D occurs when sin2θ=1:
Dmax=eu2g(1+e)
12. Conclusion:
Therefore, the distance D must be less than eu2g(1+e).
A ball is projected with an initial velocity u at an angle θ with the horizontal. After hitting a vertical wall, it returns to the same point. We need to show that the distance D from the point of projection to the wall must be less than eu2(1+e)g, where e is the coefficient of restitution.
The initial velocity can be resolved into horizontal and vertical components:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
The time T taken for the ball to reach the wall can be calculated using the horizontal motion:
T=Ducosθ
The total time of flight for the projectile motion (up and down) until it returns to the same height is given by:
Ttotal=2usinθg
Let T1 be the time taken to reach the wall, then the time taken after the collision to return to the same point is:
TR=Ttotal−T1=2usinθg−Ducosθ
After the collision, the horizontal component of the velocity becomes eucosθ (due to the coefficient of restitution).
The distance traveled after the collision back to the original point can be expressed as:
D=eucosθ⋅TR
Substitute TR into the distance equation:
D=eucosθ(2usinθg−Ducosθ)
Rearranging gives:
D+eDucosθ=2eu2sinθcosθg
D(1+eucosθ)=2eu2sinθcosθg
Solving for D:
D=2eu2sinθcosθg(1+eucosθ)
Using the identity sin2θ=2sinθcosθ:
D=eu2sin2θg(1+e)
The maximum value of D occurs when sin2θ=1:
Dmax=eu2g(1+e)
Therefore, the distance D must be less than eu2g(1+e).
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONExercise:Level 2 Comprehension Based Questions
Explore 3 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEY ENGLISHChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
- CIRCULAR MOTIONBook:DC PANDEY ENGLISHChapter:CIRCULAR MOTIONExercise:Medical entrances s gallery
Explore 19 Videos
Similar Questions
A ball is projected from ground with a velocity V at an angle θ to the vertical. On its path it makes an elastic collison with a vertical wall and returns to ground. The total time of flight of the ball is
View SolutionA ball is projected from a point in a horizontal plane with a velocity of 20 m/s at 30∘ with the horizontal. Coefficient of restitution is 0.8, then
View SolutionA ball is projected from a point A with some velocity at an angle 30∘ with the horizontal as shown in (Fig. 5.204). Consider a target at point B. The ball will hit the target if it thrown with a velocity v0 equal to.
 .
.
View SolutionA projectile, thrown with velocity v0 at an angle α to the horizontal, has a range R. it will strike a vertical wall at a distance R/2 from the point of projection with a speed of
View SolutionA ball is projected at t=0 with velocity v0 at angle θ with horizontal. It strikes a smooth wall at a distance from it and then falls at a distance d from the wall. Coefficient of restitution is 'e' then :
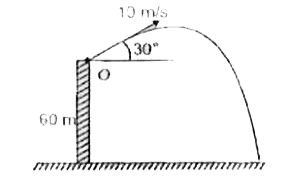
View SolutionA ball is projected from a point O as shown in figure it will strike the ground after (g = 10 m/s2 )
View SolutionA ball is thrown from a point in level with velocity u and at a horizontal distance r from the top os a tower of height h.
At what horizontal distance x from the foot of the tower does the ball hit the ground ?
View SolutionA stone is projected from the ground with a velocity of 20 m/s at angle 30∘ with the horizontal. After one second it clears a wall then find height of the wall. (g=10ms−2)
View SolutionA ball is projected from a point on the floor wilth a speed of 15 m/s at an angle of 600 with the horizontal. Will ilt hit a vertical wall 5 m away from the point of projection and perpendiculaer to the plane of projection without and perpendicular to the plane of projection without hitting the floor? will the answer differ if the wall is 22 m away?
View SolutionA ball is projected from the ground at angle θ with the horizontal. After 1s, it is moving at angle 45∘ with the horizontal and after 2s it is moving horizontally. What is the velocity of projection of the ball ?
View Solution