Two blocks of masses 2kg and M are at rest on an inclined plane and are separated by a distance of 6.0m as shown. The coefficient of friction between each block and the inclined plane is 0.25. The 2kg block is given a velocity of 10.0m/s up the inclined plane. It collides with M, comes back and has a velocity of 1.0m/s when it reaches its initial position. The other block M after the collision moves 0.5m up and comes to rest. Calculate the coefficient of restitution between the blocks and the mass of the block M.
[Take sinθ=tanθ=0.05 and g=10m/s2]
![Two blocks of masses 2kg and M are at rest on an inclined plane and are separated by a distance of 6.0m as shown. The coefficient of friction between each block and the inclined plane is 0.25. The 2kg block is given a velocity of 10.0m//s up the inclined plane. It collides with M, comes back and has a velocity of 1.0m//s when it reaches its initial position. The other block M after the collision moves 0.5m up and comes to rest. Calculate the coefficient of restitution between the blocks and the mass of the block M. [Take sintheta=tantheta=0.05 and g=10m//s^2]](https://d10lpgp6xz60nq.cloudfront.net/physics_images/DCP_V02_CH11_E01_193_Q01.png)
The correct Answer is:A, B, D
Let v1= velocity of block 2kg just before collision
v2= velocity of block 2kg just after collision
and v3= velocity of block M just after collision.
Applying work energy theorem
(change in kinetic energy=work done by all the forces) at different stages as shown in figure.

[12m{v21−(10)2}]=−6μmgcosθ−mgh1
(m=2kg)
or v21−100=2[6μgcosθ+gh1]
cosθ=√1−sin2θ=√1−(0.05)2=0.99
∴v21=100−2[(6)(0.25)(10)(0.99)+(10)(0.3)]
⇒v1=8m/s

ΔKE=Wfriction+Wgravity
12m[(1)2−(v22)]=−6μmgcosθ+mgh1
or 1−v21=2[−6μcosθ+gh1]
=2[(−6)(0.25)(10)(0.99)+(10)(0.3)]
=−23.7
∴v22=24.7 or v2=5m/s

ΔKE=Wfriction+Wgravity
12M[0−v23]=−(0.5)(μ)(M)gcosθ−Mgh2
or −v23=−μcosθ−2gh2
or v23=(0.25)(10)(0.99)+2(10)(0.025)
or v23=2.975
∴v3≈1.72m/s
Now,
(i) Coefficient of restitution
=Relative velocity of separationRelative velocity of approach
=v2+v3v1=5+1.728=6.728
or e≈0.84
(ii) Applying conservation of linear momentum before and after collision
2v1=Mv3−2v2
∴M=2(v1+v2)v3=2(8+5)1.72=261.72
M=15.12kg
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Comprehension Based Questions3videos
CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION
- BOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions15videos
CENTRE OF MASS, IMPULSE AND MOMENTUM
- BOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery19videos
CIRCULAR MOTION
Similar Questions
A block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View SolutionA block of mass m is placed at rest on an inclination θ to the horizontal. If the coefficient of friction between the block and the plane is μ, then the total force the inclined plane exerts on the block is
View Solution
Knowledge Check
- Question 1 - Select One
A block of mass 3 kg rests on a rough inclined plane making an angle of 30∘. With the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A9.8 NB0.7×9.8×√3 NC9.8×√3 ND0.7×9.8 N - Question 2 - Select One
A block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A10.3NB23.8NC11.9ND6.3N - Question 3 - Select One
Two blocks each of mass M are resting on a frictionless inclined plane as shown in fig then:
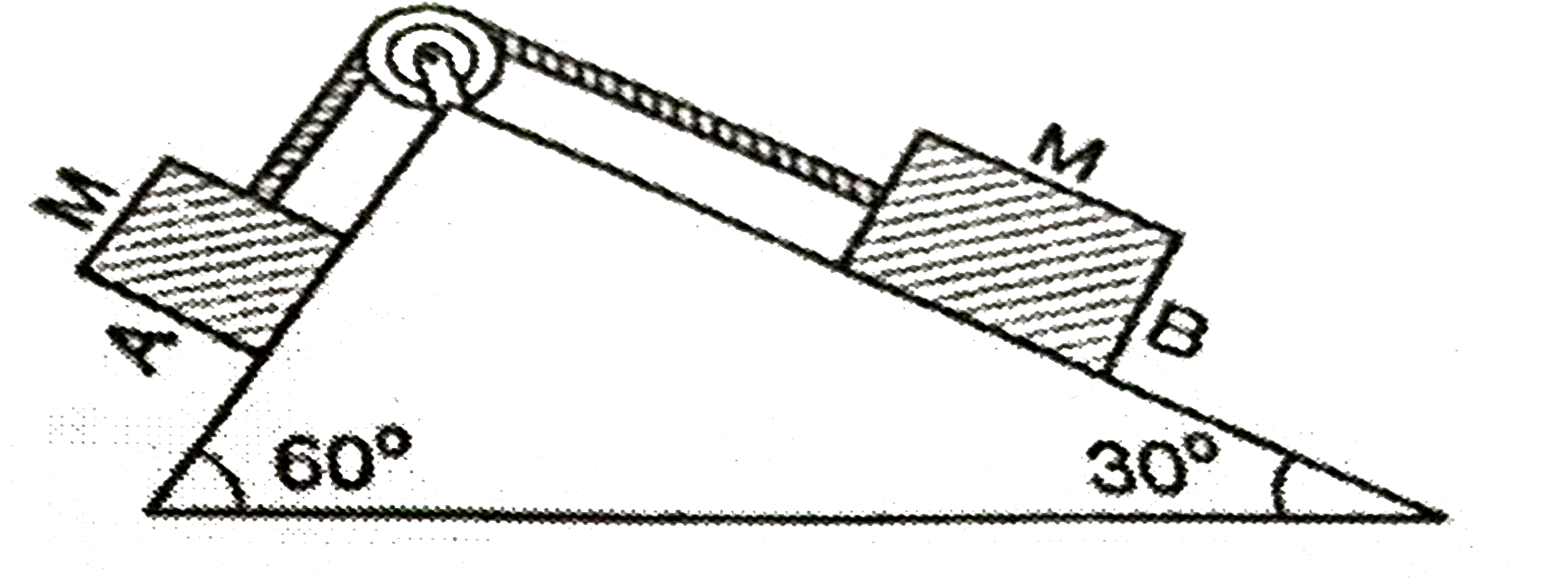 AThe block A moves down the planeBThe block B moves down the plane.CBoth the blocks remain at restDBoth the blocks move down the plane.
AThe block A moves down the planeBThe block B moves down the plane.CBoth the blocks remain at restDBoth the blocks move down the plane.
Two identical blocks A and B of equal masses are placed on rough inclined plane as shown in the figure. Initially the block A is √2m behind the block B. Coefficient of kinetic friction for the blocks A and B are 0.2 and 0.3 respectively (g=10m/s2). By the time the two blocks come on the same line on the inclined plane if they are released simultaneously, the distance moved by B is βm Find the value of β
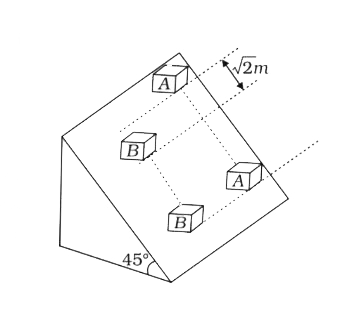 View Solution
View SolutionFind the force required to pull a block of mass 100kg up an incline of 8∘ at a uniform speed of 5m/s. The coefficient of friction between the block and the plane is 0.3.
View SolutionA block of mass m slides down an inclined plane of inclination θ with uniform speed. The coefficient of friction between the block and the plane is μ. The contact force between the block and the plane is
View SolutionA block of mass m is at rest on a rough inclined plane of angle of inclination θ. If coefficient of friction between the block and the inclined plane is μ, then the minimum value of force along the plane required to move the block on the plane is
View SolutionA block of mass 2 kg slides down an incline plane of inclination 30°. The coefficient of friction between block and plane is 0.5. The contact force between block and plank is :
View Solution
