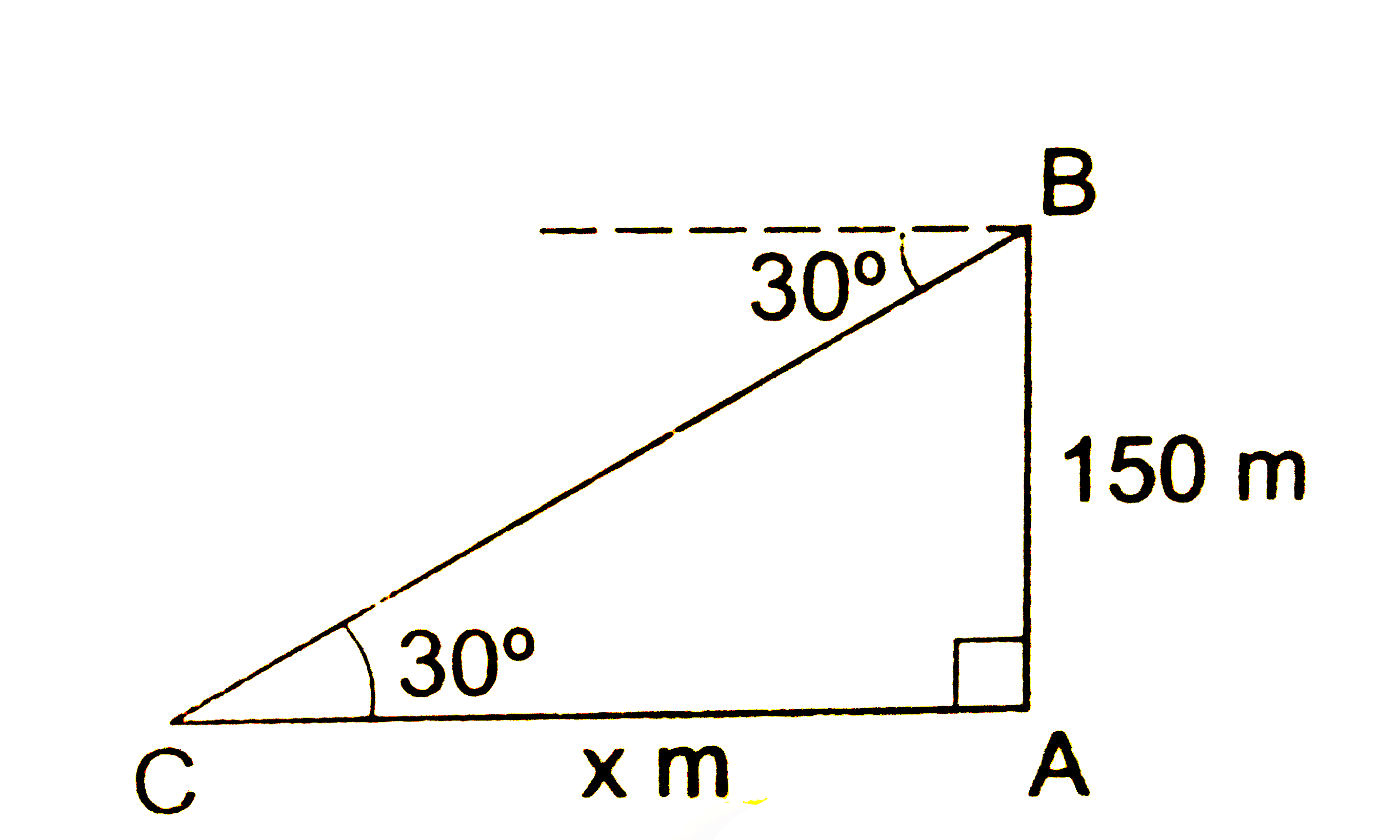
The angle of depression of a car parked on the road from the top of the 150 m high tower is $30^{\circ}$ . Find the distance of the car from the tower.

A

$50 \sqrt{3} m$

B

$150 \sqrt{3} m$

C

$150 \sqrt{2} m$

D

$75 m$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Let AB be the tower and C be the position of the car on the ground such that $∠ A C B = 30^{\circ}$ ,
Let AC = x metres. Then , $\frac{A C}{A B} = {cot 30}^{\circ} \Rightarrow \frac{x}{150} = \sqrt{3}$ .
$\Rightarrow x = 150 \sqrt{3}$ .

|

Updated on:21/07/2023

Class 10 MATHS SOME APPLICATIONS OF TRIGONOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The angle of depression of a point from the top of a 200 m high tower is $45^{\circ}$ . The distance of the point from the tower is
A $\frac{200}{\sqrt{3}} m$
B $200 m$
C $200 \sqrt{3}$ m
Dnone of these
Question 2 - Select One
The angle of depression of a ship from the top of a 30 m hight tower is $60^{\circ}$ . The distance of ship from the base of the tower is
A30m
B $30 \sqrt{3} m$
C $10 \sqrt{3} m$
D10m
Question 3 - Select One
If the angle of depression of an object from a 75 m high tower is $30^{\circ}$ , then the distance of the object from the tower is
A $25 \sqrt{3}$ m
B $50 \sqrt{3}$ m
C $75 \sqrt{3}$ m
D150 m

RS AGGARWAL-HEIGHTS AND DISTANCES-Multiple Choice Questions (Mcq)

Home
Ask Doubt
Profile