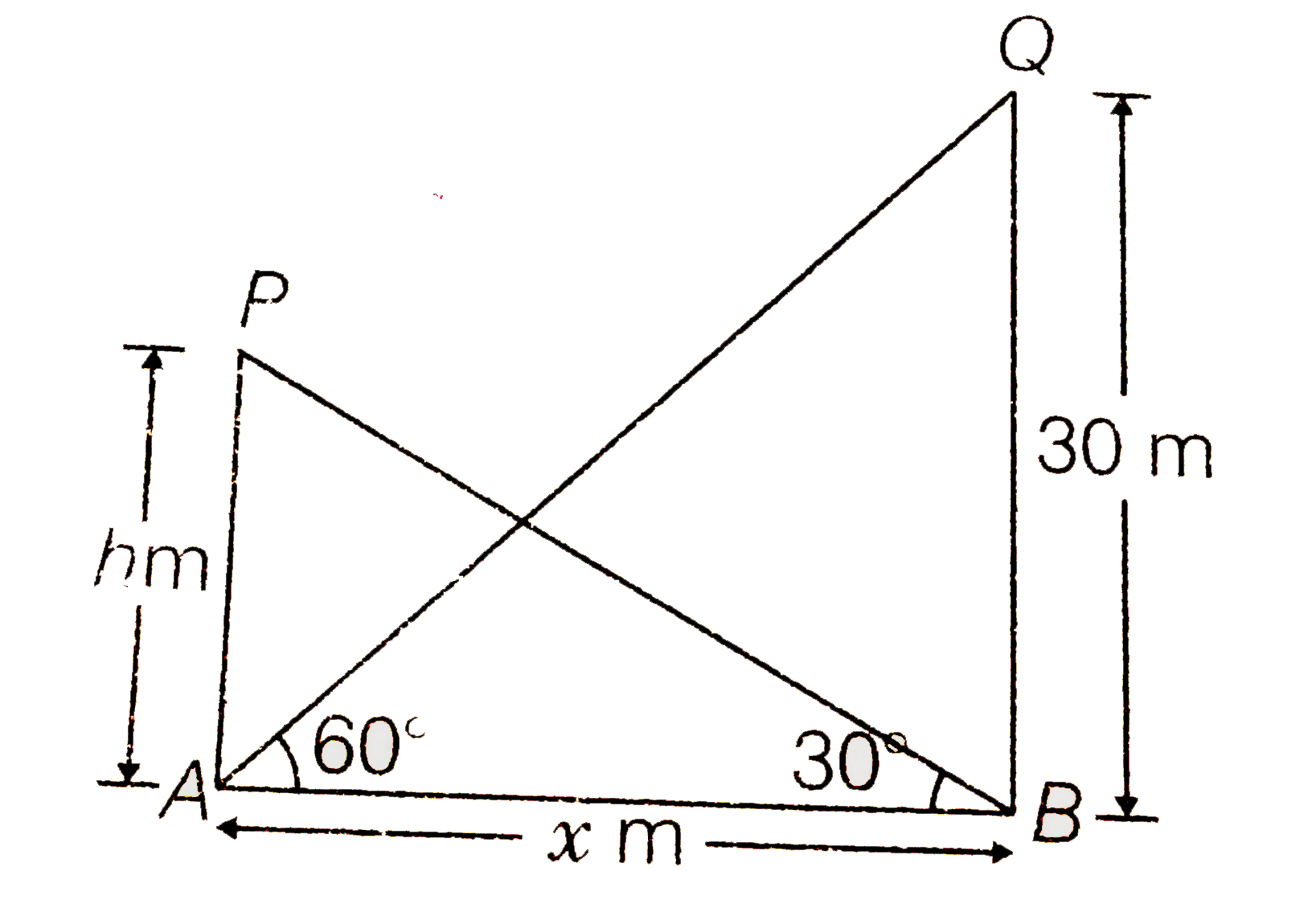
The angle of elevation of the top of a tower 30 m high from the foot of another tower in the same plane is 60∘ and the angle of elevation of the top of the second tower from the foot of the first tower is 30∘. Find the distance between the two and also the height of the tower.
Let the distance between the two towers = AB = x m and height of the other tower = PA = h m
Given that height of the tower = QB = 30 M and ∠QAB=60∘,∠PBA=30∘
Now, in ΔQAB,tan60∘=QBAB=30x
⇒√3=30x
∴x=30√3.√3√3=30√3√3=30√33=10√3m
and in ΔPBA,
tan30∘=PAAB=hx
⇒1√3=h10√3
⇒h=10m
Hence, the required distance and height are 10√3m and 10 m respectively.
Topper's Solved these Questions
Similar Questions
The angle of elevation of the top of a tower 30 m high from the foot of another tower in the same plane is 60∘ and the angle of elevation of the top of the second tower from the foot of the first tower is 30∘. Find the distance between the two towers and also the height of the other tower.
View SolutionThe angle of elevation of the top of a tower 30 m high from the foot of another tower in the same plane is 60∘ and the angle of elevation of the top of the second tower from the foot of the first tower is 30∘. The distance between the two towers is m times the height of the shorter tower. What is m equal to ?
View Solution
Knowledge Check
The angle of elevation of the top of a tower 30 m high from the foot of another tower in the same plane is 60∘ and the angle of elevation of the top iof the second tower from the foot of the first tower is 30∘. The distance between the two towers isntimes the height of the shorter tower. What is nequal to?
A√2B√3C12D13The angle of elevation of the top of a tower of height H from the foot of another tower in the same plane is 60∘ and the angle of elevation of the top of the second tower from the foot of the first tower is 30∘. If is the height of the other tower is h , then which one of the following is correct?
AA. H=2hBB. H=√3hCC. H=3hDD. None of theseThe angle of elevation of the top of a tower of height H from the foot of another tower in the same plane is 60∘ and the angle of elevation of the top of the second tower from the foot of the first tower is 30∘. If h is the height of the other tower, then which one of the following is correct?
AH=2hBH=√3hCH=3hDNone of the above
The angle of elevation of the top of a building from the foot of the tower is 30∘ and the angle of elevation of the top of the tower from the foot of the building is 45∘. If the tower is 30 m high, find the height of the building.
View SolutionThe angle of elevation of the top of a building from the foot of the tower is 30∘and the angle of elevation of the top of the tower from the foot of the building is 60∘. If the tower is 50 m high, find the height of the building.
View SolutionThe angle of elevation of the top of a building from the foot of tower is 30∘ and the angle of elevation of the top of the tower from the foot of the building is 60∘. It the tower is 30 m high, then the height of the building is
View SolutionThe angle of elevation of the top of a building from the foot of the tower is 30∘ and the angle of elevation of the top of tower from the foot of the building is 60∘,If the tower is 50 m high, find the height of the building.
View SolutionThe angle of elevation of the top of a hill from the foot of a tower is 60∘ and the angle of elevation of the top of the tower from the foot of the hill is 30∘. If the tower is 50 m high, then what is the height of the hill?
View Solution