If the angles of elevation of the top of a tower from two points at distances a and b from the base and in the same straight line with it are complementary then the height of the tower is
√ab
√ab
√a+b
√a−b
The correct Answer is:B
To solve the problem, we need to find the height of the tower given that the angles of elevation from two points at distances a and b from the base of the tower are complementary.
Let's denote:
- The height of the tower as h.
- The angle of elevation from the point at distance a as θ1.
- The angle of elevation from the point at distance b as θ2.
Since the angles are complementary, we have:
θ1+θ2=90∘
This implies:
θ2=90∘−θ1
Using the tangent function, we can express the height of the tower in terms of the angles and distances:
1. From the first point (distance a):
tan(θ1)=ha⇒h=atan(θ1)
2. From the second point (distance b):
tan(θ2)=hb⇒h=btan(θ2)
Since θ2=90∘−θ1, we can use the identity:
tan(90∘−θ1)=cot(θ1)
Thus:
tan(θ2)=cot(θ1)=1tan(θ1)
Now substituting this into the equation for h:
h=btan(θ2)=bcot(θ1)=btan(θ1)
Now we have two expressions for h:
1. h=atan(θ1)
2. h=btan(θ1)
Setting these two equations equal to each other:
atan(θ1)=btan(θ1)
Multiplying both sides by tan(θ1) (assuming tan(θ1)≠0):
atan2(θ1)=b
Now, we can solve for tan2(θ1):
tan2(θ1)=ba
Substituting this back into the expression for h:
h=atan(θ1)=a√ba=√ab
Thus, the height of the tower is:
h=√ab
- The height of the tower as h.
- The angle of elevation from the point at distance a as θ1.
- The angle of elevation from the point at distance b as θ2.
θ1+θ2=90∘
This implies:
θ2=90∘−θ1
1. From the first point (distance a):
tan(θ1)=ha⇒h=atan(θ1)
tan(θ2)=hb⇒h=btan(θ2)
tan(90∘−θ1)=cot(θ1)
Thus:
tan(θ2)=cot(θ1)=1tan(θ1)
h=btan(θ2)=bcot(θ1)=btan(θ1)
1. h=atan(θ1)
2. h=btan(θ1)
atan(θ1)=btan(θ1)
atan2(θ1)=b
tan2(θ1)=ba
h=atan(θ1)=a√ba=√ab
h=√ab
Topper's Solved these Questions
- HEIGHTS AND DISTANCESBook:RS AGGARWALChapter:HEIGHTS AND DISTANCESExercise:Exercise 14
Explore 38 Videos
- COORDINATE GEOMETRYBook:RS AGGARWALChapter:COORDINATE GEOMETRYExercise:Multiple Choice Questions (Mcq)
Explore 34 Videos
- LINEAR EQUATIONS IN TWO VARIABLES Book:RS AGGARWALChapter:LINEAR EQUATIONS IN TWO VARIABLES Exercise:QUESTION
Explore 2 Videos
Similar Questions
The angles of elevation of the top of a tower from two points at distances a and b metres from the base and in the same straight line with it are complementary. Prove that the height of the tower is √ab metres.
View SolutionIf the angles of elevation of the top of a tower from two points at a distance of 4m and 9m from the base of the tower and in the same straight line with it are complementary, find the height of the tower.
View Solution
Knowledge Check
The angles of elevation of the top of a tower from two points on the ground at distances 32 m and 18 m from its base and in the same straight line with it are complementary. The height(in m) of the tower is .....
A24B20C28D16The angles of elevation of the top of a tower from two points which are at distances of 10 m and 5 m from the base of the tower and in the same straight line with it are complementary. The height of the tower is
A5mB15mC5√2mD75m
The angles of elevation of the top of a tower from two points situated at distances 36m and 64m from its base and in the same straight line with it are complementary. What is the heigth of the tower
View SolutionIf the angles of elevation of a tower from two points distant a and b from the base and in the same straight line with it are complementary, then the height of the tower is ab (b) √ab (c) ab (d) √ab
View SolutionThe angles of elevation of the top of a tower from two points at a distance of 4 m and 9 m from the base of the tower and in the same straight line with it are complementary. Prove that the height of the tower is 6m.
View SolutionThe angles of elevation of the top of a tower from two points at a distance of 4 m and 9 m from the base of the tower and in the same straight line with it are complementary. Prove that the height of the tower is 6 m.
View SolutionThe angles of elevatio of the top of a tower from two points 8 m and 32 m from the base and in the same straight line with it are complementary. Find the heigt of the tower.
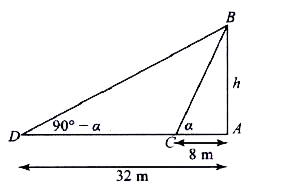
View SolutionThe angles of elevation of the top of a tower from two points which are at distances of 10 m and 5 m from the base of the tower and in the same straight line with it are complementary. The height of the tower is :
View Solution
