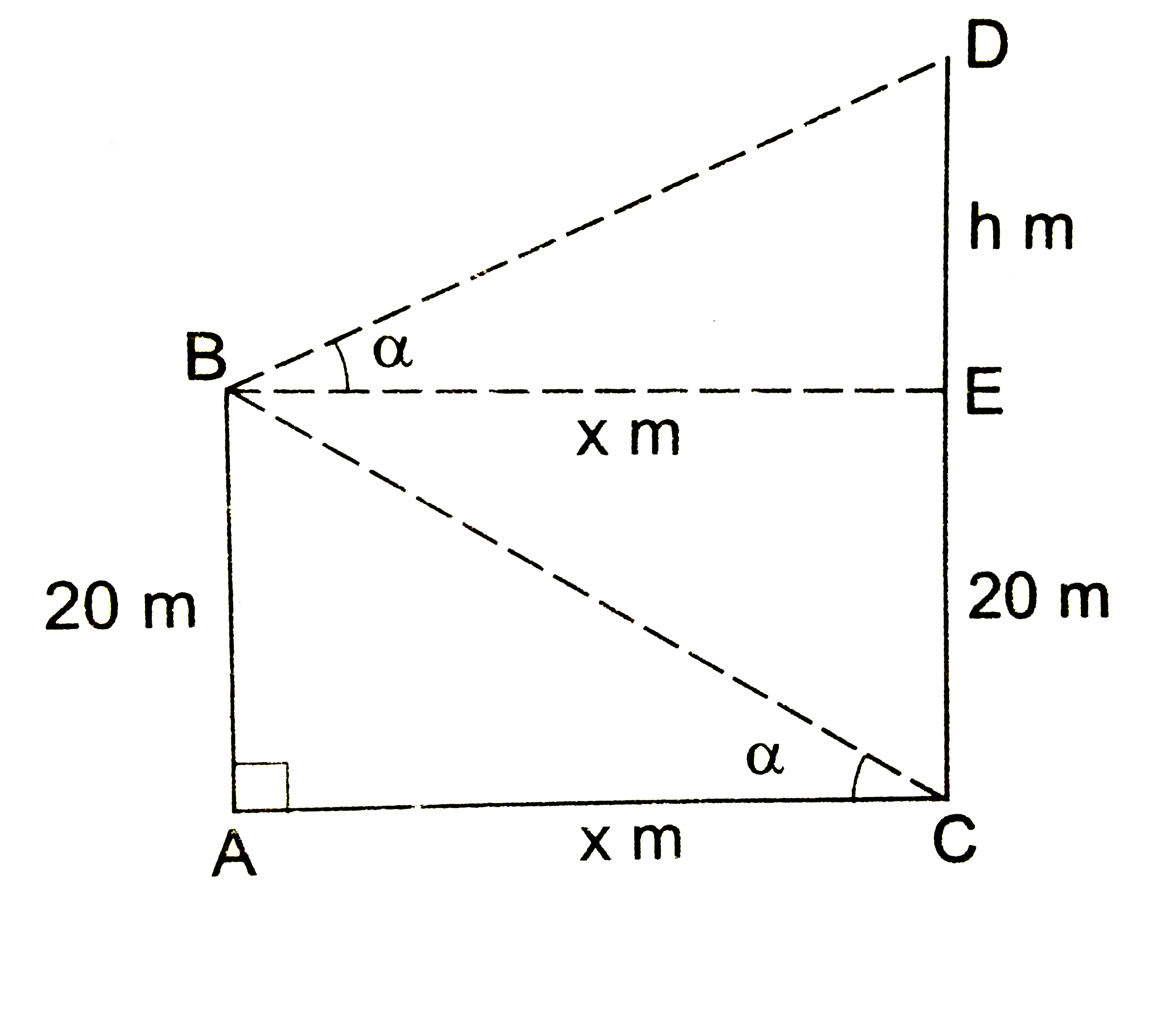
From the top of a cliff 30 metres high, the angle of elevation of the

From the top of cliff 50 m high the angle fo elevation of a tower is found to be equal ot the angle of depression of the foot of the tower. The height of the tower is …….
A50 m
B150 m
C100 m
D125 m
From the top of the house 18m high, if the angle of elevation of the top of a tower is 45° and the angle of depression of the foot of the tower is 30°, then the height of the tower is
A $18 \sqrt{3}$ m
B $18 (\sqrt{3} + 1)$ m
C $18 (\sqrt{3} - 1) m$
D $6 \sqrt{3}$ m
From the top of a 20 metre high building , the angle of elevation of the top of a tower is $60^{\circ}$ and the angle of depression of its foot is at $45^{\circ}$ , then the height of the tower is $(\sqrt{3} = 1.732)$
Aa.45 . 46 metre
Bb.45 . 64 metre
Cc.54 . 64 metre
Dd.54 . 46 metre

From the top of a cliff 30 metres high, the angle of elevation of the top of a tower is found to be equal to the angle of depression of the foot of the tower. The height of the tower is