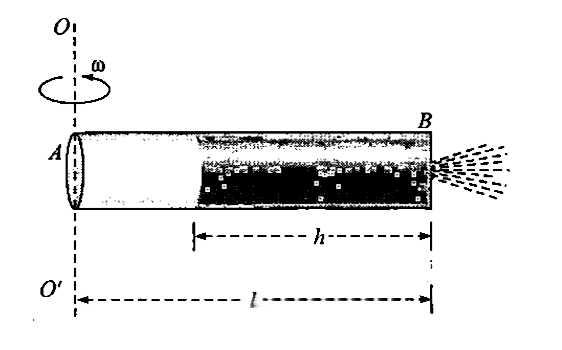
A horizontally oriented tube AB of length l rotates with a constant angular velocity $ω$ about a stationary vertical axis $O O^{'}$ passing through the end A (figure). The tube is filled with an ideal fluid. The end A of the tube is open, the closed end B has a very small orifice. Find the velocity of the fluid relative to the tube as a function of the column "height" h.

Video Solution

Text Solution

Verified by Experts

In a rotating frame (with constant angular velocity) the Eulerian equation is
$- \to V p + ρ \to g + 2 ρ (\to' v \times \to ω) + ρ ω^{2} \to r = ρ \frac{d \to' v}{d t}$
In the frame of rotating tube the liquid in the "column" is practically static because the orifice is sufficiently small. Thus the Eulerian Eq. in projection form along $\to r$ (which is the position vector of an arbitrary liquid element of length $d r$ relative to the rotation axis) reduces to
$\frac{- d p}{d r} + ρ ω^{2} r = 0$
or, $d p = ρ ω^{2} r d r$
so, $p \int p_{0} d p = ρ ω^{2} r \int (l - h) r d r$
Thus $p (r) = p_{0} + \frac{ρ ω^{2}}{2} [r^{2} - {(l - h)}^{2}]$ (1) Hence the pressure at the end B just before the orifice i.e.
$p (l) = p_{0} + \frac{ρ ω^{2}}{2} (2 l h - h^{2})$ (2)
Then applying Bernoull's theorem at the orifice for the points just inside and outside of the end B
$p_{0} + \frac{1}{2} ρ ω^{2} (2 l h - h^{2}) = p_{0} + \frac{1}{2} ρ v^{2}$ (where v is the sought velocity)
So, $v = ω h \sqrt{\frac{2 l}{h} - 1}$

Updated on:21/07/2023

A uniform rod of mass $m$ and length $l$ rotates in a horizontal plane with an angular velocity $ω$ about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
A $\frac{1}{2} m ω^{2} x$
B $\frac{1}{2} m ω^{2} (1 - \frac{x^{2}}{l})$
C $\frac{1}{2} m ω^{2} l (1 - \frac{x^{2}}{l^{2}})$
D $\frac{1}{2} m ω^{2} l [1 - \frac{x}{l}]$
A thin uniform copper rod of length l and cross-section area A and mass m rotates uniformly with an angular velocity $ω$ in a horizontal plane about a vertical axis passing through one of its ends. The elongation of the rod will be
A $\frac{m ω^{2} l^{2}}{6 A Y}$
B $\frac{m ω^{2} l^{2}}{3 A Y}$
C $\frac{m ω^{2} l^{2}}{A Y}$
D $\frac{m ω^{2} l^{2}}{2 A Y}$