A thin uniform copper rod of length l and mass m rotates uniformly with an angular velocity $ω$ in a horizontal plane about a vertical axis passing through one of its ends. Determine the tension in the rod as a function of the distance r from the rotation axis. Find the elongation of the rod.

Video Solution

Text Solution

Verified by Experts

Let us consider an element of the rod at a distance $r$ from it's rotation axis. As the element rotates in a horizontal circle of radius r, we have from Newton's second law in projection form directed toward the axis of rotation:
$T - (T + d T) = (d m) ω^{2} r$
or , $- d T = (\frac{m}{l} d r) ω^{2} r = \frac{m}{l} ω^{2} r d r$
At the free end tension becomes zero. Integrating the above expression we get, thus
$- 0 \int T d T = \frac{m}{l} ω^{2} l \int r r d r$
Thus $T = \frac{m ω^{2}}{l} (\frac{l^{2} - r^{2}}{2}) = \frac{m ω^{2} l}{2} (1 - \frac{r^{2}}{l^{2}})$
Elongation in elemental length $d r$ is given by:
$δ ξ = \frac{σ (r)}{E} d r = \frac{T}{S E} d r$
(where S is the cross sectional area of the rod and T is the tension in the rod at the considered element)
or, $δ ξ = \frac{m ω^{2} l}{2 S E} (1 - \frac{r^{2}}{l^{2}}) d r$
Thus the sought elongation
$ξ = \int d x i = \frac{m ω^{2} l}{2 S E} l \int 0 (1 - \frac{r^{2}}{l^{2}}) d r$
or, $ξ = \frac{m ω^{2} l}{2 S E} \frac{2 l}{3} = \frac{(S l ρ)}{3 S E} ω^{2} l^{3}$
$= \frac{1}{2} \frac{ρ ω^{2} l^{3}}{E}$ (where $ρ$ is the density of the copper.)

Updated on:21/07/2023

A thin uniform copper rod of length l and cross-section area A and mass m rotates uniformly with an angular velocity $ω$ in a horizontal plane about a vertical axis passing through one of its ends. The elongation of the rod will be
A $\frac{m ω^{2} l^{2}}{6 A Y}$
B $\frac{m ω^{2} l^{2}}{3 A Y}$
C $\frac{m ω^{2} l^{2}}{A Y}$
D $\frac{m ω^{2} l^{2}}{2 A Y}$
A uniform metal rod is rotated in horizontal plane about a vertical axis passing through its end at uniform rate. The tension in the rod is
Asame at all points
Bdifferent at differen points and maximum at centre of rod
Cdifferent at different points and minimum at axis of rotation.
Ddifferent at different points and maximum at axis of rotation
A thin uniform copper rod length $l$ and mass m rotates unifomly with an angular velocity $ω$ about a vertical axis passing through one of its ends as shown in the figure. Young's modulus of copper is Y. Breaking stress is $σ_{max}$ , cross sectional area of rod is A and density of rod is uniform. Based on above information, answer the following questions

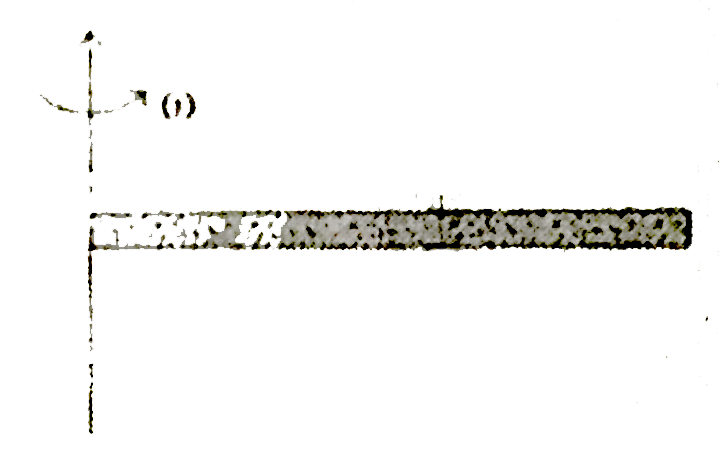
The maximum angular velocity with which the rod can rotate so that is won't break, is
A $\sqrt{\frac{2 σ_{max} A}{m l}}$
B $\sqrt{\frac{2 σ_{max} A}{3 m l}}$
C $\sqrt{\frac{σ_{max} A}{2 m l}}$
D $\sqrt{\frac{3 σ_{max} A}{m l}}$