A solid copper cylinder of length l=65cm is placed on a horizontal surface and subjected to a vertical compressive force F=1000N directed downward and distributed uniformly over the end face. What will be the resulting change of the volume of the cylinder in cubic millimeters?
Volume of a solid cylinder
V=πr2l
So, ΔVV=π2rΔrlπr2l+πr2Δlπr2l=2Δrr+Δll (1)
But longitudinal strain Δl/l and accompanying lateral strain Δr/r are related as
Δrr=−μΔll (2)
Using (2) in (1), we get:
ΔVV=Δll(1−2μ) (3)
But Δll=−F/πr2E
(Because the increment in the length of cylinder Δl is negative)
So, ΔVV=−Fπr2E(1−2μ)
Thus, ΔV=−FlE(1−2μ)
Negative sign means that the volume of the cylinder has decreased.
Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:PHYSICAL FUNDAMENTALS OF MECHANICSExercise:Hydrodynamics
Explore 25 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:PHYSICAL FUNDAMENTALS OF MECHANICSExercise:Relativistic Mechanics
Explore 49 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:PHYSICAL FUNDAMENTALS OF MECHANICSExercise:Dynamics Of A Solid Body
Explore 56 Videos
- OSCILLATIONS AND WAVESBook:IE IRODOV, LA SENA & SS KROTOVChapter:OSCILLATIONS AND WAVESExercise:Electromagnetic Waves, Radiation
Explore 36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBook:IE IRODOV, LA SENA & SS KROTOVChapter:THERMODYNAMICS AND MOLECULAR PHYSICSExercise:Transport Phenomena
Explore 38 Videos
Similar Questions
There is a uniform elastic plank moveing over a smooth horizontal plane due to a constant force F0 distributed uniformly over the front end of the plank whose area is A. If Young's modulus of the material is Y, then the compressive strain of the plank along the direction of the force is F0KAY. What is K = ?
View SolutionA uniform thin rod has mass m and length l. One end of the rod lies over rough horizontal surface and the other end is connected to a light vertical string as shown in the figure. When string is cut, there is no slipping between rod and surface.Calculate the friction force (in N) on the rod immediately after the string is cut .(Given mg=16√3N)

View Solution
Knowledge Check
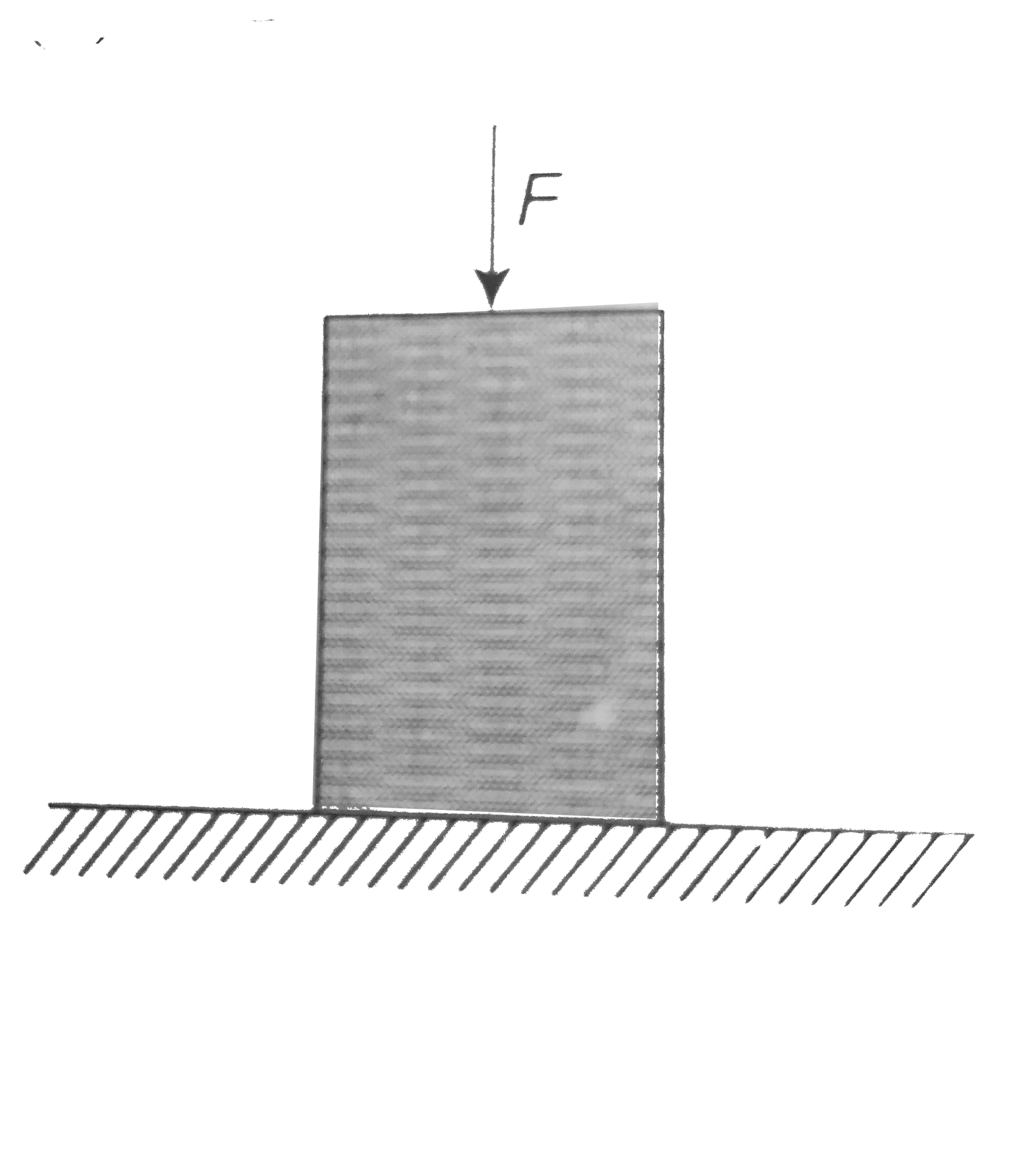
A metal cylinder of length L is subjected to a uniform compressive to a uniform compressive force F as shown in the figure. The material of the cylinder has Young's modulus Y and poisson's ratio σ The change in volume of the cylinder isAσFLYB(1−σ)FLYC(1+2σ)FLYD(1−2σ)FLYA uniform rod of mass m and length l is placed over a smooth horizontal surface along the y-axis and is at rest as shown in Fig. An impulsive force F is applied for a small time △t along x-direction at point A. The x-coordinate of end A of the rod when the rod becomes parallel to x-axis for the first time is [initially, the coordinate of centre of mass of the rod is (0,0)]
 Aπl12Bl2(1+π12)C12(1−π6)Dl2(1+π6)
Aπl12Bl2(1+π12)C12(1−π6)Dl2(1+π6)A uniform rod of mass m, length l is placed over a smooth horizontal surface along y -axis and is at rest as shown in figure. An impulsive force F is applied for a small time Δt along x-direction at point A. The x-coordinte of end A of the rod when the rod becomes parallel to x-axis for the first is (in itially the coordinates opf centre of mass of the rod is (0,0).
 Aπl12Bl2(1+π12)Cl2(1−π6)Dl2(1+π6)
Aπl12Bl2(1+π12)Cl2(1−π6)Dl2(1+π6)
A solid cylinder with r=0.1m and mass M=2kg is placed such that it is in contact with the vertical and a horizontal surface as shown in Fig. The coefficient of friction is μ=(1/3) for both the surfaces. Find the distance (in CM) from the centre of the cylinder at .which a force F=40N should be applied vertically so that the cylinder just starts rotating in anticlockwise direction.
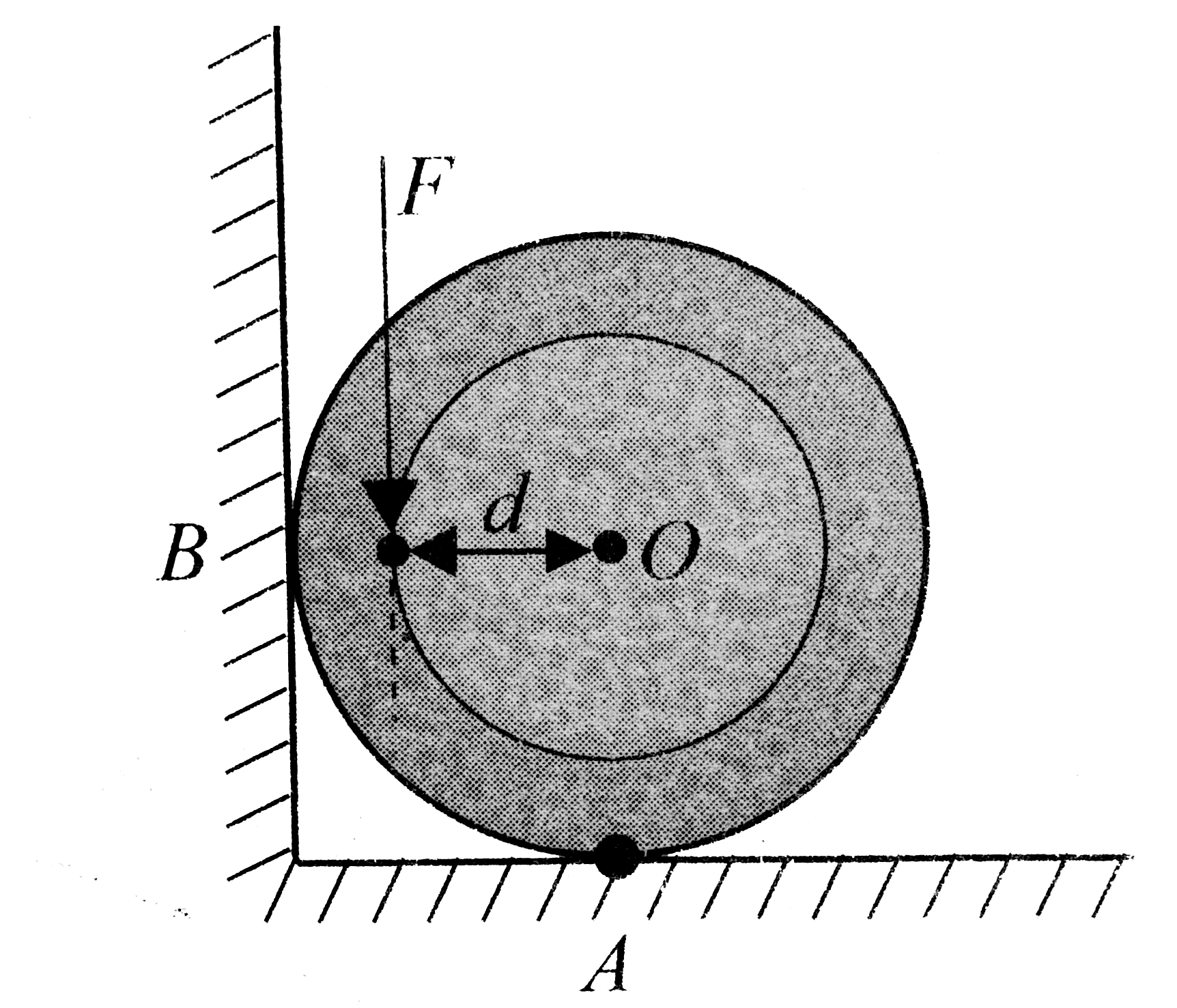
View SolutionA conducting cylinder of length L is placed on a rough horizontal floor. A horizontal magnetic field is applied perpendicular to the length of cylinder. F1 and F2 are the minimum forces required to move the cylinder for two possible directions of current, which can be passed through the cylinder. Calculate the coefficient of friction between the floor and the cylinder. Assume F1>F2.
View SolutionTwo identical particles A & B of mass m=2kg is connected at the end of the spring (K=50N/m) and placed on a smooth horizontal surface. Now, force F=10N and 2F are applied on the particles in opposite direction as shown in figure. Initially spring is in natural length.
Simultaneously particle A receives an impulse towards B which impats speed v0 to it, find v0 in m/s for which length of elongation is two times the length of compression in the spring during subsequent motion?

View SolutionA straight rod AB of mass M and length L is placed on a frictionless horizontal surface. A force having constant magnitude F and a fixed direction start acting at the end A. The rod is initially prependicular to the force. The initial acceleration of end B is
View Solution
A uniform elastic rod of cross section area A natural length L and Young's modulus Y is placed on a smooth horizontal surface. Now two horizontal forces (of magnitude F and 3F) directed along the length of rod and in opposite direction act at two of its ends as shown . After the rod has acquired steady state, the extension of the rod will be
View Solution