A ring of radius 0.1m is made out of thin metallic wire of area of cross section 10−6m2. The ring has a uniform charge of π coulombs. Find the change in the radius of the rig when a charge of 10−8C is placed at the center of the ring. Young's modulus of hte metal is 2×1011Nm−2.
The correct Answer is:2.25 mm
q=10−8C,λ=Q2πr=π2π(0.1)=5Cm−1
Take an are of ring subtending small angle θ at the center. Charge on this arc is dq=λrθ
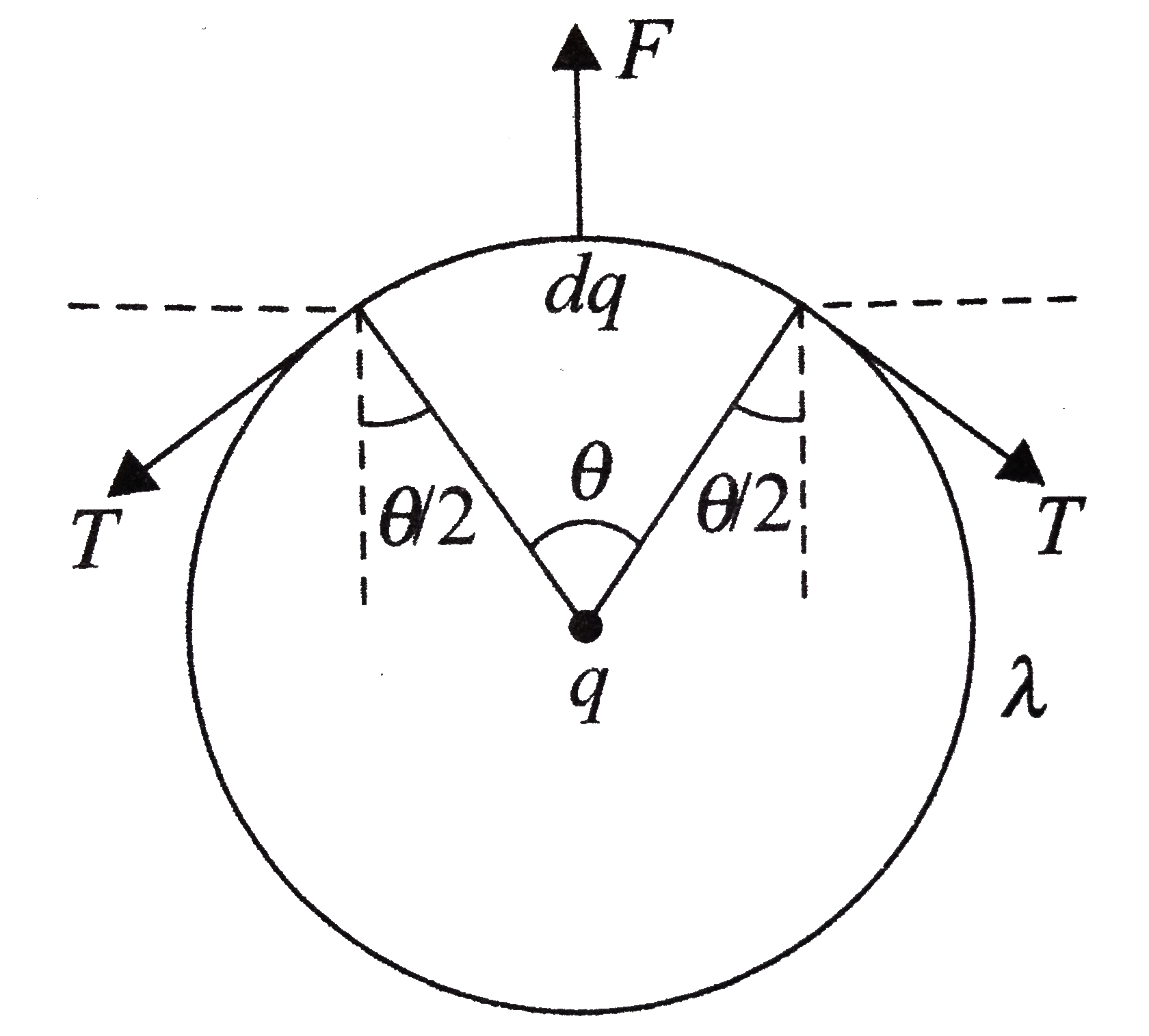
For net force to be zero on this arc,
2Tsin(θ2)=F
or, 2Tsin(θ2)=kq(λrθ)r2
or, T=kqλr
=9×109×10−8×50.1=4500N
Δr=TrAY=4500×0.110−6×2×1011
=225×10−5m=2.25mm.
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Single Correct
47 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Multiple Correct
8 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Exercises
58 Videos
- COMMUNICATION SYSTEMBOOK - CENGAGE PHYSICSCHAPTER - COMMUNICATION SYSTEMEXERCISE - QUESTION BANK
19 Videos
- Current ElectricityBOOK - CENGAGE PHYSICSCHAPTER - Current ElectricityEXERCISE - QUESTION BANK
40 Videos
Similar Questions
Knowledge Check
A light rod AC of length 2.00 m is suspended from the ceiling horizontally by means of two vertical wires of equal length tied to its ends. One of the wires is made of steel and is of cross-section 10−3m2 and the other is of brass of cross-section 2×10−3m2. The position of point D from end A along the rod at which a weight may be hung to produce equal stress in both the wires is [Young's modulus of steel is 2×1011Nm2 and for brass is 1×1011Nm−2]
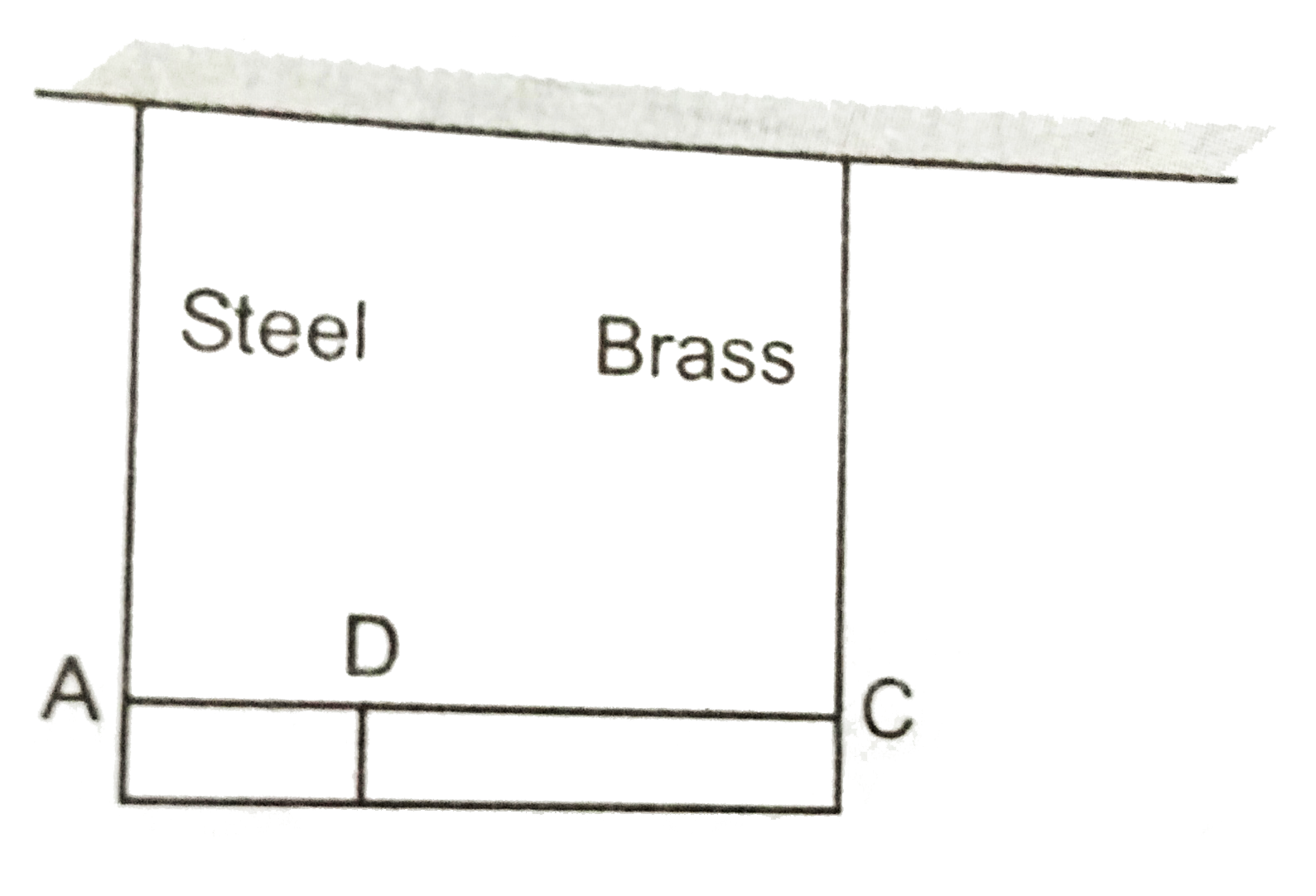 A1.00mB(2/3)mC(4/3)mD(5/3)m
A1.00mB(2/3)mC(4/3)mD(5/3)mA ring of charge with radius 0.5m has 0.002π m gap. If the ribg carries a charge of +1C the electric field at the center is
 A7.5×107NC−1B7.2×107NC−1C6.2×107NC−1D6.5×107NC−1
A7.5×107NC−1B7.2×107NC−1C6.2×107NC−1D6.5×107NC−1A wire of area of cross-section 10−6m2 is increased in length by 0.1 %. The tension produced is 1000 N. The Young's modulus of wire is
A1012N/m2B1011N/m2C1010N/m2D109N/m2
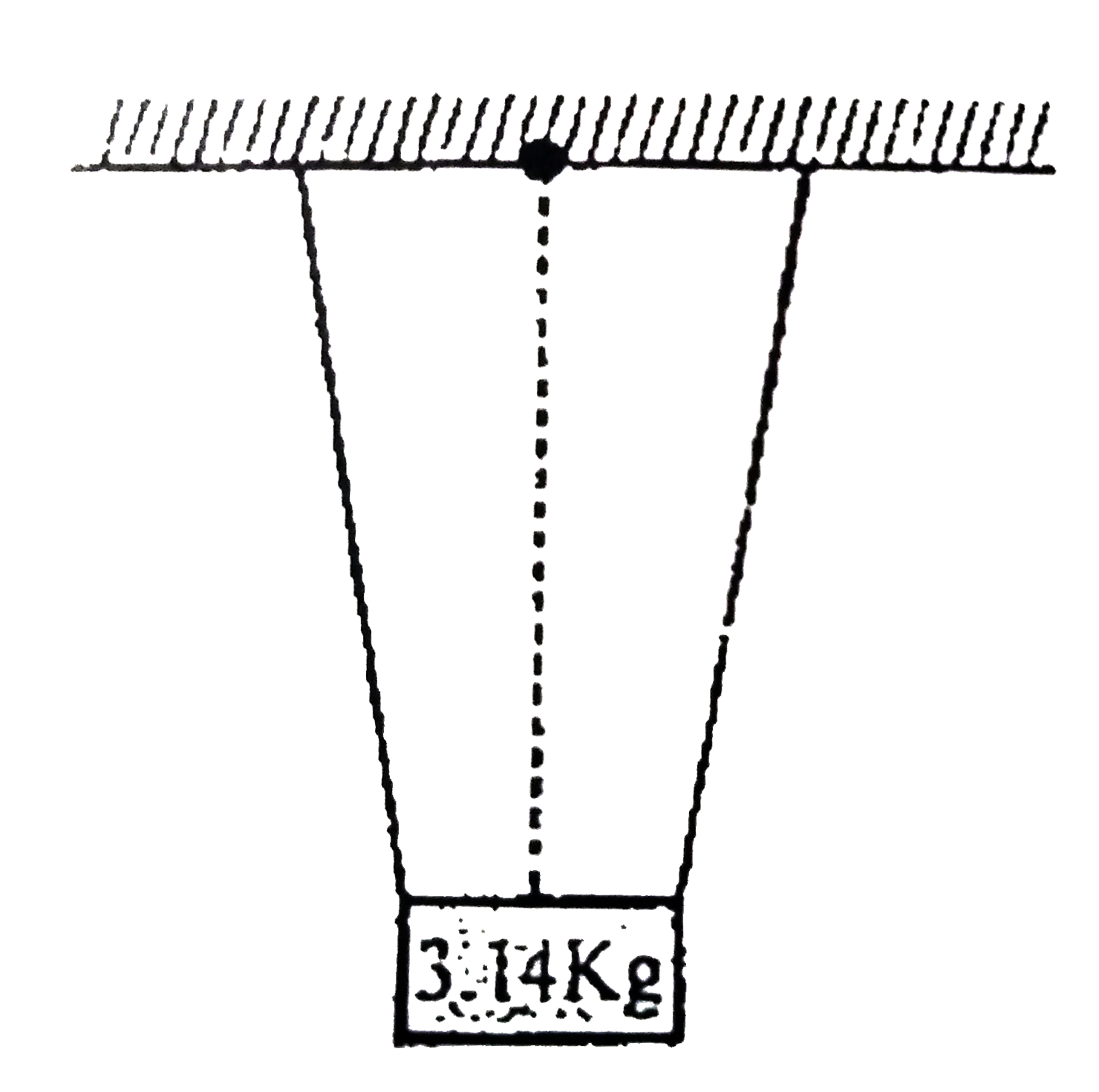
A body of mass 3.14 kg is suspended from one end of a non uniform wire of length 10m, such that the radius of the wire is changing uniformly from 9.8×10−4 m at one end to 5×10−4 m at the other end. Find the change in length of the wire in mm. Young's modulus of the material of the wire is 2×1011N/m2 (mass of wire is negligible)
View SolutionThe area of cross-section of a railway track is 0.01m2 . The temperature variation is 10∘C. Coefficient of linear expansion of material of track is 10−5/∘C . The energy stored per meter in the track is ______ J/m.
(Young's modulus of material of track is 1011Nm−2 )
View SolutionIf a uniform brass wire of length 5 m and radius 10−3 m is extended by 10−3m, then the energy stored in the wire will be
(Young's modulus for brass =10×1010N/m2)
View SolutionA semicircular ring of radius 0.5 m is uniformly charged with a total charge of 1.5×10−9 coul. The electric potential at the centre of this ring is :
View SolutionA wire of length 10 m and cross-section are 10−6m2 is stretched with a force of 20 N. If the elongation is 1 mm, the Young's modulus of material of the wire will be
View Solution
