Two mutually perpendicular long straight conductors carrying uniformly distributed charges of linear charges densities λ1 and λ2 are positon at a distance a from each other. How does the interaction between the rods depends on a ?

The correct Answer is:F=λ1λ2πε0
Let us make two - dimensional view of the situation. Beacuase of symmetry we can say the force on wire 2 (λ2) will be the along the negative y-direction.
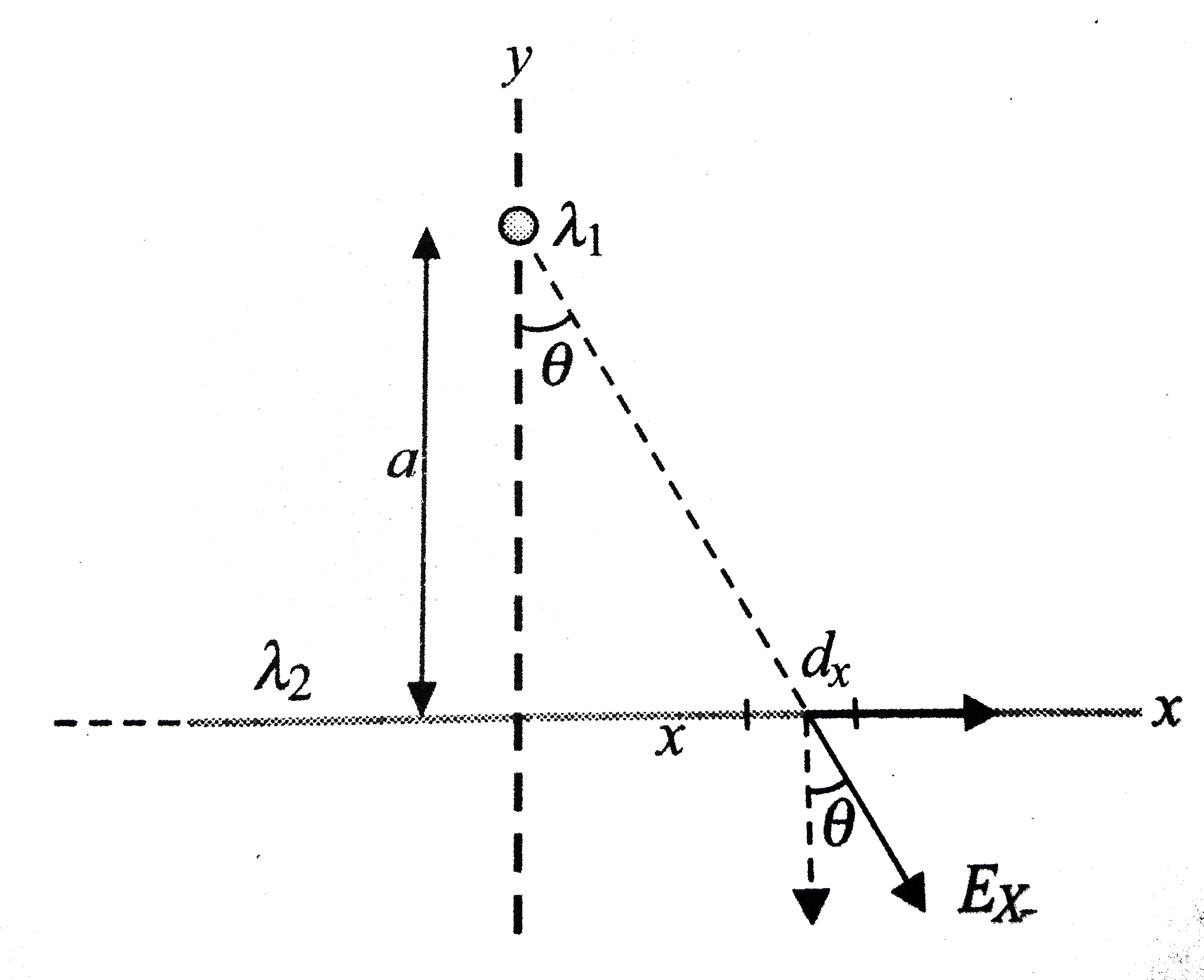
dF=Exdqcosθ
F=∫df=∫λ12πε0√a2+x2(λ2dx)cosθ
where cosθ=a√a2+x2
F=λ1λ22πε0a∫∞−∞dxa2+x2=λ1λ2a2πε0×1a[tan−1xa]∞−∞
F=λ1λ22πε0
It is independent of a.
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Single Correct
Explore 47 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Multiple Correct
Explore 8 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Exercises
Explore 58 Videos
- COMMUNICATION SYSTEMBook:CENGAGE PHYSICSChapter:COMMUNICATION SYSTEMExercise:QUESTION BANK
Explore 19 Videos
- Current ElectricityBook:CENGAGE PHYSICSChapter:Current ElectricityExercise:QUESTION BANK
Explore 40 Videos
Similar Questions
Write the expression of electric field due to an infinitely long, straight, wire having linear charge density λ at a point that is at a distance r from it.
View SolutionCalculate the potential at a point on the perpendicular bisector of uniformly charged thin rod (linear density of charge =λ) of length 2l at a distance a from the centre of the rod.
View Solution
Knowledge Check
Two parallel infinite line charges with linear charge densities +λC/m and −λ C/m are placed at a distance of 2R in free space. What is the electric field mid-way between the two line charges?
Aλ2πε0RN/CBzeroC2λπε0RN/CDλπε0RN/CTwo infinitely long thin styraight wires having uniform linear charge densities λ and 2λ are arranged parallel to each other at a distance r apart.The intensity of the electric field at a point midway between them is
A2λπε0Bλπε0rCλ2πε0rD3λ2πε0rTwo infinitely long parallel wires having linear charge densities λ1 and λ2 respectively are placed at a distance of R metres. The force per unit length on either wire will be (k=14πε0)
Ak2λ1λ2R2Bk2λ1λ2RCkλ1λ2R2Dkλ1λ2R
An infinitely long straight conductor carrying a charge with a linear density +T and a point charge −Q are at a certain distance from each other. In which of the three regions (I, II or III) are there points that (a) lie on the line passing through tho point charge perpendicular to the conductor. and (b) at which ..th~ field strength is zero 1)
View SolutionTwo mutually perpendicular infinite wires along x-axis and y-axis carry charge densities λ1 and λ2 (see figure). The 1 electric line of force at P is along the line y=1√3x, where P is also a point lying on the same line, then find λ1/λ2

View SolutionTwo mutually perpendicular infinite wires along x -axis and y -axis carry charge densities λ1 and λ2, respectively. The electric line of force at P is along, the line y=1√3x, where P is also a point lying on the same line. Find λ2λ1.
'(##CEN_KSR_PHY_JEE_C18_E01_025_Q12##)'
View SolutionA sphere of radius R is charged uniformly with a volume charge density. It is disassembled and reassembled in two identical spheres kept far from each other.
View SolutionThe electric field intensity due to a thin infinity long straight wire of uniform linear charge density λ at O is-
View Solution