If ABCD is a cyclic quadrilateral with ∠A=50∘,∠B=80∘, then Cand∠D are
100∘,130∘
115∘,115∘
110∘,120∘
130∘,100∘
The correct Answer is:D
To find the angles C and D in the cyclic quadrilateral ABCD where ∠A=50∘ and ∠B=80∘, we can use the properties of cyclic quadrilaterals.
1. Understand the Property of Cyclic Quadrilaterals:
In a cyclic quadrilateral, the sum of the opposite angles is always 180∘. This means:
∠A+∠C=180∘
∠B+∠D=180∘
2. Calculate Angle C:
We know ∠A=50∘. Using the property mentioned:
∠C=180∘−∠A
Substituting the value of ∠A:
∠C=180∘−50∘=130∘
3. Calculate Angle D:
We know ∠B=80∘. Using the property:
∠D=180∘−∠B
Substituting the value of ∠B:
∠D=180∘−80∘=100∘
4. Final Result:
Therefore, the angles are:
∠C=130∘and∠D=100∘
Summary:
- ∠C=130∘
- ∠D=100∘
In a cyclic quadrilateral, the sum of the opposite angles is always 180∘. This means:
∠A+∠C=180∘
∠B+∠D=180∘
We know ∠A=50∘. Using the property mentioned:
∠C=180∘−∠A
Substituting the value of ∠A:
∠C=180∘−50∘=130∘
We know ∠B=80∘. Using the property:
∠D=180∘−∠B
Substituting the value of ∠B:
∠D=180∘−80∘=100∘
Therefore, the angles are:
∠C=130∘and∠D=100∘
- ∠C=130∘
- ∠D=100∘
Topper's Solved these Questions
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XI)
22 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XII)
90 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IX)
5 Videos
- DISCOUNTBOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself
10 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
Similar Questions
Knowledge Check
If ABCD is a cyclic quadrilateral with ∠A=50∘,∠B=80∘ , then ∠C and ∠D are
A130∘,100∘B115∘,115∘C110∘,120∘D130∘,100∘If ABCD is a cyclic quadrilateral, then cosA+cosB is equal to
A0BcosC+cosDC−(cosC+cosD)DcosC−cosDIn a given figure, ABCD is cyclic quadrilateral, in which ∠DAB=80∘, then measures of ∠DCE is
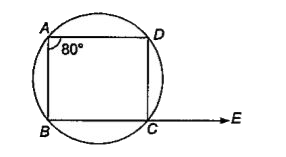 A80∘B90∘C120∘D140∘
A80∘B90∘C120∘D140∘
If ABCD is a cyclic quadrilateral in which ∠A=(x+y+10)∘,∠B=(y+30)∘,∠C=(x−y−20)∘ and ∠D=(x+y)∘. Find xandy.
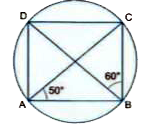
View SolutionIn Fig , ABCD is a cyclic quadrilateral . If ∠BAC=50∘and∠DBC=60∘ then find ∠BCD.
View SolutionIf ABCD is a cyclic quadrilateral, then tanA+tanC=_________.
View SolutionABCD is a cyclic quadrilateral in which: BCAD, ∠ADC=1100 and ∠BAC=500. Find ∠DAC ∠DBC=800 and ∠BAC=400. Find ∠BCD ∠BCD=1000and ∠ABD=700 find ∠ADB
View SolutionIn Figure, ABCD is a cyclic quadrilateral. Find the value of x
View Solution
