ABCD is a cyclic quadrilateral. AB and DC are produced to meet at P. If ∠ADC=70∘ and ∠DAB=60∘, then the ∠PBC+∠PCB is
130∘
150∘
155∘
180∘
The correct Answer is:A
To solve the problem step by step, we will use the properties of cyclic quadrilaterals and the angles formed when lines are extended.
Step 1: Identify the angles in the cyclic quadrilateral
Given that ∠ADC=70∘ and ∠DAB=60∘.
Step 2: Calculate the opposite angles
In a cyclic quadrilateral, the opposite angles sum up to 180∘.
- Therefore, we can find ∠ABC as follows:
∠ABC=180∘−∠ADC=180∘−70∘=110∘
- Similarly, we can find ∠CDA:
∠CDA=180∘−∠DAB=180∘−60∘=120∘
Step 3: Analyze the angles at point P
Since AB and CD are extended to meet at point P, we can analyze the angles formed:
- The angle ∠PBC is equal to ∠ABC because they are vertically opposite angles. Thus:
∠PBC=110∘
- The angle ∠PCB is equal to ∠CDA because they are also vertically opposite angles. Thus:
∠PCB=120∘
Step 4: Calculate the sum of angles ∠PBC+∠PCB
Now, we can find the sum of the angles:
∠PBC+∠PCB=110∘+120∘=230∘
Conclusion
Thus, the sum ∠PBC+∠PCB is 230∘.
Given that ∠ADC=70∘ and ∠DAB=60∘.
In a cyclic quadrilateral, the opposite angles sum up to 180∘.
- Therefore, we can find ∠ABC as follows:
∠ABC=180∘−∠ADC=180∘−70∘=110∘
- Similarly, we can find ∠CDA:
∠CDA=180∘−∠DAB=180∘−60∘=120∘
Since AB and CD are extended to meet at point P, we can analyze the angles formed:
- The angle ∠PBC is equal to ∠ABC because they are vertically opposite angles. Thus:
∠PBC=110∘
- The angle ∠PCB is equal to ∠CDA because they are also vertically opposite angles. Thus:
∠PCB=120∘
Now, we can find the sum of the angles:
∠PBC+∠PCB=110∘+120∘=230∘
Thus, the sum ∠PBC+∠PCB is 230∘.
Topper's Solved these Questions
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XI)
Explore 22 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XII)
Explore 90 Videos
- GEOMETRYBook:KIRAN PUBLICATIONChapter:GEOMETRYExercise:QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IX)
Explore 5 Videos
- DISCOUNTBook:KIRAN PUBLICATIONChapter:DISCOUNTExercise:Test Yourself
Explore 10 Videos
- LCM AND HCF Book:KIRAN PUBLICATIONChapter:LCM AND HCF Exercise:Test Yourself
Explore 18 Videos
Similar Questions
Knowledge Check
ABCD is a cyclic quadrilateral. AB and DC are produced to meet at P. If ∠ADC=70degreeand∠DAB=60degree, then the ∠PBC+∠PCB is
A130∘B150∘C155∘D180∘ABCD is a cyclic quadrilateral whose diagonals intersect at P. If AB=BC,∠DBC=70∘and∠BAC=30∘, then the measure of ∠PCD is :
A35∘B50∘C55∘D30∘ABCD is a cyclic quadrilateral. The side AB is extended to E in such a way that BE =BC. If ∠ADC=70∘,∠BAD=95∘, then ∠DCE is equal to
A140∘B120∘C165∘D110∘
ABCD is a cyclic quadrilateral. The side AB is extended to E in such a way that BE =BC. If ∠ADC=70∘,∠BAD=95∘, then ∠DCE is equal to
View SolutionABCD is a cyclic quadrilateral. Diagonals AC and BD meets at P. If ∠APB=110∘ and ∠CBD=30∘, then ∠ADB measures
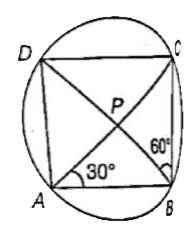
View SolutionIn the given figure, ABCD is a cyclic quadrilateral and diagonals D bisect each other at P. If ∠DBC=60∘and∠BAC=30∘, then ∠BCD is:
View SolutionA dog takes 3 leaps for every 5 leaps of a hare. If one leap of the dog is equal to 3 leaps of the hare, the ratio of the speed of the dog to that of the hare is :
View SolutionABCD is a cyclic quadrilateral in which sides AD and BC are produced to meet at P, and sides DC and AB meet at Q when produced. If ∠A=60∘ and ∠ABC=72∘ then ∠DPC−∠BQC=
ABCD एक चक्रीय चतुर्भुज है जिसमें भुजाओं AD तथा BC को बढ़ाया जाता है जो P पर मिलती हैं तथा भुजाओं DC और AB को बढ़ाया जाता है जो Q पर मिलती हैं | यदि ∠A=60∘ तथा ∠ABC=72∘ है, तो ∠DPC−∠BQC= ?
View Solution