ABCD is a cyclic quadrilateral. The side AB is extended to E in such a way that BE =BC. If ∠ADC=70∘,∠BAD=95∘, then ∠DCE is equal to
140∘
120∘
165∘
110∘
The correct Answer is:A
To solve the problem, we will follow these steps:
Step 1: Understand the Cyclic Quadrilateral Properties
A cyclic quadrilateral is a quadrilateral whose vertices lie on a circle. The opposite angles of a cyclic quadrilateral sum up to 180 degrees.
Step 2: Identify Given Angles
We are given:
- Angle ∠ADC=70∘
- Angle ∠BAD=95∘
Step 3: Find Angle ∠BCD
Using the property of cyclic quadrilaterals, we can find angle ∠BCD:
∠BAD+∠BCD=180∘
Substituting the known value:
95∘+∠BCD=180∘
∠BCD=180∘−95∘=85∘
Step 4: Find Angle ∠ACD
Next, we can find angle ∠ACD using the property of opposite angles:
∠ADC+∠ACD=180∘
Substituting the known value:
70∘+∠ACD=180∘
∠ACD=180∘−70∘=110∘
Step 5: Analyze Triangle BEC
Since BE=BC, triangle BEC is isosceles with BE=BC. Therefore, the angles opposite these equal sides are equal:
Let ∠EBC=x. Then,
∠ECB=x
The sum of angles in triangle BEC is:
∠EBC+∠ECB+∠BEC=180∘
Substituting the known values:
x+x+∠BEC=180∘
2x+∠BEC=180∘
∠BEC=180∘−2x
Step 6: Relate Angles
We know that:
∠ACD=∠BEC
Thus,
110∘=180∘−2x
Solving for x:
2x=180∘−110∘
2x=70∘
x=35∘
Step 7: Find Angle ∠DCE
Now, we can find angle ∠DCE:
∠DCE=∠ACD−∠ECB
Substituting the known values:
∠DCE=110∘−35∘=75∘
Final Answer
Thus, the angle ∠DCE is equal to 75∘.
---
A cyclic quadrilateral is a quadrilateral whose vertices lie on a circle. The opposite angles of a cyclic quadrilateral sum up to 180 degrees.
We are given:
- Angle ∠ADC=70∘
- Angle ∠BAD=95∘
Using the property of cyclic quadrilaterals, we can find angle ∠BCD:
∠BAD+∠BCD=180∘
Substituting the known value:
95∘+∠BCD=180∘
∠BCD=180∘−95∘=85∘
Next, we can find angle ∠ACD using the property of opposite angles:
∠ADC+∠ACD=180∘
Substituting the known value:
70∘+∠ACD=180∘
∠ACD=180∘−70∘=110∘
Since BE=BC, triangle BEC is isosceles with BE=BC. Therefore, the angles opposite these equal sides are equal:
Let ∠EBC=x. Then,
∠ECB=x
The sum of angles in triangle BEC is:
∠EBC+∠ECB+∠BEC=180∘
Substituting the known values:
x+x+∠BEC=180∘
2x+∠BEC=180∘
∠BEC=180∘−2x
We know that:
∠ACD=∠BEC
Thus,
110∘=180∘−2x
Solving for x:
2x=180∘−110∘
2x=70∘
x=35∘
Now, we can find angle ∠DCE:
∠DCE=∠ACD−∠ECB
Substituting the known values:
∠DCE=110∘−35∘=75∘
Thus, the angle ∠DCE is equal to 75∘.
Topper's Solved these Questions
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XI)
22 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE- XII)
90 Videos
- GEOMETRYBOOK - KIRAN PUBLICATIONCHAPTER - GEOMETRYEXERCISE - QUESTIONS ASKED IN PREVIOUS SSC EXAMS (TYPE-IX)
5 Videos
- DISCOUNTBOOK - KIRAN PUBLICATIONCHAPTER - DISCOUNTEXERCISE - Test Yourself
10 Videos
- LCM AND HCF BOOK - KIRAN PUBLICATIONCHAPTER - LCM AND HCF EXERCISE - Test Yourself
18 Videos
Similar Questions
In the adjoining figure, ABCD is a cyclic quadrilateral whose side AB is the diameter of the circle . If ∠ADC=140∘ , then find the value of ∠BAC.

View SolutionIn Figure, ABCD is a cyclic quadrilateral whose side AB is a diameter of the circle through A, B, C, D. If (∠ADC)=1300, find ∠BAC
View Solution
Knowledge Check
ABCD is a cyclic quadrilateral. The side AB is extended to E in such a way that BE =BC. If ∠ADC=70∘,∠BAD=95∘, then ∠DCE is equal to
A140∘B120∘C165∘D110∘If ABCD is a cyclic quadrilateral, then cosA+cosB is equal to
A0BcosC+cosDC−(cosC+cosD)DcosC−cosDIn a cyclic quadrilateral ABCD, the side AB is extended to a point X. If ∠XBC=82∘ and ∠ADB=47∘ , then the value of ∠BDC is
A40∘B35∘C30∘D25∘
ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and angle BAC= 50∘. Then angle ADC is equal to:
ABCD एक ऐसा चक्रीय चतुर्भुज है कि AB इसे घेरने वाले वृत्त का व्यास ह तथा कोण BAC= 50∘ है| कोण ADC का मान ज्ञात करें |
View SolutionIn the adjoining figure, ABCD is a cyclic quadrilateral.If side BC is produced upto point E and ∠DAB=95∘, then find the value of ∠DCE.

View SolutionABCD is a cyclic quadrilateral. AB and DC are produced to meet at P. If ∠ADC=70∘ and ∠DAB=60∘, then the ∠PBC+∠PCB is
View SolutionABCD is a cyclic quadrilateral whose diagonals intersect at P. If AB=BC,∠DBC=70∘and∠BAC=30∘, then the measure of ∠PCD is :
View SolutionABCD is a cyclic quadrilateral whose side AB is a diameter of the circle through A, B, C, D. If ∠ADC=130∘, find ∠BAC.
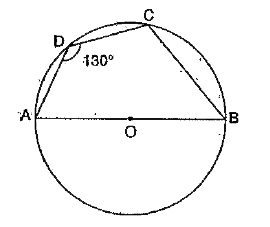
View Solution
