A solid spherical conductor of radius R has a spherical cavity of radius at its centre. A charge is kept at the centre. The charge at the inner surface, outer surface are respectively.
+Q,−Q
−Q,+Q
Q,−Q
+Q,0
The correct Answer is:B
To solve the problem, we need to analyze the situation involving a solid spherical conductor with a cavity and a charge placed at the center. We will use Gauss's law to determine the charges induced on the inner and outer surfaces of the conductor.
1. Understanding the Setup:
- We have a solid spherical conductor of radius R.
- There is a spherical cavity of radius A at the center of the conductor.
- A charge +Q is placed at the center of the cavity.
2. Applying Gauss's Law Inside the Cavity:
- Inside the cavity, the electric field E is zero because the conductor shields the cavity from external electric fields.
- According to Gauss's law, the electric flux Φ through a closed surface is given by:
Φ=E⋅A
- Since E=0, the electric flux Φ is also zero. Thus, the charge enclosed by any Gaussian surface inside the cavity must also be zero.
3. Determining Charge on the Inner Surface:
- Since there is a charge +Q at the center, to maintain the zero electric field inside the cavity, the inner surface of the conductor must have an induced charge of −Q.
- This is because the total enclosed charge must equal zero, so:
Qenclosed=+Q+Qinner surface=0⟹Qinner surface=−Q
4. Determining Charge on the Outer Surface:
- The conductor as a whole must remain electrically neutral. If the inner surface has a charge of −Q, the total charge on the conductor must balance this.
- Therefore, the outer surface must have a charge of +Q to ensure that the total charge of the conductor remains zero.
- Thus, the charge on the outer surface is:
Qouter surface=+Q
5. Final Answer:
- The charge at the inner surface is −Q and the charge at the outer surface is +Q.
Summary of Charges:
- Charge at the inner surface: −Q
- Charge at the outer surface: +Q
- We have a solid spherical conductor of radius R.
- There is a spherical cavity of radius A at the center of the conductor.
- A charge +Q is placed at the center of the cavity.
- Inside the cavity, the electric field E is zero because the conductor shields the cavity from external electric fields.
- According to Gauss's law, the electric flux Φ through a closed surface is given by:
Φ=E⋅A
- Since E=0, the electric flux Φ is also zero. Thus, the charge enclosed by any Gaussian surface inside the cavity must also be zero.
- Since there is a charge +Q at the center, to maintain the zero electric field inside the cavity, the inner surface of the conductor must have an induced charge of −Q.
- This is because the total enclosed charge must equal zero, so:
Qenclosed=+Q+Qinner surface=0⟹Qinner surface=−Q
- The conductor as a whole must remain electrically neutral. If the inner surface has a charge of −Q, the total charge on the conductor must balance this.
- Therefore, the outer surface must have a charge of +Q to ensure that the total charge of the conductor remains zero.
- Thus, the charge on the outer surface is:
Qouter surface=+Q
- The charge at the inner surface is −Q and the charge at the outer surface is +Q.
- Charge at the inner surface: −Q
- Charge at the outer surface: +Q
Topper's Solved these Questions
- ELECTROSTATICSBook:VMC MODULES ENGLISHChapter:ELECTROSTATICSExercise:LEVEL - 2
Explore 60 Videos
- ELECTROSTATICSBook:VMC MODULES ENGLISHChapter:ELECTROSTATICSExercise:JEE MAIN
Explore 65 Videos
- ELECTROSTATICSBook:VMC MODULES ENGLISHChapter:ELECTROSTATICSExercise:LEVEL - 0 (LONG ANSWER TYPE )
Explore 8 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT Book:VMC MODULES ENGLISHChapter:ELECTROMAGNETIC INDUCTION & ALTERNATIVE CURRENT Exercise:IMPECCABLE
Explore 52 Videos
- ENERGY & MOMENTUMBook:VMC MODULES ENGLISHChapter:ENERGY & MOMENTUMExercise:JEE ADVANCE (ARCHIVE) - TRUE/FALSE TYPE
Explore 1 Video
Similar Questions
A solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distanace R/4 from the centre of sphere. The gravitational force on mass m is
View SolutionA solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distance R/4 from the centre of sphere. The gravitational force on mass m is
View SolutionA spherical body of radius 'b' has a concentric cavity of radius 'a' as shown. Thermal conductivity of the material is K. Find thermal resistance between inner surface P and outer surface Q.
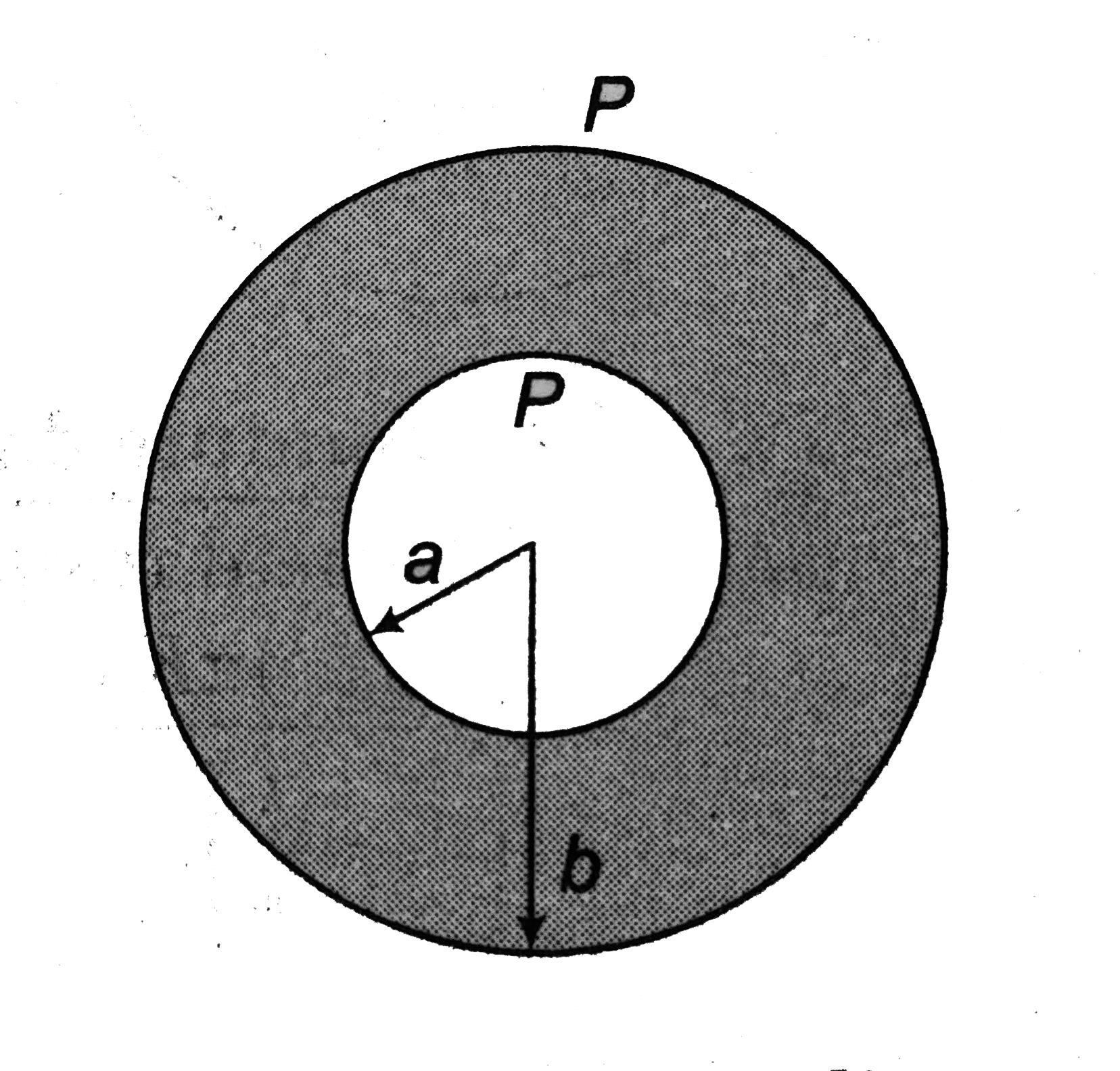
View SolutionA solid sphere of radius R has a spherical cavity of radius r as shown in the figure. If the volume charge density σ is uniformly distributed over the whole volume of the sphere, then electric field strength at the centre of the sphere will be
View SolutionA point charge is kept at the centre of a metallic insulated spherical shell. Then
View SolutionA point charge is kept at the centre of a metallic insulated spherical shell. Then
View SolutionA spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
(a) A charge q is placed at the center of the shell. What is the surface charge density on the inner and outer surfaces of the shell ?
(b) Is the electric field intensity inside a cavity (with no charge) zero, even if the shell is not spherical, but has any irregular shape ? Explain.
View SolutionStatement-1 : A hollow metallic sphere of inner radius a and outer radius b has charge q at the centre. A negatively charged moves from inner surface outer surface. Then total work done will be zero.
Statement-2: Potential is constant inside the metallic sphere.
View SolutionA conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
If q1 is shifted to the centre of the cavity, then: (choose the correct alternative)
View SolutionA conducting sphere of radius R2 has a spherical cavity of radius R1 which is non concentric with the sphere. A point charge q1 is placed at a distance r from the centre of the cavity. For this arrangement, answer the following questions.
Electric potential at the centre of the cavity will be :
View Solution