A point charge is kept at the centre of a metallic insulated spherical shell. Then
Electric field out side the sphere is zero
Electric field inside the sphere is zero
Net induced charge on the sphere is zero
Electric potential inside the sphere is zero.
The correct Answer is:C
To solve the problem of a point charge placed at the center of a metallic insulated spherical shell, we can follow these steps:
Step 1: Understand the Configuration
We have a point charge q located at the center of a metallic insulated spherical shell. The shell is conductive and insulated, meaning it can redistribute charges but does not allow external electric fields to influence the charge distribution.
Hint: Remember that in conductors, charges can move freely and will redistribute themselves in response to an electric field.
Step 2: Induced Charges on the Inner Surface
Due to the presence of the point charge q at the center, the inner surface of the spherical shell will have an induced charge. This induced charge will be equal in magnitude but opposite in sign to the point charge, resulting in an induced charge of −q on the inner surface.
Hint: The induced charge on the inner surface of a conductor is always equal and opposite to the charge inside it.
Step 3: Induced Charges on the Outer Surface
Since the shell is neutral overall and has an induced charge of −q on the inner surface, the outer surface of the shell must have an induced charge of +q to maintain the neutrality of the entire shell.
Hint: The total charge of a conductor must remain constant; any induced charge on one surface must be balanced by an equal and opposite charge on another surface.
Step 4: Electric Field Inside the Shell
Inside the metallic shell (between the inner surface and the outer surface), the electric field is zero. This is because the charges on the inner surface redistribute themselves in such a way that they cancel out any electric field within the conducting material.
Hint: In electrostatics, the electric field inside a conductor in electrostatic equilibrium is always zero.
Step 5: Electric Field Outside the Shell
To find the electric field outside the shell, we can use Gauss's law. The total charge enclosed by a Gaussian surface outside the shell is q (the point charge) plus −q (the induced charge on the inner surface) plus +q (the induced charge on the outer surface). This results in a net charge of q enclosed by the Gaussian surface.
Hint: Use Gauss's law, which states that the electric flux through a closed surface is proportional to the enclosed charge.
Step 6: Conclusion
The electric field outside the shell is not zero and behaves as if there is a point charge q located at the center of the shell. The net induced charge on the shell itself is zero, as the positive and negative charges balance each other out.
Final Answer: The net induced charge on the spherical shell is zero.
We have a point charge q located at the center of a metallic insulated spherical shell. The shell is conductive and insulated, meaning it can redistribute charges but does not allow external electric fields to influence the charge distribution.
Due to the presence of the point charge q at the center, the inner surface of the spherical shell will have an induced charge. This induced charge will be equal in magnitude but opposite in sign to the point charge, resulting in an induced charge of −q on the inner surface.
Since the shell is neutral overall and has an induced charge of −q on the inner surface, the outer surface of the shell must have an induced charge of +q to maintain the neutrality of the entire shell.
Inside the metallic shell (between the inner surface and the outer surface), the electric field is zero. This is because the charges on the inner surface redistribute themselves in such a way that they cancel out any electric field within the conducting material.
To find the electric field outside the shell, we can use Gauss's law. The total charge enclosed by a Gaussian surface outside the shell is q (the point charge) plus −q (the induced charge on the inner surface) plus +q (the induced charge on the outer surface). This results in a net charge of q enclosed by the Gaussian surface.
The electric field outside the shell is not zero and behaves as if there is a point charge q located at the center of the shell. The net induced charge on the shell itself is zero, as the positive and negative charges balance each other out.
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise-II
56 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Practice Exercise
57 Videos
- ELECTRIC CHARGES AND FIELDSBOOK - AAKASH SERIESCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Assess Your Self
13 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
Similar Questions
A point charge +q is placed at the centre of a conducting spherical shell of inner radius a and outer radius b. Find the charge appearing on the inner and outer surface of the shell.
View SolutionA ball with charge −50e is placed at the centre of a hollow spherical shell has a net charge of −50e. What is the charge on the shell's outer surface ?
View SolutionA point charge Q is placed at the centre of a spherical conducting shell. A total charge of -q is placed on the shell. The magnitude of the electric field at point P1 at a distance R1 from the centre is X. The magnitude of the electric field due to induced charges at point P2 a distance R2 from the centre is Y. The values of X and Y are respectively.

View SolutionA point charge +Q is placed at the centre of an isolated conducting shell of radius R. Find the electrostatic potential energy stored outside the spherical shell if the shell also contains charge +Q distributed uniformly over it.
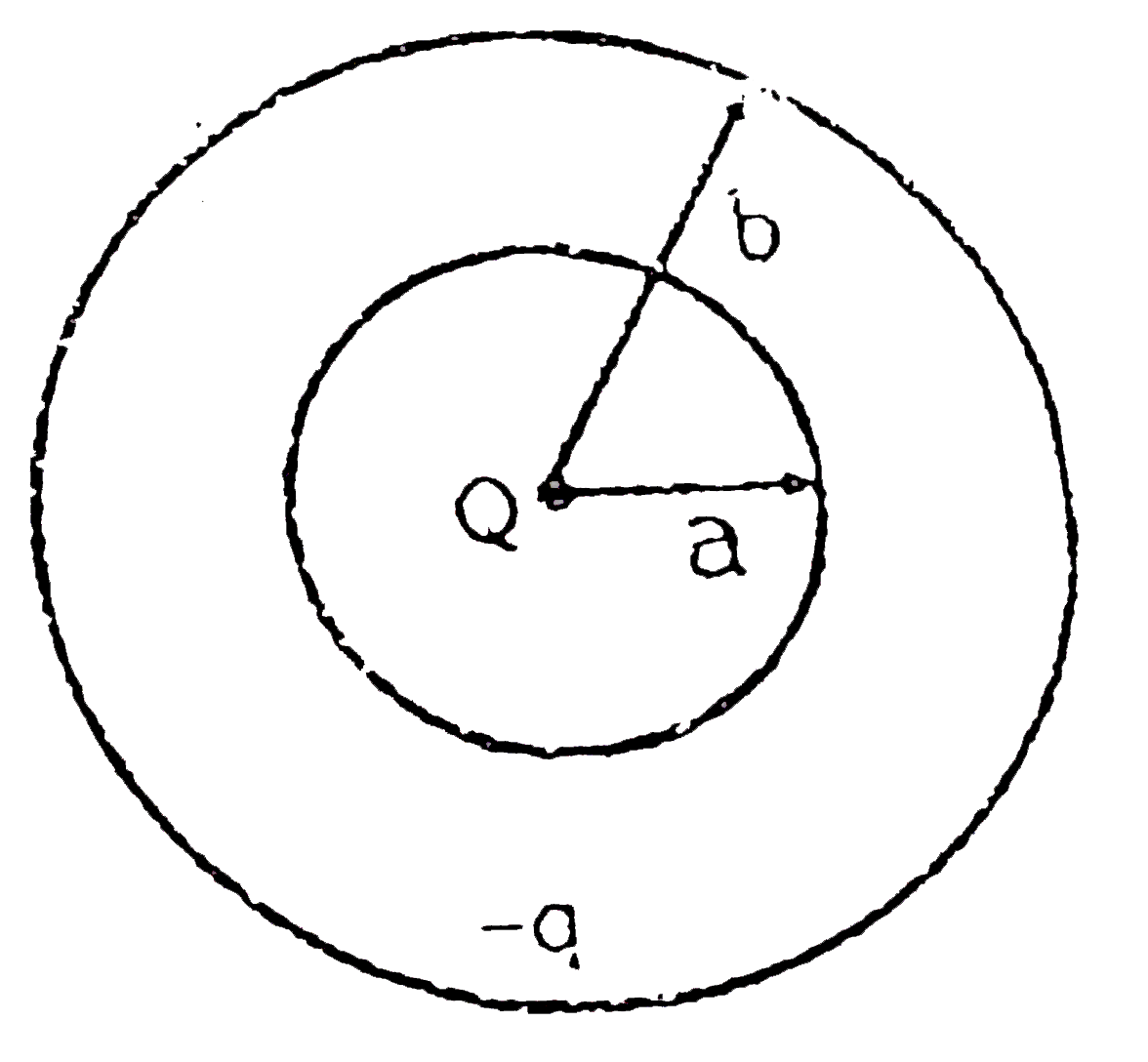
View SolutionShown in the figure a spherical shell with an inner radius 'a' and an outer radius 'b' is made of conducting metarial. A charge +Q is placed at the centre of the spherical shell and a total charge -q s placed on the shell. Charge -q is districuted on the surfaces as :
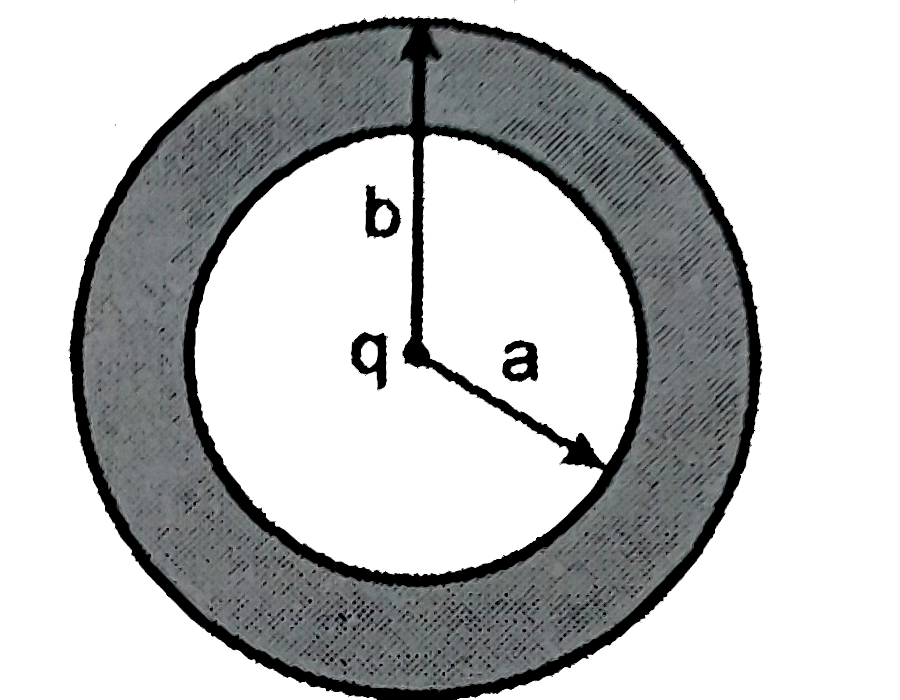
View SolutionA point charge q is brought slowly from infinity and is placed at the centre of a conducting neutral spherical shell of inner radius a and outer radius b, then find work done by external agent :
View SolutionA thin. Metallic spherical shell contains charge Q on it. A point charge q is placed at the centre of the shell and another charge q1 is placed outside it as shown in figure . All the three charges are positive. The force on the charge at the centre is

View SolutionA metallic spherical shell of radius R has a charge -Q on it. A point charge +Q is placed at the centre of the shell. Which of the graphs shown below may correctly represent the variation of electric field E with distance r from the centre of shell ?
View SolutionA thin metallic spherical shell carries a charge Q on it. A point charge Q1 is placed at the centre of the shell and another charge q is placed outside as shown in figure ahead. All the charges are positive . Discuss the various forces acting on Q1.

View SolutionA point charge .q. is placed at the centre of an uncharged conducting shell of inner radius R and outer radius 2R. If the point charge is removed from the centre of the shell, to infinity (without any electric contact with the shell). Work done by electric force is
View Solution
