A spherical conducting shell of inner radius r1 and outer radius r2 has a charge Q.
(a) A charge q is placed at the center of the shell. What is the surface charge density on the inner and outer surfaces of the shell ?
(b) Is the electric field intensity inside a cavity (with no charge) zero, even if the shell is not spherical, but has any irregular shape ? Explain.
To solve the problem step by step, we will address both parts of the question regarding the spherical conducting shell.
Part (a): Surface Charge Density on the Inner and Outer Surfaces
1. Understanding the Setup:
- We have a spherical conducting shell with inner radius r1 and outer radius r2.
- The shell has a total charge Q.
- A charge q is placed at the center of the shell.
2. Charge Induction on the Inner Surface:
- When charge q is placed at the center, it induces a charge of −q on the inner surface of the shell. This is because the conducting material will rearrange its charges to maintain electrostatic equilibrium.
- Therefore, the total charge on the inner surface, Qinner=−q.
3. Calculating Surface Charge Density on the Inner Surface:
- The surface charge density σ1 on the inner surface is given by:
σ1=QinnerArea of inner surface=−q4πr21
4. Charge Induction on the Outer Surface:
- The total charge on the shell is Q. Since −q is on the inner surface, the remaining charge on the outer surface must be:
Qouter=Q+q
5. Calculating Surface Charge Density on the Outer Surface:
- The surface charge density σ2 on the outer surface is given by:
σ2=QouterArea of outer surface=Q+q4πr22
Summary for Part (a):
- The surface charge density on the inner surface is:
σ1=−q4πr21
- The surface charge density on the outer surface is:
σ2=Q+q4πr22
---
Part (b): Electric Field Intensity Inside the Cavity
1. Understanding Electric Field in Conductors:
- In electrostatics, the electric field inside a conductor in equilibrium is always zero. This is true regardless of the shape of the conductor.
2. Electric Field Inside the Cavity:
- Since the cavity is empty (with no charge), and the shell is a conductor, the electric field intensity inside the cavity is zero.
- This holds true even if the shell is not spherical and has an irregular shape.
3. Reasoning:
- The reason for this is that the charges on the conducting shell will redistribute themselves in such a way that they cancel any electric field within the conductor and the cavity.
- The net work done by the electric field in moving a test charge around a closed loop inside the cavity is zero, indicating that the electric field itself must be zero.
Summary for Part (b):
- The electric field intensity inside the cavity is zero, regardless of the shape of the shell, due to the properties of conductors in electrostatic equilibrium.
---
- We have a spherical conducting shell with inner radius r1 and outer radius r2.
- The shell has a total charge Q.
- A charge q is placed at the center of the shell.
- When charge q is placed at the center, it induces a charge of −q on the inner surface of the shell. This is because the conducting material will rearrange its charges to maintain electrostatic equilibrium.
- Therefore, the total charge on the inner surface, Qinner=−q.
- The surface charge density σ1 on the inner surface is given by:
σ1=QinnerArea of inner surface=−q4πr21
- The total charge on the shell is Q. Since −q is on the inner surface, the remaining charge on the outer surface must be:
Qouter=Q+q
- The surface charge density σ2 on the outer surface is given by:
σ2=QouterArea of outer surface=Q+q4πr22
- The surface charge density on the inner surface is:
σ1=−q4πr21
- The surface charge density on the outer surface is:
σ2=Q+q4πr22
- In electrostatics, the electric field inside a conductor in equilibrium is always zero. This is true regardless of the shape of the conductor.
- Since the cavity is empty (with no charge), and the shell is a conductor, the electric field intensity inside the cavity is zero.
- This holds true even if the shell is not spherical and has an irregular shape.
- The reason for this is that the charges on the conducting shell will redistribute themselves in such a way that they cancel any electric field within the conductor and the cavity.
- The net work done by the electric field in moving a test charge around a closed loop inside the cavity is zero, indicating that the electric field itself must be zero.
- The electric field intensity inside the cavity is zero, regardless of the shape of the shell, due to the properties of conductors in electrostatic equilibrium.
Topper's Solved these Questions
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SET -II
Explore 9 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SET -III
Explore 4 Videos
- XII BOARDSBook:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:XII BOARDSExercise:SET-III DELHI BOARD
Explore 10 Videos
- SAMPLE PAPER 2019Book:XII BOARD PREVIOUS YEAR PAPER ENGLISHChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
Similar Questions
A metal spherical shell has an inner radius R1 and outer radius R2. A charge Q is placed at the center of the spherical cavity. What will be surface charge density on (i) the inner surface, and (ii) the outer surface ?
View SolutionA metallic spherical shell has an inner radius R1 and outer radius R2. A charge is placed at the centre of the spherical cavity. The surface charge density on the inner surface is

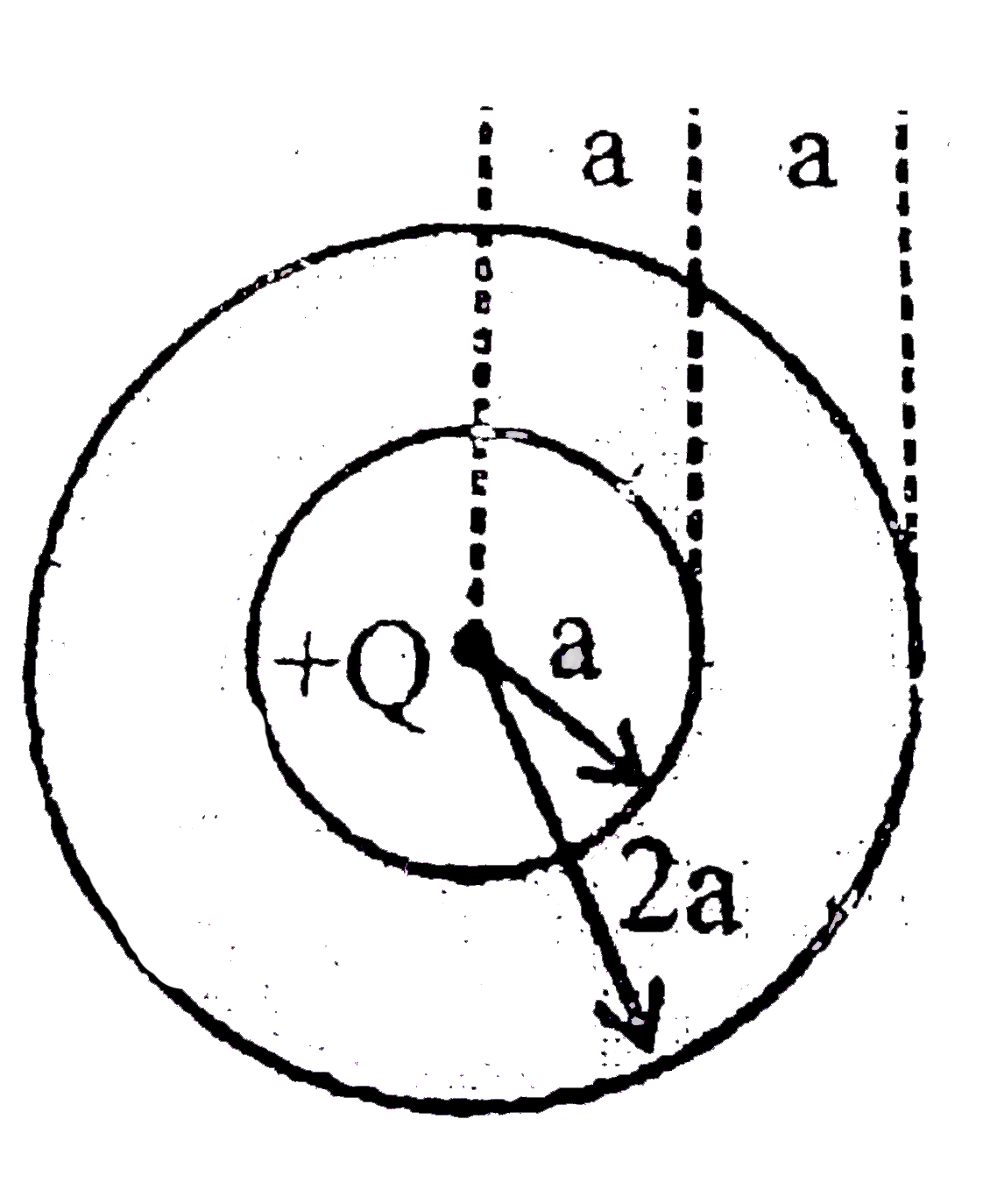
View SolutionA solid spherical conducting shell has inner radius a and outer radius 2a. At the center of the shell is located a point charge +Q. What must the excess charge of the shell be in order for the charge density on the inner and outer surface of the shell to be exactly equal?
View SolutionTwo concentric conducting shells A and B are of radii R and 2R. A charge +q is placed at the centre of the shells. Shell B is earthed and a charge q is given to shell A. Find the charge on outer surface of A and B.
View SolutionA thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R/2 from the centre of the shell is
View SolutionA thin spherical conducting shell of radius R has a charge q. Another charge Q is placed at the centre of the shell. The electrostatic potential at a point P a distance R2 from the centre of the shell is
View SolutionA conducting shell of radius R carries charge–Q. A point charge +Q is placed at the centre of shell. The electric field E varies with distance r (from the centre of the shell) as :
View SolutionA point charge +q is placed at the centre of a conducting spherical shell of inner radius a and outer radius b. Find the charge appearing on the inner and outer surface of the shell.
View SolutionA point charge Q is placed inside a conducting spherical shell of inner radius 3R and outer radius 5R at a distance R from the centre of the shell. The electric potential at the centre of the shell will be
View SolutionA ball with charge −50e is placed at the centre of a hollow spherical shell has a net charge of −50e. What is the charge on the shell's outer surface ?
View Solution