Question

How does an unpolarised light get polarised when passed through a polaroid ?
Two polaroids are set in crossed positions . A third polaroid is placed between the two making an angle $\theta$ with the pass axis of the first polaroid . Write the expression for the intensity of light transmitted from the second polaroid . In what orientations will the transmitted intensity be (i) minimum and (ii) maximum ?

Explanation

If an unpolarised light wave is incident on a polaroid then the light get linearly polarised with the electric vector oscillating along a direction perpendicular to the aligned molecules .
If the light from an ordinary source (like a sodium lamp) passes through a polaroid sheet `P_(1)`, it is observed that its intensity is reduced by half.Rotating `P_(1)` has no effect on the transmitted beam and transmitted intensity constant .
Let an identical piece of polaroid `P_(2)` be placed before `P_(1)` , the light from the lamp is reduced in intensity on passing through `P_(2)` alone . But rotating `P_(1)` has effect on the light coming from `P_(2)`.
In one position , the intensity transmitted by `P_(2)` followed by `P_(1)` is nearly zero . When turned by `90^(@)` from this position `P_(1)` transmits nearly the full intensity emerging from `P_(2)` as shown in fig.

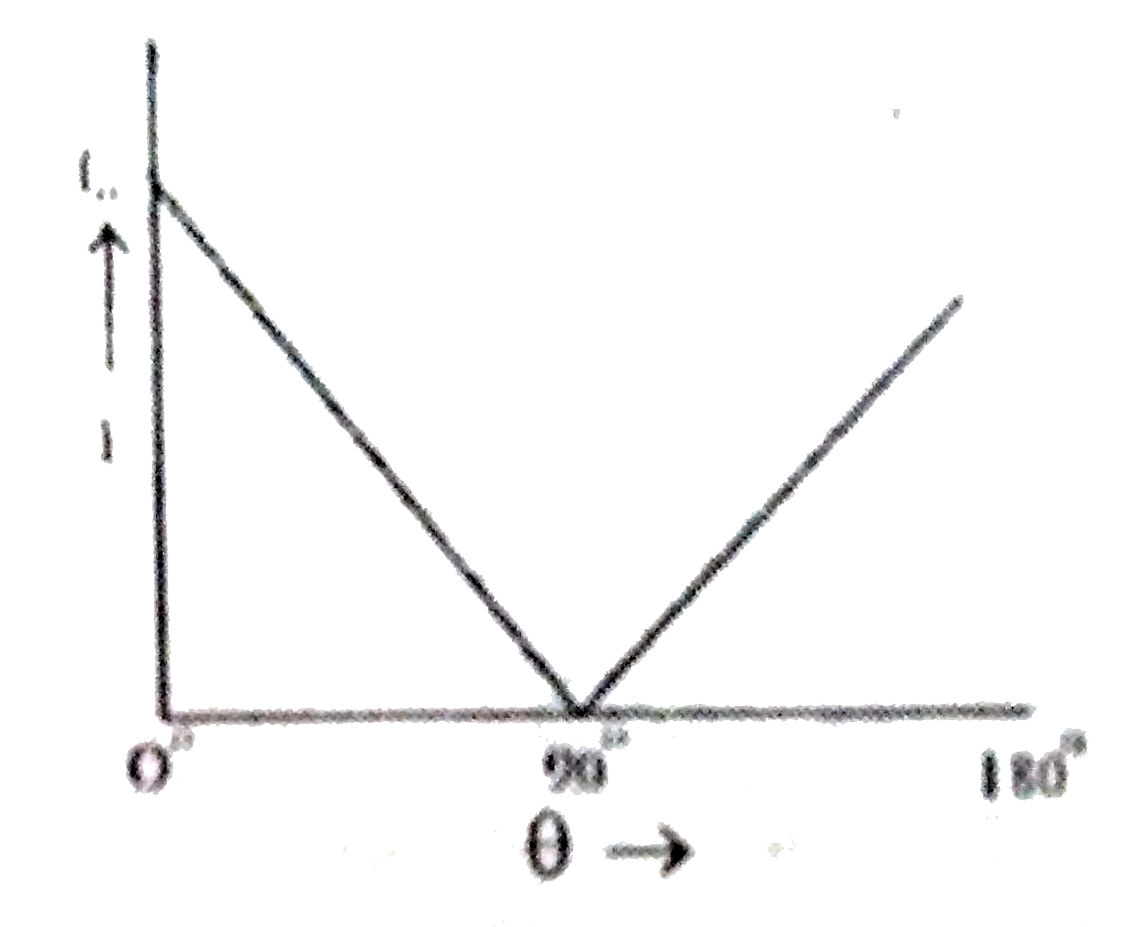
Graph : The graph between intensity of light and the angle between polariser and analyser is shown in the figure .
When the polaroid is rotated in the path of plane polarised light , its intensity will vary from maximum (When the vibrations of the plane polarised light are parallel to the axis of the polaroid ) to minimum (When the direction of vibrations becomes perpendicular to the axis of the crystal).
Let `I_(0)` be the intensity of polarised light after passing through first polariser `P_(1) ` . Then intensity of light after passing through second polariser `P_(2)` is given by `I = I_(0) cos^(2) theta`.