In the figure, a ladder of mass m is shown leaning against a wall. It is in static equilibrium making an angle θ with the horizontal floor. The coefficient of friction between the wall and the ladder is μ1 and that between the floor and the ladder is μ2. the normal reaction of the wall on the ladder is N1 and that of the floor is N2. if the ladder is about to slip. than
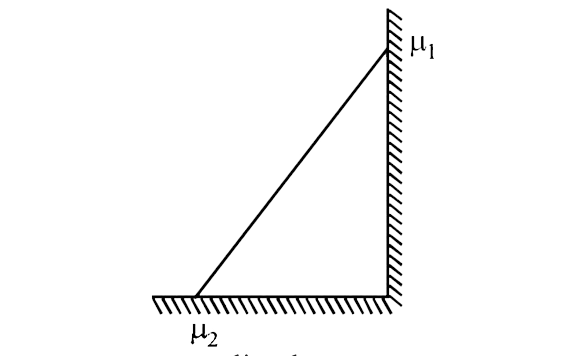
μ1=0,μ2≠0 and N2,tanθ=mg/2
μ1=0,μ2=0 and N1tanθ=mg/2
μ1≠0μ2≠0 and N2=mg1+μ1μ2
μ1=0μ2≠0 and N1tanθ=mg/2
The correct Answer is:C, D
Consider of translational equilibrium,
N1=μ2N2………..(i)

N2+μ1N1=Mg……..(ii)
Solving N2=mg1+μ1μ2,N2=μ1mg1+μ1μ2
Appling torque equation about corner (left) point on the florr
mgl2cosθ=N1lsinθ+μ1N1lcosθ
Solving tanθ=1−μ1μ22μ2
Topper's Solved these Questions
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (LINKED COMPREHENSIVE TYPE)
7 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (ASSERTION & REASON TYPE)
2 Videos
- ROTATIONAL MOTION BOOK - VMC MODULES ENGLISHCHAPTER - ROTATIONAL MOTION EXERCISE - JEE Advanced (Archive) (SINGLE OPTION CORRECT TYPE)
26 Videos
- REVISION TEST-2 JEEBOOK - VMC MODULES ENGLISHCHAPTER - REVISION TEST-2 JEEEXERCISE - PHYSICS
25 Videos
- SIMPLE HARMONIC MOTION BOOK - VMC MODULES ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - 7-previous year question
46 Videos
Similar Questions
The length of a uniform ladder of mass m is 1. It leans against a smooth wall making an angle θ with the horizontal. What should be the force of friction between the floor and ladder so that the ladder does not slip ?
View SolutionA block of mass m kg is pushed up against a wall by a force P that makes an angle 'θ' with the horizontal as shown in figure. The coefficient of static friction between the block and the wall is μ. The minimum value of P that allows the block to remain stationary is
View Solution
Knowledge Check
A ladder, metres long standing on a floor leans against a vertical wall . If the top of the ladder slides downwards at the rate of 10 cm/sec , then the rate at which the angel between the floor and the ladder is decreasing when the lower end of the ladder is 2 metres away from the wall is
A110 radian/secB120 radina/secC5 radian/secD10 radian/ sec
A ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
View SolutionA particle of mass m strikes a horizontal smooth floor with velocity u making an angle θ with the floor and rebound with velocity v making an angle θ with the floor. The coefficient of restitution between the particle and the floor is e. Then
View SolutionA ladder 13 m long rests against a vertical wall. If the foot of the ladder is 5 m from the foot of the wall, find the distance of the other end of the ladder from the ground.
View SolutionA ladder 13 m long rests against a vertical wall. If the foot of the ladder is 5 m from the foot of the wall, find the distance of the other end of the ladder from the ground.
View SolutionA ladder of mass 10kg is held at reat against a smooth wall on a rough ground as shown in figure. The normal reaction between the wall and the ladder is
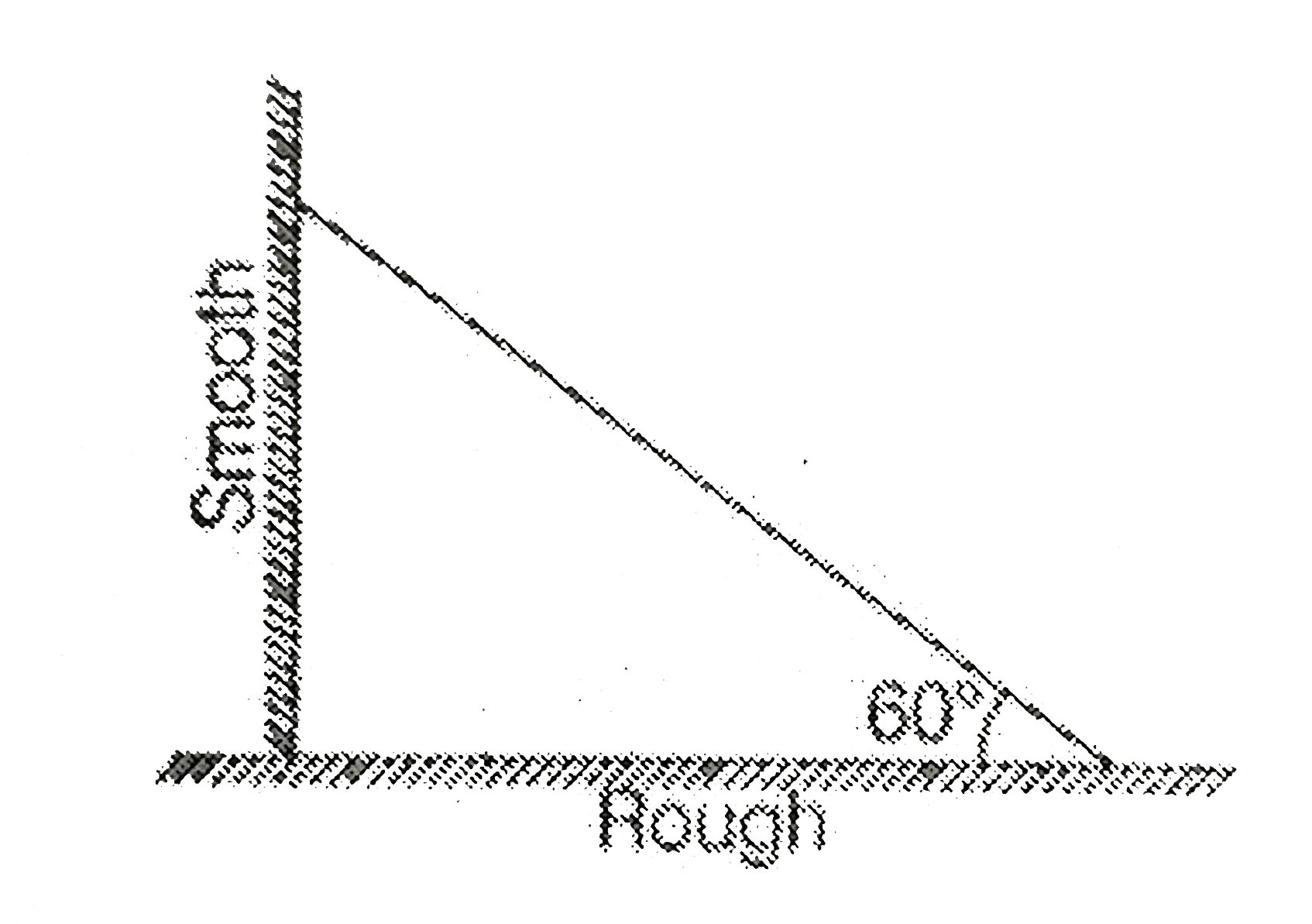
View SolutionA uniform ladder of mass 10 kg leans against a smooth vertical wall making an angle of 530 with it. The other end rests on a rough horizontal floor. Find the normal force and the frictional force that the floor exerts on the ladder
View SolutionA ladder 5m long rests against a vertical wall. If its top slides down at the rate of 10cm/sec, then, when the foot of the ladder is 4m away from the wall, the angle between the floor and the ladder is decreasing at the rate of
View Solution
