The length of a uniform ladder of mass m is 1. It leans against a smooth wall making an angle θ with the horizontal. What should be the force of friction between the floor and ladder so that the ladder does not slip ?
To solve the problem of finding the force of friction between the floor and the ladder so that the ladder does not slip, we can follow these steps:
1. Identify the Forces Acting on the Ladder:
- The ladder has a weight mg acting downwards at its center of mass (midpoint).
- There is a normal force N1 acting upwards at the base of the ladder (on the floor).
- There is a normal force N2 acting horizontally at the point where the ladder touches the wall.
- There is a frictional force f acting horizontally at the base of the ladder, opposing the tendency to slip.
2. Set Up the Equilibrium Conditions:
- For the ladder to be in equilibrium, the sum of vertical forces must equal zero, and the sum of horizontal forces must equal zero.
- The vertical forces give us the equation:
N1=mg
- The horizontal forces give us the equation:
f=N2
3. Taking Moments About Point O:
- To find the frictional force, we can take moments about point O (the point where the ladder touches the wall).
- The moment due to the weight of the ladder (acting at a distance of 12cosθ from point O) is:
Moment due to weight=mg⋅(12cosθ)
- The moment due to the normal force N1 (acting at a distance of 1sinθ from point O) is:
Moment due to N1=N1⋅(1sinθ)
4. Setting Up the Moment Equation:
- For the ladder to be in rotational equilibrium, the sum of moments about point O must be zero:
N1⋅(1sinθ)−mg⋅(12cosθ)=0
5. Solving for N1:
- Rearranging the moment equation gives:
N1sinθ=mg2cosθ
- Solving for N1:
N1=mg2⋅cosθsinθ=mg2cotθ
6. Finding the Friction Force:
- Since we established that f=N2 and N2=N1, we can conclude:
f=mg2cotθ
Final Answer:
The force of friction between the floor and the ladder so that the ladder does not slip is:
f=mg2cotθ
- The ladder has a weight mg acting downwards at its center of mass (midpoint).
- There is a normal force N1 acting upwards at the base of the ladder (on the floor).
- There is a normal force N2 acting horizontally at the point where the ladder touches the wall.
- There is a frictional force f acting horizontally at the base of the ladder, opposing the tendency to slip.
- For the ladder to be in equilibrium, the sum of vertical forces must equal zero, and the sum of horizontal forces must equal zero.
- The vertical forces give us the equation:
N1=mg
- The horizontal forces give us the equation:
f=N2
- To find the frictional force, we can take moments about point O (the point where the ladder touches the wall).
- The moment due to the weight of the ladder (acting at a distance of 12cosθ from point O) is:
Moment due to weight=mg⋅(12cosθ)
- The moment due to the normal force N1 (acting at a distance of 1sinθ from point O) is:
Moment due to N1=N1⋅(1sinθ)
- For the ladder to be in rotational equilibrium, the sum of moments about point O must be zero:
N1⋅(1sinθ)−mg⋅(12cosθ)=0
- Rearranging the moment equation gives:
N1sinθ=mg2cosθ
- Solving for N1:
N1=mg2⋅cosθsinθ=mg2cotθ
- Since we established that f=N2 and N2=N1, we can conclude:
f=mg2cotθ
The force of friction between the floor and the ladder so that the ladder does not slip is:
f=mg2cotθ
Topper's Solved these Questions
- FRICTIONBOOK - ICSECHAPTER - FRICTIONEXERCISE - Conceptual short answer questions with answer
9 Videos
- FRICTIONBOOK - ICSECHAPTER - FRICTIONEXERCISE - Long answer questions
2 Videos
- FRICTIONBOOK - ICSECHAPTER - FRICTIONEXERCISE - Selected problems
30 Videos
- DYNAMICSBOOK - ICSECHAPTER - DYNAMICSEXERCISE - SHORT ANSWER QUESTIONS WITH ANSWERS
12 Videos
- GRAVITATIONBOOK - ICSECHAPTER - GRAVITATIONEXERCISE - FROM THE HUBBLE TELESCOP
2 Videos
Similar Questions
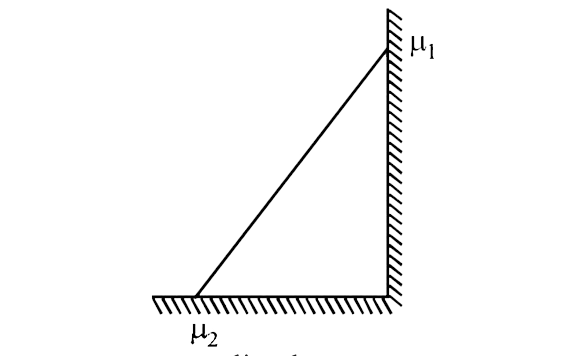
In the figure, a ladder of mass m is shown leaning against a wall. It is in static equilibrium making an angle θ with the horizontal floor. The coefficient of friction between the wall and the ladder is μ1 and that between the floor and the ladder is μ2. the normal reaction of the wall on the ladder is N1 and that of the floor is N2. if the ladder is about to slip. than
View SolutionA uniform ladder of mass 10 kg leans against a smooth vertical wall making an angle of 530 with it. The other end rests on a rough horizontal floor. Find the normal force and the frictional force that the floor exerts on the ladder
View Solution
Knowledge Check
A 4m long ladder weighing 25kg rests with its upper end against a smooth wall and lower end on rough ground.What should be the minimum coefficient of friction between the ground and the ladder for it to be inclined at 60∘ with the horizontal without slipping? (Takeg=10m/s2)
Aa. 0.19Bb. 0.29Cc. 0.39Dd. 0.49
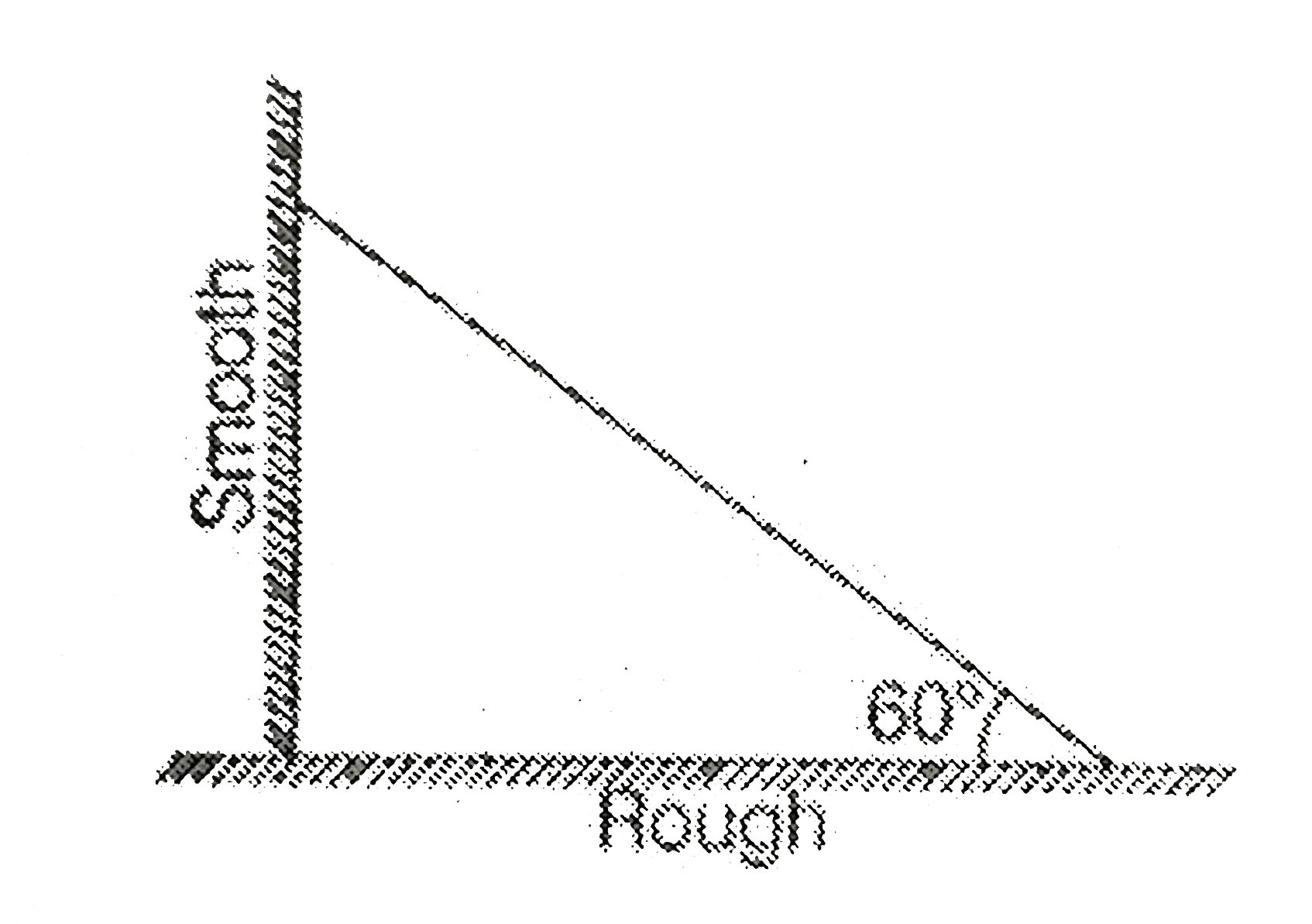
A ladder of mass 10kg is held at reat against a smooth wall on a rough ground as shown in figure. The normal reaction between the wall and the ladder is
View SolutionA ladder leaning against a wall makes an angle of 60° with the horizontal. If the foot of the ladder is 2.5 m away from the wall, find the length of the ladder.
View SolutionA uniform ladder of length L and mass m1 rests against a frictionless wall. The ladder makes an angle θ with the horizontal. (a) Find the horizontal and vertical forces the ground exerts on the base of the ladder when a firefighter of mass m2 is a distance x from the bottom. (b) If the ladder is just on the verge of slipping when the firefighter is a distance d from the bottom, what is the coefficient of static friction between ladder and ground?
View SolutionA4m long ladder weighing 25kg rests with its upper and against a smooth wall and lower and on rought ground.What should be the minimum coefficient of friction between the ground and ladder for it to be inclined at 60∘ with the horizontal without slipping? (Takeg=10m/s2)
View SolutionA ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
View SolutionA force P acts on a smooth block of mass m placed on a horizontal floor at an angle θ with with horizontal on the block

View SolutionA ladder of length 5m is placed against a smooth wall as shown in figure. The coefficient or friction is μ between ladder and ground. What is the minimum value of μ , If the ladder is not to slip?

View Solution
