A force of 3kg wt is sufficient to pull a block of 4kgwt over a flat surface. What is the angle of friction ?
The correct Answer is:36.86∘
To solve the problem, we need to find the angle of friction given that a force of 3 kg weight is sufficient to pull a block of 4 kg weight over a flat surface.
1. Understand the Forces Involved:
- The weight of the block (W) is given as 4 kg wt. This is equivalent to W=4g where g is the acceleration due to gravity (approximately 9.8m/s2).
- The pulling force (F) is given as 3 kg wt, which is F=3g.
2. Identify the Normal Force (N):
- On a flat surface, the normal force (N) acting on the block is equal to its weight:
N=W=4kg wt
3. Set Up the Equation for Friction:
- The frictional force (F_f) opposing the motion can be represented as:
Ff=μN
- Here, μ is the coefficient of friction.
4. Relate the Forces:
- Since the block is just about to move, the pulling force (F) is equal to the frictional force (F_f):
F=Ff
- Thus, we can write:
3g=μ(4g)
5. Cancel Out g:
- Since g appears on both sides, we can cancel it out:
3=4μ
6. Solve for the Coefficient of Friction (μ):
- Rearranging gives:
μ=34=0.75
7. Calculate the Angle of Friction (θ):
- The angle of friction (θ) is related to the coefficient of friction by the equation:
tanθ=μ
- Therefore:
tanθ=0.75
8. Find θ using the Inverse Tangent Function:
- To find the angle θ, we take the arctangent (inverse tangent):
θ=tan−1(0.75)
- Using a calculator, we find:
θ≈36.86∘
Final Answer:
The angle of friction is approximately 36.86∘.
---
- The weight of the block (W) is given as 4 kg wt. This is equivalent to W=4g where g is the acceleration due to gravity (approximately 9.8m/s2).
- The pulling force (F) is given as 3 kg wt, which is F=3g.
- On a flat surface, the normal force (N) acting on the block is equal to its weight:
N=W=4kg wt
- The frictional force (F_f) opposing the motion can be represented as:
Ff=μN
- Here, μ is the coefficient of friction.
- Since the block is just about to move, the pulling force (F) is equal to the frictional force (F_f):
F=Ff
- Thus, we can write:
3g=μ(4g)
- Since g appears on both sides, we can cancel it out:
3=4μ
- Rearranging gives:
μ=34=0.75
- The angle of friction (θ) is related to the coefficient of friction by the equation:
tanθ=μ
- Therefore:
tanθ=0.75
- To find the angle θ, we take the arctangent (inverse tangent):
θ=tan−1(0.75)
- Using a calculator, we find:
θ≈36.86∘
The angle of friction is approximately 36.86∘.
Topper's Solved these Questions
Similar Questions
A horizontal force of 490 N is required to slide a sledge weighing 600 kg f over a flat surface. Calculate the coefficient of friction.
View SolutionA force of 100 N is applied on a block of mass 3 kg as shown in figure. The coefficient of friction between the surface and the block is 14 .Then the friction force acting on the block is
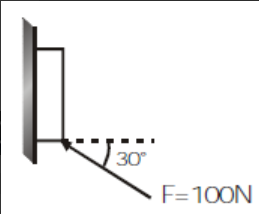
View SolutionA force of 98 N is just able to move a block of mass 20 kg on a rough horizontal surface. Calculate the coefficient of friction and the angle of friction (g=9.8ms−2).
View SolutionMinimum force is needed to move a block on rought surface, if θ= angle of friction.
Angle of friction and angle of repose are numerically same.
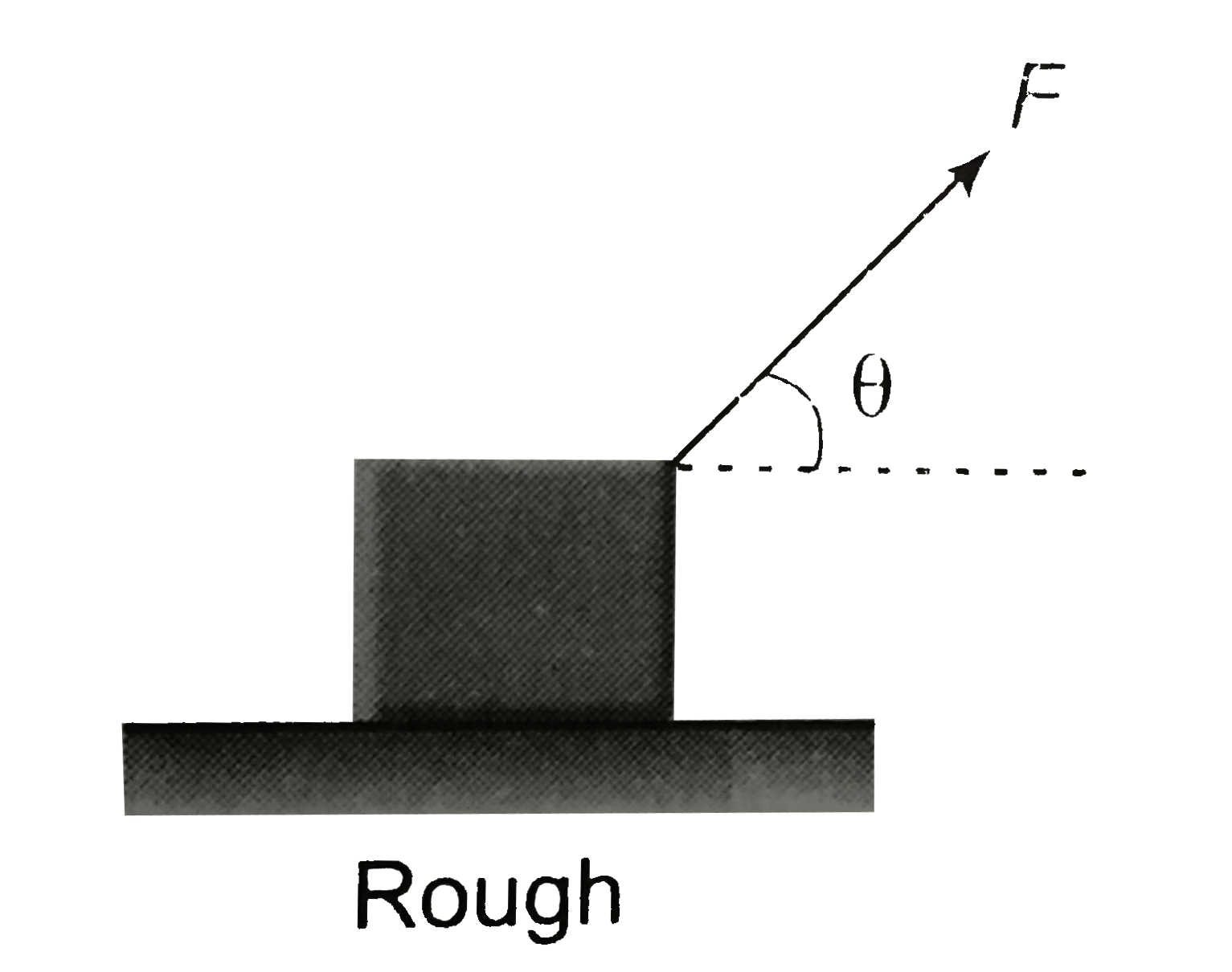
View SolutionA block of mass m is moving on a rough horizontal surface. μ is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View SolutionA force of 49 N just able to move a block of wood weighing 10 kg on a rough horizontal surface , then coefficient of friction is
View SolutionA force of 100N is applied on a block of mass 3kg as shown below. The coefficient of friction between wall and the block is 1/4. The friction force acting on the block is :
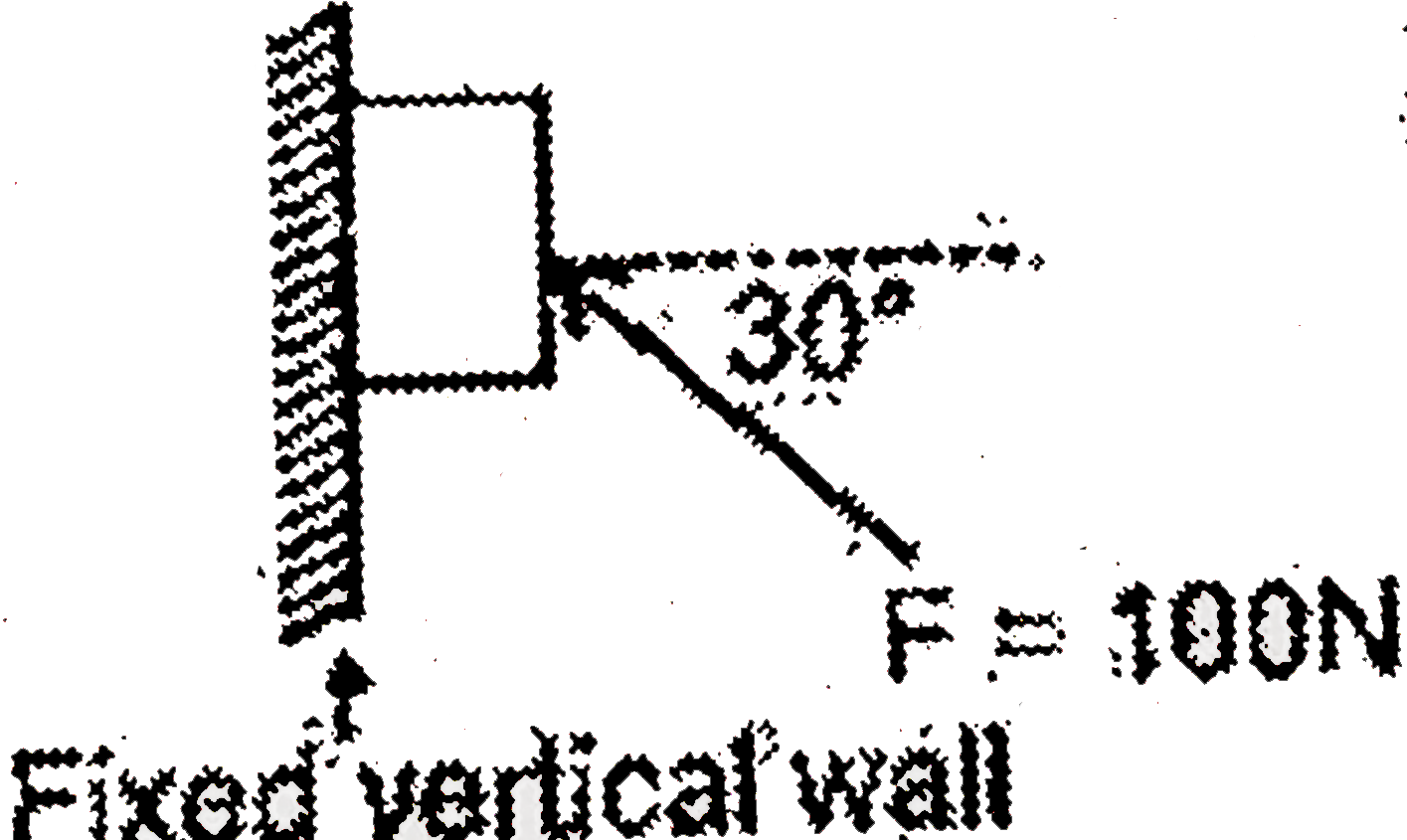
View SolutionA block weighing 20N rests on a horizontal surface . The coefficient of static friction between the block and surface is 0.4 and the coeffiecient of kinetic frictioni 0.20
a.How much is the friction force excerted on the block?
b. How much will the friction force be if a horizontal force of 5N is exerted on the block?
c. What is the minimum force that will start the block in motion ?
d. What is the minimum force that will keep the block in motion once is has been started ?
e. If the horizontal force is 10N what is the friction force?
View SolutionA wooden block of 100 kg is about to be pushed on a floor of coefficient of friction 0.4. What is the magnitude of the force of friction on the wooden block when it is just pushed ?
View SolutionA force of 750 N is applied to a block of mass 102 kg to prevent it from sliding on a plane with an inclination angle 30∘ with the horizontal. If the coefficients of static friction and kinetic friction between the block and the plane are 0.4 and 0.3 respectively, then the frictional force acting on the block is
View Solution