A ladder of length l and mass m is placed against a smooth vertical wall, but the ground is not smooth. Coefficient of friction between the ground and the ladder is μ. The angle θ at which the ladder will stay in equilibrium is
θ=tan−1(μ)
θ=tan−1(2μ)
θ=tan−1(μ2)
none of these
The correct Answer is:D
To solve the problem of determining the angle θ at which a ladder of length l and mass m stays in equilibrium against a smooth vertical wall, we will follow these steps:
Step 1: Draw the Free Body Diagram (FBD)
1. Draw the ladder leaning against the wall, making an angle θ with the ground.
2. Identify the forces acting on the ladder:
- Weight mg acting downward at the center of the ladder.
- Normal force N2 acting upward at the base of the ladder.
- Normal force N1 exerted by the wall acting horizontally towards the ladder.
- Friction force f acting horizontally at the base of the ladder, opposing the motion.
Step 2: Apply Force Balance in Horizontal Direction
- The horizontal forces must balance:
f=N1
- The friction force can also be expressed in terms of the normal force at the base:
f=μN2
- Therefore, we can write:
N1=μN2(Equation 1)
Step 3: Apply Force Balance in Vertical Direction
- The vertical forces must balance:
N2=mg(Equation 2)
Step 4: Substitute Equation 2 into Equation 1
- From Equation 2, substitute N2 into Equation 1:
N1=μmg(Equation 3)
Step 5: Apply Torque Balance
- To find the angle θ, we need to balance the torques about the base of the ladder (point O):
- The torque due to the weight mg acts at a distance of l2cosθ (perpendicular distance).
- The torque due to the normal force N1 acts at a distance of lsinθ (perpendicular distance).
- Setting the torques equal:
mg⋅(l2cosθ)=N1⋅(lsinθ)
Step 6: Simplify the Torque Equation
- Cancel l from both sides:
mg⋅(12cosθ)=N1sinθ
- Substitute N1 from Equation 3:
mg⋅(12cosθ)=μmgsinθ
- Cancel mg from both sides:
12cosθ=μsinθ
Step 7: Rearrange to Find θ
- Rearranging gives:
tanθ=12μ
- Therefore, the angle θ can be expressed as:
θ=tan−1(12μ)
Final Answer
The angle θ at which the ladder will stay in equilibrium is:
θ=tan−1(12μ)
1. Draw the ladder leaning against the wall, making an angle θ with the ground.
2. Identify the forces acting on the ladder:
- Weight mg acting downward at the center of the ladder.
- Normal force N2 acting upward at the base of the ladder.
- Normal force N1 exerted by the wall acting horizontally towards the ladder.
- Friction force f acting horizontally at the base of the ladder, opposing the motion.
- The horizontal forces must balance:
f=N1
- The friction force can also be expressed in terms of the normal force at the base:
f=μN2
- Therefore, we can write:
N1=μN2(Equation 1)
- The vertical forces must balance:
N2=mg(Equation 2)
- From Equation 2, substitute N2 into Equation 1:
N1=μmg(Equation 3)
- To find the angle θ, we need to balance the torques about the base of the ladder (point O):
- The torque due to the weight mg acts at a distance of l2cosθ (perpendicular distance).
- The torque due to the normal force N1 acts at a distance of lsinθ (perpendicular distance).
- Setting the torques equal:
mg⋅(l2cosθ)=N1⋅(lsinθ)
- Cancel l from both sides:
mg⋅(12cosθ)=N1sinθ
- Substitute N1 from Equation 3:
mg⋅(12cosθ)=μmgsinθ
- Cancel mg from both sides:
12cosθ=μsinθ
- Rearranging gives:
tanθ=12μ
- Therefore, the angle θ can be expressed as:
θ=tan−1(12μ)
The angle θ at which the ladder will stay in equilibrium is:
θ=tan−1(12μ)
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Multiple Correct9videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Linked Comprehension28videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Subjective13videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger2videos
RIGID BODY DYNAMICS 2
Similar Questions
A uniform ladder of length 3.25 m and weight 250 N is placed against a smooth vertical wall with its lower end 1.25 m from the wall. If coefficient of friction between the ladder and the floor is 0.3, then, find the frictional force acting on the ladder at the point of contact between ladder and floor.
View SolutionA ladder of length 5m is placed against a smooth wall as shown in figure. The coefficient or friction is μ between ladder and ground. What is the minimum value of μ , If the ladder is not to slip?
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
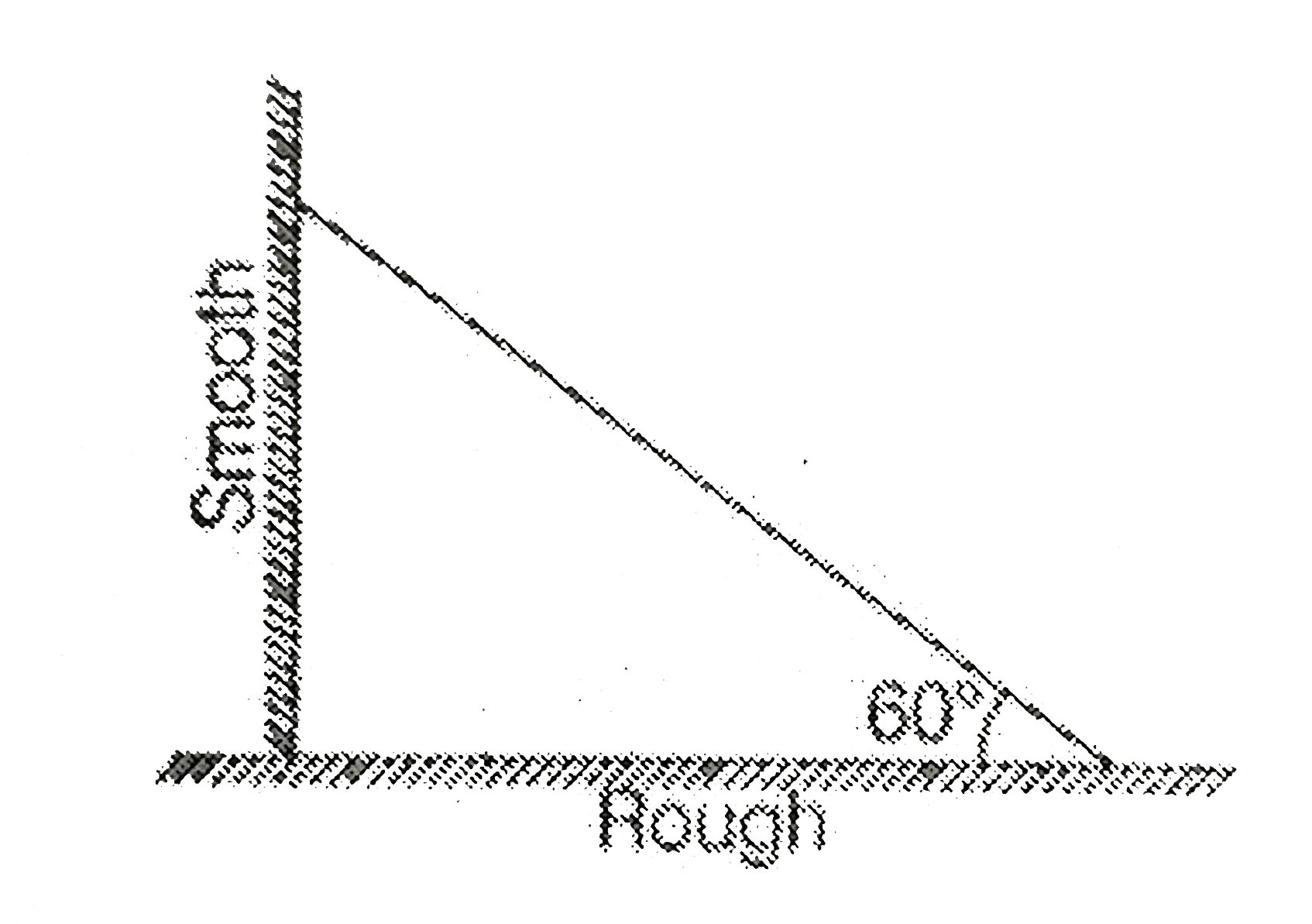
A 4m long ladder weighing 25kg rests with its upper end against a smooth wall and lower end on rough ground.What should be the minimum coefficient of friction between the ground and the ladder for it to be inclined at 60∘ with the horizontal without slipping? (Takeg=10m/s2)
Aa. 0.19Bb. 0.29Cc. 0.39Dd. 0.49
A ladder of length 5m is placed against a smooth wall as shown in figure. The coefficient or friction is μ between ladder and ground. What is the minimum value of μ , If the ladder is not to slip?
 View Solution
View SolutionA ladder of mass 10kg is held at reat against a smooth wall on a rough ground as shown in figure. The normal reaction between the wall and the ladder is
 View Solution
View SolutionIf a ladder is not in balance against a smooth vertical wall, then it can be made in balance by :-
View SolutionA4m long ladder weighing 25kg rests with its upper and against a smooth wall and lower and on rought ground.What should be the minimum coefficient of friction between the ground and ladder for it to be inclined at 60∘ with the horizontal without slipping? (Takeg=10m/s2)
View SolutionIn the figure, a ladder of mass m is shown leaning against a wall. It is in static equilibrium making an angle θ with the horizontal floor. The coefficient of friction between the wall and the ladder is μ1 and that between the floor and the ladder is μ2. the normal reaction of the wall on the ladder is N1 and that of the floor is N2. if the ladder is about to slip. than
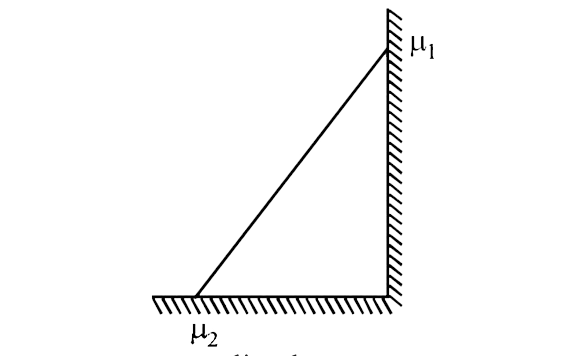 View Solution
View SolutionThe length of a uniform ladder of mass m is 1. It leans against a smooth wall making an angle θ with the horizontal. What should be the force of friction between the floor and ladder so that the ladder does not slip ?
View SolutionA ladder of length 5m is placed against a smooth wall as shown in figure. The coefficient or friction is μ between ladder and ground. What is the minimum value of μ , If the ladder is not to slip?
 View Solution
View Solution
