A block of mass m kg is pushed up against a wall by a force P that makes an angle 'θ' with the horizontal as shown in figure. The coefficient of static friction between the block and the wall is μ. The minimum value of P that allows the block to remain stationary is
To solve the problem of finding the minimum value of force P that allows the block to remain stationary against the wall, we can follow these steps:
Step 1: Identify Forces Acting on the Block
The forces acting on the block are:
- The weight of the block, mg (acting downwards).
- The applied force P at an angle θ with the horizontal, which can be resolved into two components:
- Horizontal component: Pcosθ (acting towards the wall).
- Vertical component: Psinθ (acting upwards).
- The normal force N exerted by the wall on the block (acting horizontally).
- The frictional force f acting upwards, which is given by f=μN, where μ is the coefficient of static friction.
Step 2: Apply Newton's Second Law in the Horizontal Direction
In the horizontal direction, the block is in equilibrium, so the horizontal component of the applied force must equal the normal force:
N=Pcosθ
Step 3: Apply Newton's Second Law in the Vertical Direction
In the vertical direction, the forces must also balance. The upward forces (frictional force and vertical component of P) must equal the downward force (weight of the block):
f+Psinθ=mg
Substituting the expression for the frictional force:
μN+Psinθ=mg
Now substituting N=Pcosθ:
μ(Pcosθ)+Psinθ=mg
Step 4: Rearranging the Equation
Now we can rearrange the equation to solve for P:
μPcosθ+Psinθ=mg
Factoring out P:
P(μcosθ+sinθ)=mg
Step 5: Solve for P
Now, we can solve for P:
P=mgμcosθ+sinθ
Final Answer
The minimum value of the force P that allows the block to remain stationary is:
P=mgμcosθ+sinθ
---
The forces acting on the block are:
- The weight of the block, mg (acting downwards).
- The applied force P at an angle θ with the horizontal, which can be resolved into two components:
- Horizontal component: Pcosθ (acting towards the wall).
- Vertical component: Psinθ (acting upwards).
- The normal force N exerted by the wall on the block (acting horizontally).
- The frictional force f acting upwards, which is given by f=μN, where μ is the coefficient of static friction.
In the horizontal direction, the block is in equilibrium, so the horizontal component of the applied force must equal the normal force:
N=Pcosθ
In the vertical direction, the forces must also balance. The upward forces (frictional force and vertical component of P) must equal the downward force (weight of the block):
f+Psinθ=mg
Substituting the expression for the frictional force:
μN+Psinθ=mg
Now substituting N=Pcosθ:
μ(Pcosθ)+Psinθ=mg
Now we can rearrange the equation to solve for P:
μPcosθ+Psinθ=mg
Factoring out P:
P(μcosθ+sinθ)=mg
Now, we can solve for P:
P=mgμcosθ+sinθ
The minimum value of the force P that allows the block to remain stationary is:
P=mgμcosθ+sinθ
Topper's Solved these Questions
- LAWS OF MOTIONBook:AAKASH SERIESChapter:LAWS OF MOTIONExercise:EXERCISE - I
Explore 157 Videos
- LAWS OF MOTIONBook:AAKASH SERIESChapter:LAWS OF MOTIONExercise:EXERCISE - II
Explore 136 Videos
- LAWS OF MOTIONBook:AAKASH SERIESChapter:LAWS OF MOTIONExercise:Problem
Explore 162 Videos
- GEOMETRICAL OPTICSBook:AAKASH SERIESChapter:GEOMETRICAL OPTICSExercise:ADDITIONAL PRACTICE EXERCISE (LEVEL-II PRACTICE SHEET (ADVANCED) INTEGER TYPE QUESTIONS)
Explore 10 Videos
- LOGIC GATESBook:AAKASH SERIESChapter:LOGIC GATESExercise:Exercise (Very Short Answer)
Explore 10 Videos
Similar Questions
A block of mass m slides down an inclined plane which makes an angle θ with the horizontal. The coefficient of friction between the block and the plane is μ. The force exerted by the block on the plane is
View SolutionA block of mass 5kg is (i) pushed in case (A) and (ii) pulled in case (B), by a force F=20N, making an angle of 30∘ with the horizontal, as shown in the figures. The coefficient of friction between the block and floor is μ=0.2. The difference between the accelerattion of the block, in case (B) and case (A) will be : (g=10ms−2).
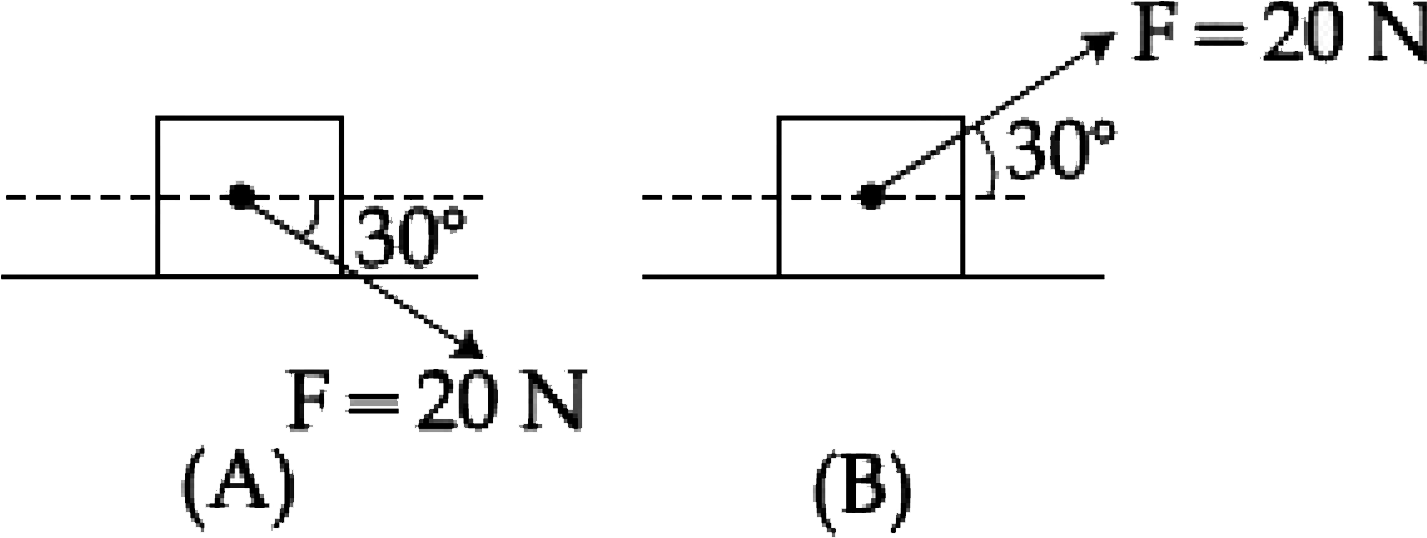 .
.
View Solution
Knowledge Check
A block of mass 3 kg rests on a rough inclined plane making an angle of 30∘. With the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A9.8 NB0.7×9.8×√3 NC9.8×√3 ND0.7×9.8 NA block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
A10.3NB23.8NC11.9ND6.3N
A block of mass 2kg rests on a rough inclined plane making an angle of 30∘ with the horizontal. The coefficient of static friction between the block and the plane is 0.7. The frictional force on the block is
View SolutionA cart of mass M has a block of mass m in contact with it as shown in the figure-2.133. The coefficient of friction between the block and the cart is μ. What is the minimum acceleration of the cart so that the block m does not fall ?

View SolutionA block of mass m is placed on a rough plane inclined at an angle θ with the horizontal. The coefficient of friction between the block and inclined plane is μ . Ifθ<tan−1(μ) , then net contact force exerted by the plane on the block is :
View SolutionA block slides down an inclined plane that makes an angle θ with the horizontal. If the coefficient of kinetic friction is μ, find the acceleration of the block.
View SolutionA block of mass m is pressed against a wall by a spring as shown in the figure. The spring has natural length , l(l>L), and the coefficient of friction between the block and the wall is μ . The minimum spring constant K necessary for equilibrium is:
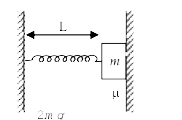
View SolutionA block of mass 1 kg is pressed against a wall by applying a horizontal force of 10N on the block. If the coefficient of friction between the block and the wall is 0.5, magnitude of the frictional force acting on the block is
View Solution