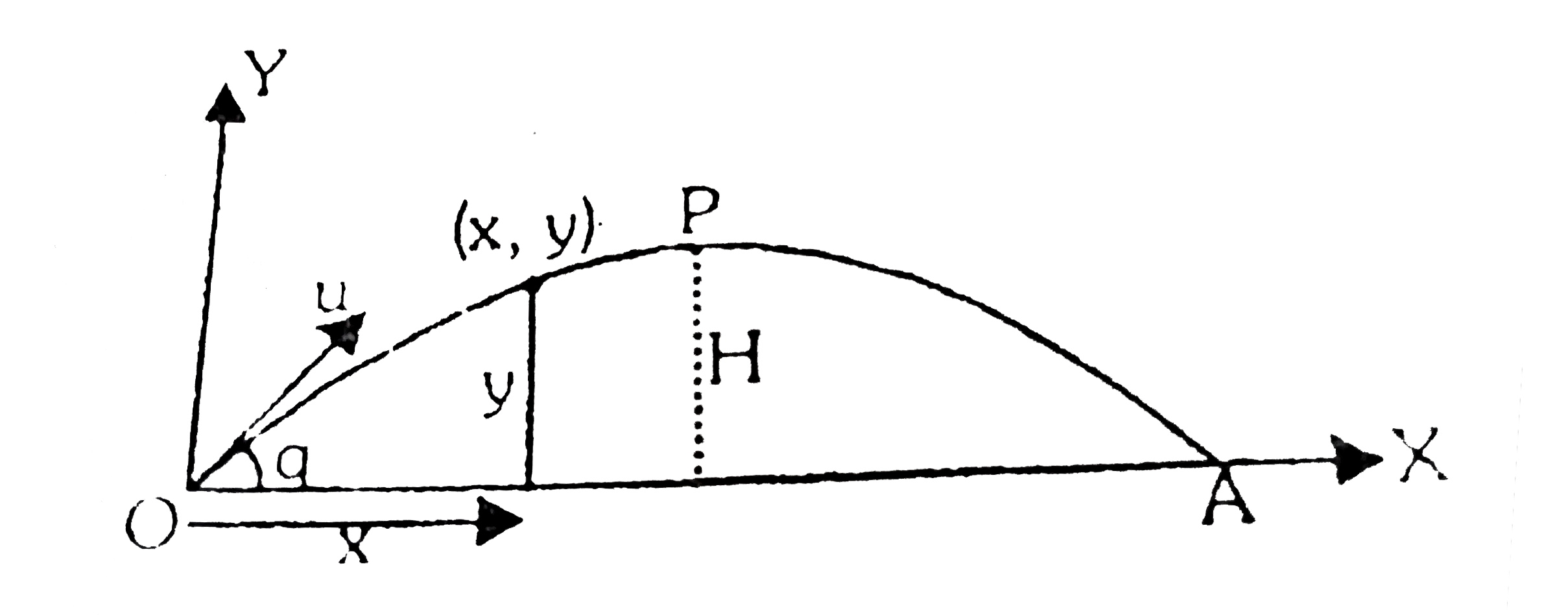The trajectory of a projectile in a vertical plane is y=ax−bx2, where aandb are constant and xandy are, respectively, horizontal and vertical distances of the projectile from the point of projection. The maximum height attained by the particle and the angle of projectile from the horizontal are.
The correct Answer is:ucos(α+θ)cosθ
To solve the problem of finding the maximum height attained by the projectile and the angle of projection from the horizontal given the trajectory equation y=ax−bx2, we can follow these steps:
Step 1: Identify the trajectory equation
The given equation of the trajectory is:
y=ax−bx2
where a and b are constants.
Step 2: Find the angle of projection
The angle of projection θ can be determined by comparing the given trajectory equation with the standard form of the projectile motion equation.
From the standard trajectory equation:
y=xtanθ−g2u2cos2θx2
we can identify that:
tanθ=a
Thus, the angle of projection θ is given by:
θ=tan−1(a)
Step 3: Find the maximum height
To find the maximum height, we need to find the maximum value of y. This can be done by differentiating the equation with respect to x and setting the derivative equal to zero.
1. Differentiate y with respect to x:
dydx=a−2bx
2. Set the derivative equal to zero to find the critical points:
a−2bx=0
Solving for x gives:
x=a2b
3. Substitute x back into the original equation to find y (the maximum height):
y=a(a2b)−b(a2b)2
Simplifying this:
y=a22b−b(a24b2)
y=a22b−a24b
y=2a24b−a24b=a24b
Thus, the maximum height Hmax is:
Hmax=a24b
Final Results
- The angle of projection θ is:
θ=tan−1(a)
- The maximum height attained by the projectile is:
Hmax=a24b
The given equation of the trajectory is:
y=ax−bx2
where a and b are constants.
The angle of projection θ can be determined by comparing the given trajectory equation with the standard form of the projectile motion equation.
y=xtanθ−g2u2cos2θx2
we can identify that:
tanθ=a
Thus, the angle of projection θ is given by:
θ=tan−1(a)
To find the maximum height, we need to find the maximum value of y. This can be done by differentiating the equation with respect to x and setting the derivative equal to zero.
dydx=a−2bx
a−2bx=0
Solving for x gives:
x=a2b
y=a(a2b)−b(a2b)2
Simplifying this:
y=a22b−b(a24b2)
y=a22b−a24b
y=2a24b−a24b=a24b
Thus, the maximum height Hmax is:
Hmax=a24b
- The angle of projection θ is:
θ=tan−1(a)
- The maximum height attained by the projectile is:
Hmax=a24b
Topper's Solved these Questions
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - MCQ
2 Videos
- Motion in Two DimensionsBOOK - VMC MODULES ENGLISHCHAPTER - Motion in Two DimensionsEXERCISE - JEE Main (Archive)
19 Videos
- Motion in Straight Line BOOK - VMC MODULES ENGLISHCHAPTER - Motion in Straight Line EXERCISE - IN-CHAPTER EXERCISE-J
10 Videos
- MOVING CHARGES & MAGNETISM BOOK - VMC MODULES ENGLISHCHAPTER - MOVING CHARGES & MAGNETISM EXERCISE - IN-CHAPTER EXERCISE-K
10 Videos
Similar Questions
Knowledge Check
If R and H represent horizontal range and maximum height of the projectile, then the angle of projection with the horizontal is
Atan−1(HR)Btan−1(2HR)Ctan−1(4HR)Dtan−1(4HH)The horizontal range of projectile is 4√3 times the maximum height achieved by it, then the angle of projection is
A30∘B45∘C60∘D90∘
The trajectory of a projectile in a vertical plane is y=√3x−2x2. [g=10m/s2]

Angle of projection θ is :-
View SolutionA projectile is projected from ground such that the maximum height attained by, it is equal to half the horizontal range.The angle of projection with horizontal would be
View SolutionThe horizontal range and the maximum height of a projectile are equal. The angle of projection of the projectile is
View SolutionThe trajectory of a projectile in a vertical plane is y=√3x−2x2. [g=10m/s2]

Maximum height H is :-
View SolutionThe trajectory of a projectile in a vertical plane is y=√3x−2x2. [g=10m/s2]

Time of flight of the projectile is :-
View SolutionThe horizontal and vertical displacements x and y of a projectile at a given time t are given by x=6tandy=8t−5t2 meter.The range of the projectile in metre is:
View Solution