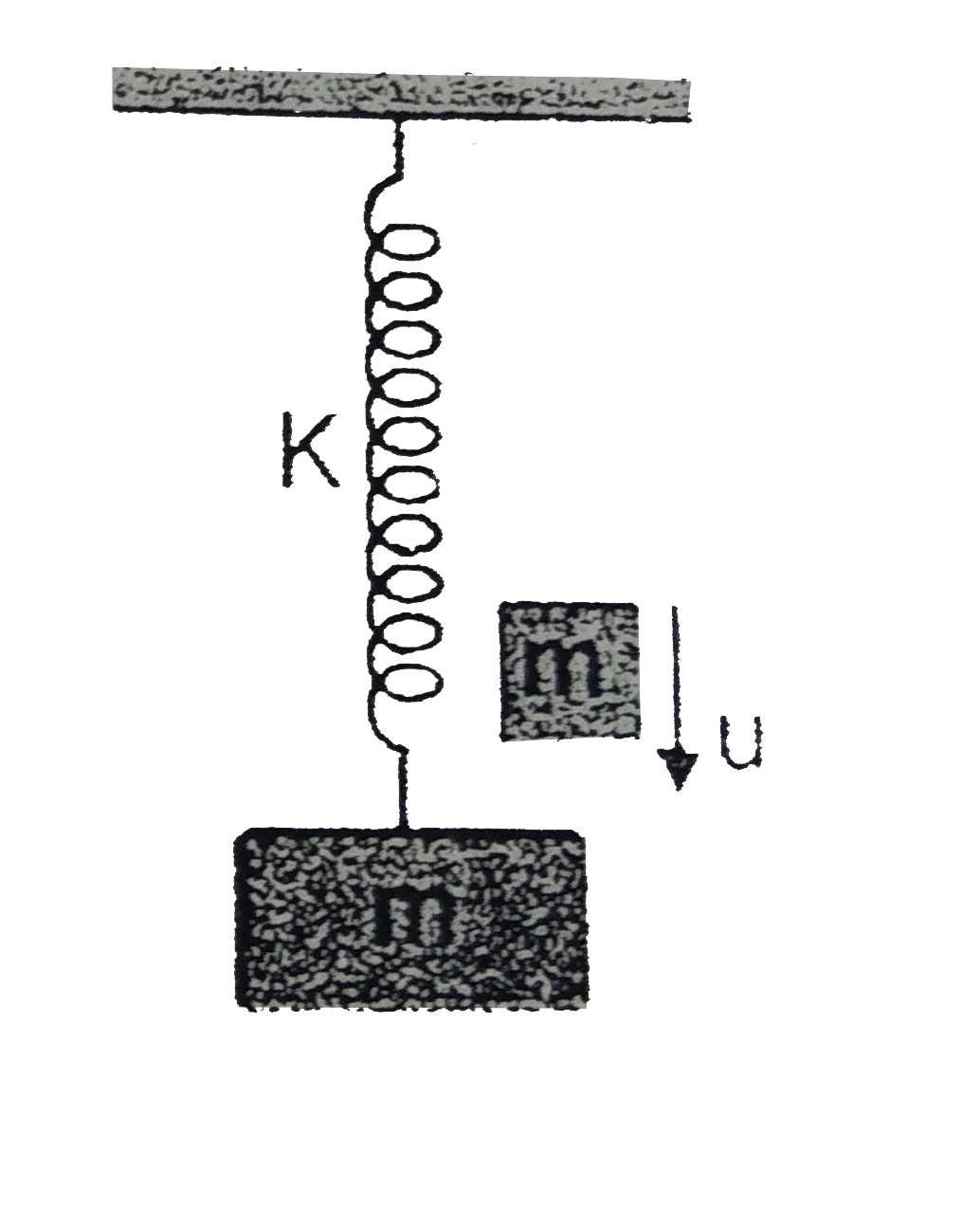
Figure shown a block P of mass m resting on a smooth horizontal ground, attached to one and a spring of force constant k in netural length. If another block od same mass and moving with a velocity u towards right is placed on the block on which stick to it due to friction ,find the time it will take to reach its exterme position. Also find the amplitude of oscillation of the combined mass 2m.

When second mass stricks to the lower mass , due to such an inelastic collision, the velocity of combined block is reduced by half , that is u/2, to conserve momentum . Now at mean position the velocity of block can be written as
u2=Aω (if A is amplitude of oscillation)
or u2=A√k2m
[Here for combined block new angular frequency of SHM is ω√k/2m]
or A=u2√2mk=u√m2k
As oscillation starts from mean position in reaching its extreme position , partical has to cover a phase of π/2 radians, thus time taken by the partical to reach its exterme position is l=π/2ω=π2√k2m
Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Subjective
21 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single Correct
107 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Exercise 4.1
23 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
Similar Questions
Consider a block of mass 'm' attached to a spring of spring constant 'K' as shown. Now a block of same mass 'm' moving with velocity 'u' strikes the other block and stick to it. Both blocks collectively start performing SHM.

The amplitude (A) of their SHM is
View SolutionFigure shown a block P of mass m resting on a smooth horizontal surface, attached to a spring of force constant k which is rigidly fixed on the wall on left side, shown in the figure . At a distance l to the right of the block there is a rigid wall. If block is pushed towards left so that spring is compressed by a distance 5l/3 and when released, if will starts its oscillations. If collision of block with the wall is considered to be perfectly elastic. Find the time period of oscillation of the block.

View SolutionConsider a block of mass 'm' attached to a spring of spring constant 'K' as shown. Now a block of same mass 'm' moving with velocity 'u' strikes the other block and stick to it. Both blocks collectively start performing SHM.
Choose the correct option(s) :
View SolutionA block of mass m moving with velocity v0 on a smooth horizontal surface hits the spring of constant k as shown. The maximum compression in spring is

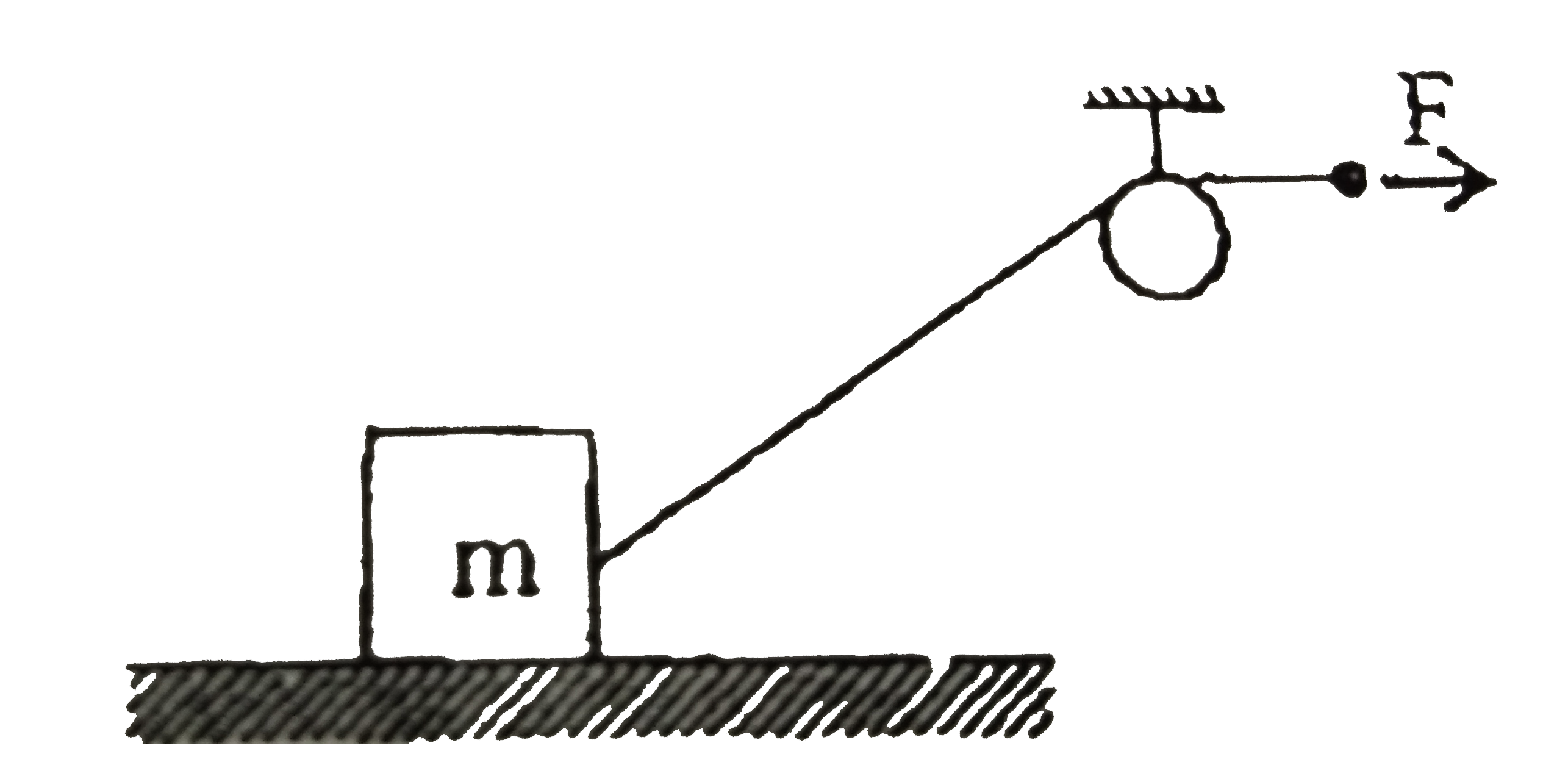
View SolutionA block of mass m is placed on a rough horizontal floor and it is pulled by a ideal string by a constant force F as shown. As the block moves towards right the friction force on block
View SolutionA bullet of mass m embeds itself in a block of mass M resting on a smooth horizontal surface, attached to a spring of force constant k. If the initial speed of the bullet is v0 along horizontal, find (a) the maximum compression of the spring and (b) the time for the bullet - block system to come to rest.
View SolutionA block of mass m is connected to a spring of force constant k. Initially the block is at rest and the spring has natural length. A constant force F is applied hrizontally towards right. The maximum speed of the block will be (there is no friction between block and the surface)

View SolutionA block of mass m1 resting on a frictionless horizontal surface is connected to a spring of spring constant k that is anchored in a near by wall. A block of mass m2 is placed on the top of the first block. The coefficient of static friction between the two blocks is μ Assuming that the two blocks move together as a unit, find the period of oscillation of the system and also find maximum oscillation amplitude that permits the two bodies to move as a unit.
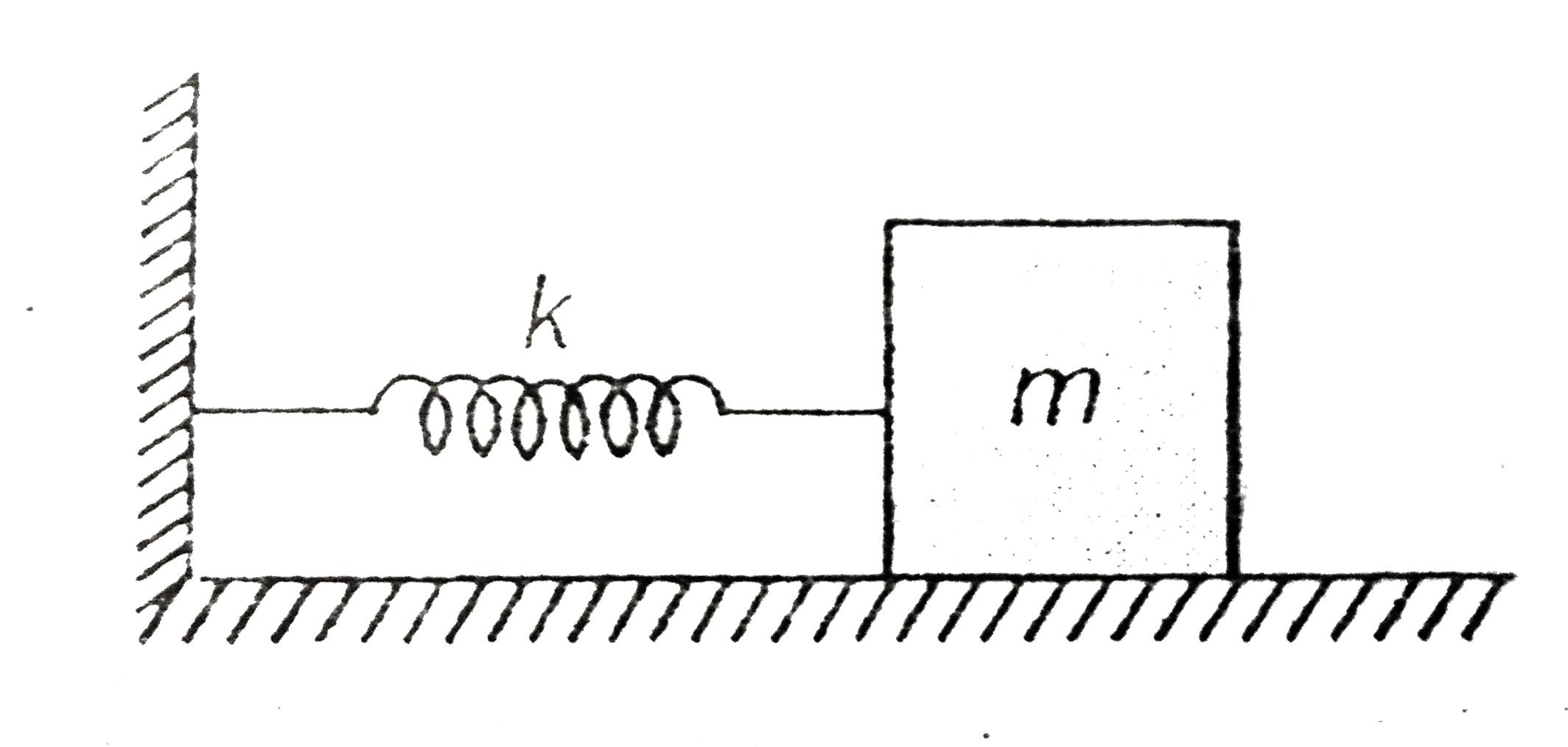
View SolutionFigure shows a block of mass 'm' resting on a smooth horizontal surface. It is connected to a rigid wall by a massless spring of stiffness 'k'. The spring is in its natural length. A constant horizontal force F starts acting on the block towards right. Find (i) speed of the block as it moves throught a distance x, (ii) speed when the block is in equlibrium and (iii) maximum extension produced in the speing.
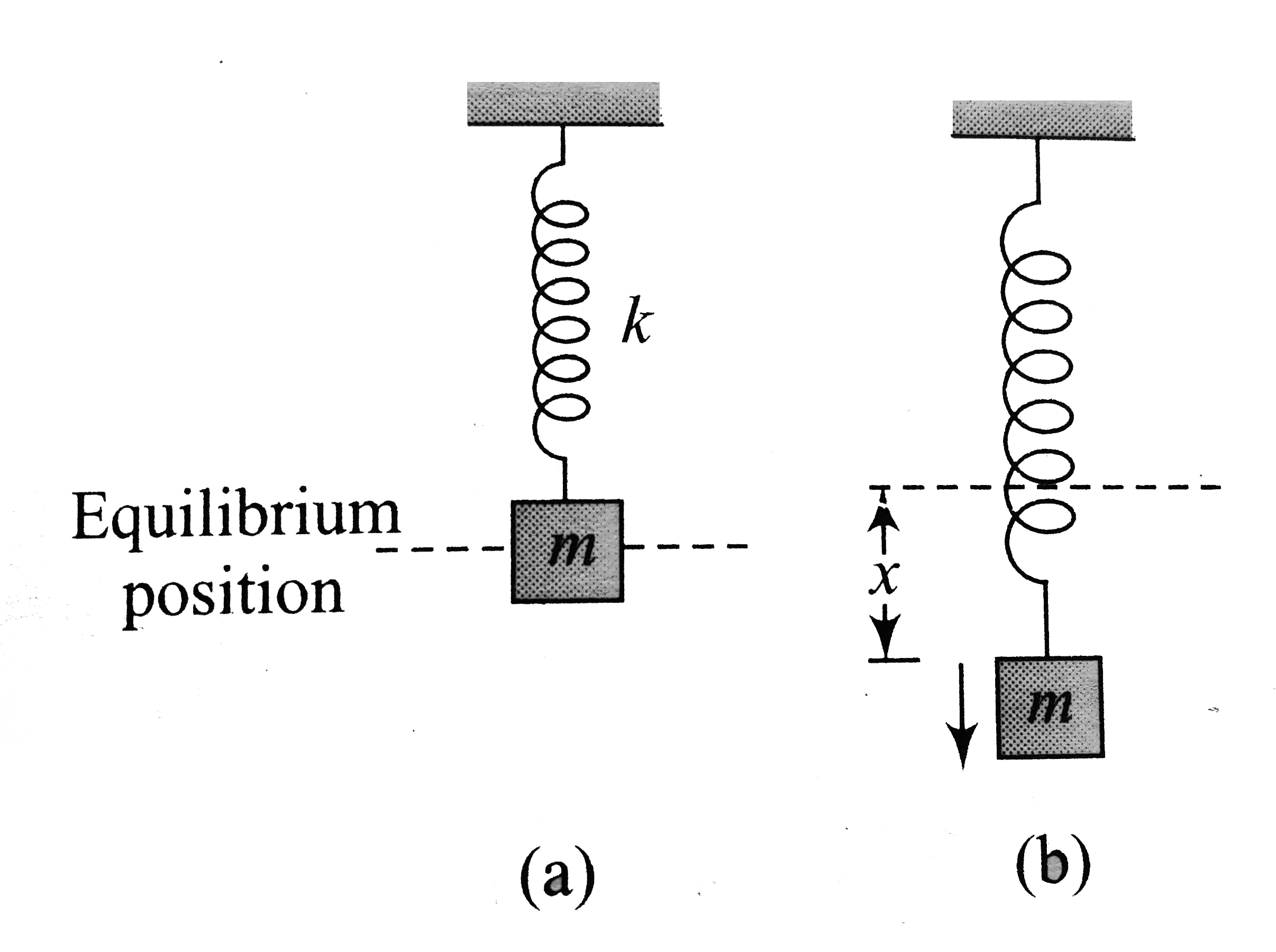
View SolutionFigure shown a spring block system hanging in equilibrium. The block of system is pulled down by the distance x and imparted a velocity v in downward direction as shown in figure. Find the time it will take to reach its mean position. Also find the maximum distance to which if will move before returning back towards mean position.
View Solution
