Figure shown a spring block system hanging in equilibrium. The block of system is pulled down by the distance x and imparted a velocity v in downward direction as shown in figure. Find the time it will take to reach its mean position. Also find the maximum distance to which if will move before returning back towards mean position.
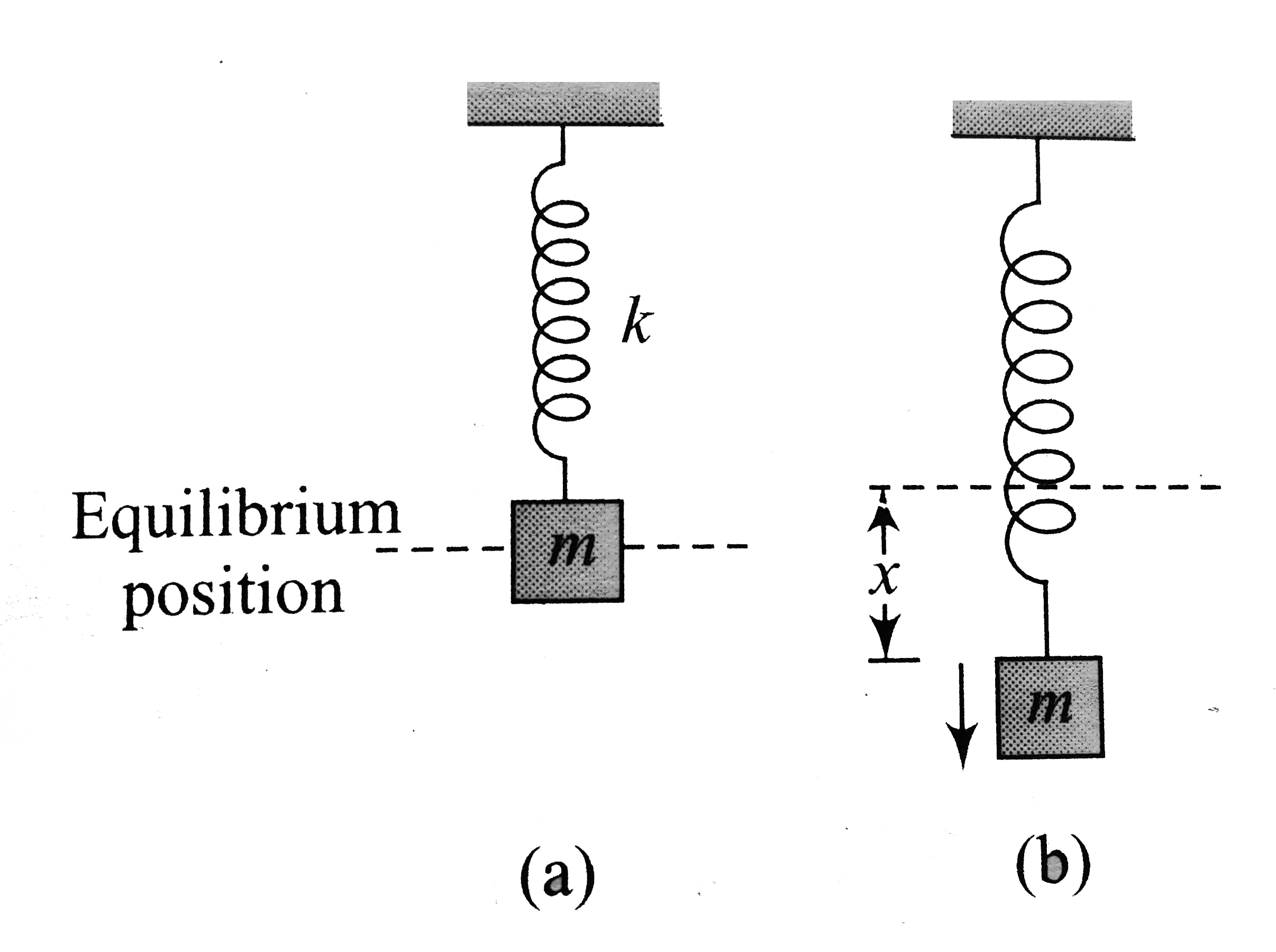
As shown in figure (b), when the block is pulled down by a distance x and throwndownward , it will start executting SHM. It will go further to a distance A (amplitude) from mean possion before returning back v at a displacement x from its mean position as
v=ω√A2−x2
or v2=km(A2−x2)
[as for spring block system ω=√km ltbr or A=√mv2k+x2)
Now timeof motion of bob can be obtained by circular motion representation of the respectiveSHM is shown in figure at t=0Att=0, block P is at a distance x force its mean position in downward direction and it is moving downwards so we can sider its corresponding circular motion partical in III quadrant as shown in reference angular velocity . We consider P will reach its mean position when partical P0 reaches position A by traversing an angle θ as shown in the figure.

Thus it will take a time gives as t=θω=⎛⎜
⎜⎝π−sin−1⎛⎜
⎜⎝x/A√km⎞⎟
⎟⎠
or t=√mk⎡⎢
⎢⎣π−sin−1⎛⎜
⎜⎝x√mv2k+x2⎞⎟
⎟⎠⎤⎥
⎥⎦
The maximum distance to which block will move from its initial position is A−x as it gas upto its lower exterme at a distance equal to its amplitude A after to mean position.
Topper's Solved these Questions
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Exercise 4.1
23 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Exercise 4.2
23 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single correct anwer type
14 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Interger
11 Videos
- MISCELLANEOUS KINEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS KINEMATICSEXERCISE - Interger type
3 Videos
Similar Questions
Figure shown a spring block system hanging in equilibrium. If a velocity v0 is imparted to the block in downwards direction . Find the amplitude of SHM of the block and the time after which it will reach a point at half the amplitude of block

View SolutionA spring block pendulum is shown in figure . The system is hanging in equilibrium. A bullet of mass m/2 moving at a speed u bites the block from downwards direction and gets embedded in it. Find the amplitude of oscillation of the block now.

View SolutionFigure shown a block P of mass m resting on a smooth horizontal ground, attached to one and a spring of force constant k in netural length. If another block od same mass and moving with a velocity u towards right is placed on the block on which stick to it due to friction ,find the time it will take to reach its exterme position. Also find the amplitude of oscillation of the combined mass 2m.

View Solution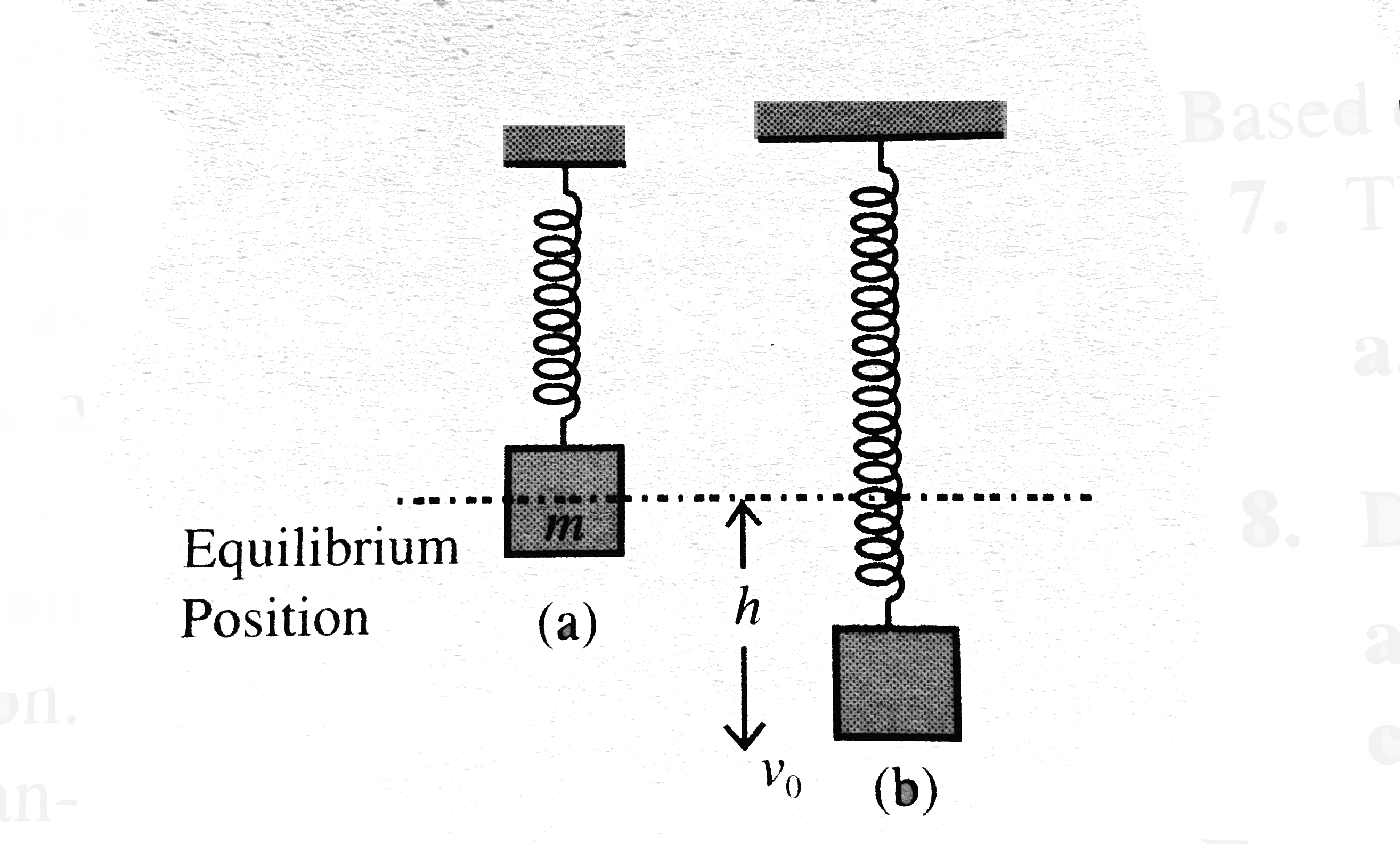
A block of mass m is connected to a spring constant k and is at rest in equilibrium as shown. Now, the block is Displacement by h below its equilibrium position and imparted a speed v0 towards down as shown in the Fig. As a result of the jerk, the block executes simple harmonic motion about its equilibrium position. Based on this information, answer the following question.
Q. Find the time taken by the block to cross the mean position for the first time.
View SolutionA block of mass m is moving with an initial velocity v0 towards a stationary spring of stiffness k attached to the wall as shown in figure

a. Find the maximum compression in the spring.
b. Is the work done by the spring negative or positive?
View SolutionFind the elastic potential energy stored in each spring shown in figure, when the block is in equilibrium. Also find the time period of vertical oscillation of the block.

View SolutionTow identical springs of spring constant k are attached to a block of mass m and to fixed supports as shown in figure. When the mass is displaced from equilibrum position by a distance x towards right, find the restoring force.

View Solution
System is shown in the figure and man is pulling the rope from both sides with constant speed u find the speed of the block.
View Solution
System is shown in the figure and man is pulling the rope from both sides with constant speed u find the speed of the block.
View Solution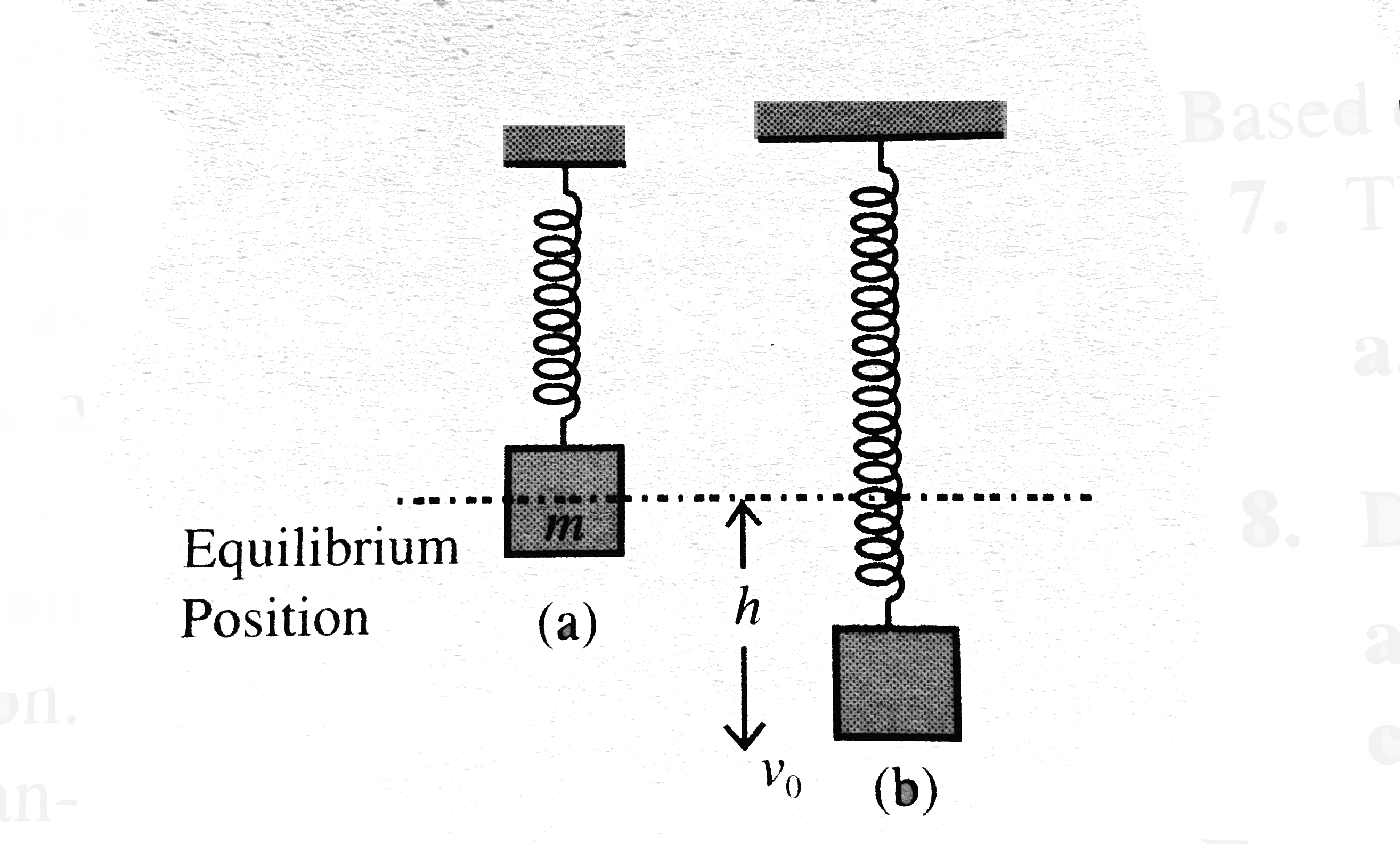
A block of mass m is connected to a spring constant k and is at rest in equilibrium as shown. Now, the block is Displacement by h below its equilibrium position and imparted a speed v0 towards down as shown in the Fig. As a result of the jerk, the block executes simple harmonic motion about its equilibrium position. Based on this information, answer the following question.
Q. Find the amplitude.
View Solution
