A thin film with index of refraction 1.33 coats a glass lens with index of refraction 1.50. Which of the following choices is the smallest film thickness that will not reflect light with wavelength 640 nm?
160 nm
240 nm
360 nm
480 nm
The correct Answer is:c
To solve the problem of finding the smallest film thickness that will not reflect light with a wavelength of 640 nm, we will use the concept of destructive interference in thin films. Here’s a step-by-step solution:
Step 1: Understand the Condition for Destructive Interference
For a thin film, destructive interference occurs when the path difference between the two reflected rays is equal to an odd multiple of half the wavelength. The condition can be expressed as:
2T=(m+12)λn
where:
- T = thickness of the film
- m = order of interference (0, 1, 2, ...)
- λ = wavelength of light in vacuum
- n = refractive index of the film
Step 2: Substitute Known Values
In this case:
- λ=640nm
- n=1.33 (the refractive index of the thin film)
Step 3: Calculate for the Smallest Value of m
We will first calculate for m=0:
2T=(0+12)640nm1.33
2T=12⋅6401.33
2T=6402.66
T=6405.32
T≈120.0nm
Step 4: Check for Validity of the Thickness
Since 120 nm is not among the options provided, we will check for the next value of m, which is m=1:
2T=(1+12)640nm1.33
2T=32⋅6401.33
2T=3×6402.66
T=3×6405.32
T≈360.0nm
Step 5: Conclusion
The smallest film thickness that will not reflect light with a wavelength of 640 nm is approximately 360 nm.
For a thin film, destructive interference occurs when the path difference between the two reflected rays is equal to an odd multiple of half the wavelength. The condition can be expressed as:
2T=(m+12)λn
where:
- T = thickness of the film
- m = order of interference (0, 1, 2, ...)
- λ = wavelength of light in vacuum
- n = refractive index of the film
In this case:
- λ=640nm
- n=1.33 (the refractive index of the thin film)
We will first calculate for m=0:
2T=(0+12)640nm1.33
2T=12⋅6401.33
2T=6402.66
T=6405.32
T≈120.0nm
Since 120 nm is not among the options provided, we will check for the next value of m, which is m=1:
2T=(1+12)640nm1.33
2T=32⋅6401.33
2T=3×6402.66
T=3×6405.32
T≈360.0nm
The smallest film thickness that will not reflect light with a wavelength of 640 nm is approximately 360 nm.
Topper's Solved these Questions
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Integer
Explore 3 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Archives
Explore 4 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Assertion- Reasoning
Explore 13 Videos
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:single correct Ansewer type
Explore 12 Videos
Similar Questions
A film with index of refraction 1.50 coats a glass lens with index of refraction 1.80. What is the minimum thickness of the thin film that will strongly reflect light with wavelength 600 nm?
View SolutionA thin film of thickness t and index of refractive 1.33 coats a glass with index of refraction 1.50.What is the least thickness t that will strong reflect light with wavelength 600nm incident normally?
View Solution
Knowledge Check
A parallel beam of sodium light of wavelength 6000Å is incident on a thin glass plate of μ=1.5, such that the angle of refraction in the plate is 60∘. The smallest thickness of the plate which will make it appear dark by reflected light is
A3926 Ã…B4353 Ã…C1396 Ã…D1921 Ã…
High-quality camera lenses are often coated to prevent reflection. A lens has an optical index of refraction of 1.72 and a coating with an optical index os refraction 1.31. For near normal incidence, the minimum thickness of the coating to prevent reflection for wavelength of 5.3×10−7m is
View SolutionA thin uniform film of refractive index 1.75 is placed on a sheet of glass of refractive index 1.5. At room temperature (20∘C) this film is just thick enoungh for light with wavelength 600 nm reflected off the top of the film to be canceled by light reflected from the top the glass. After the glass is placed in on oven and slowly heated to 170∘C, the film conceals reflected light with wavelength 606 nm. The coefficient of linear expansion of the film is (ignore any changes in the refractive index of the film due to the temperature change)
View SolutionA ray of light 10−9 second to cross a glass slab of refractive index 1.5. The thickness of the slab will be
View SolutionRefractive index of a thin soap film of a uniform thickness is 1.34. Find the smallest thickness of the film that gives in interference maximum in the reflected light when light of wavelength 5360Å fall at normal incidence.
View SolutionA material having an index of refraction of 1.30 is used as an antireflective coating on a piece of glass (n = 1.50). What should be the minimum thickness of this film in order to minimize reflection of 500 nm light?
View SolutionA ray of light is incident at an angle of 45∘ (ralative to the normal) on a thin film of a liquid with an index of refraction of √2. Assuming that the medium of both sides of the film is air, find the minimum thickness of the film required for constructive interference in the reflected light for sodium light of wavelenght 600 nm.
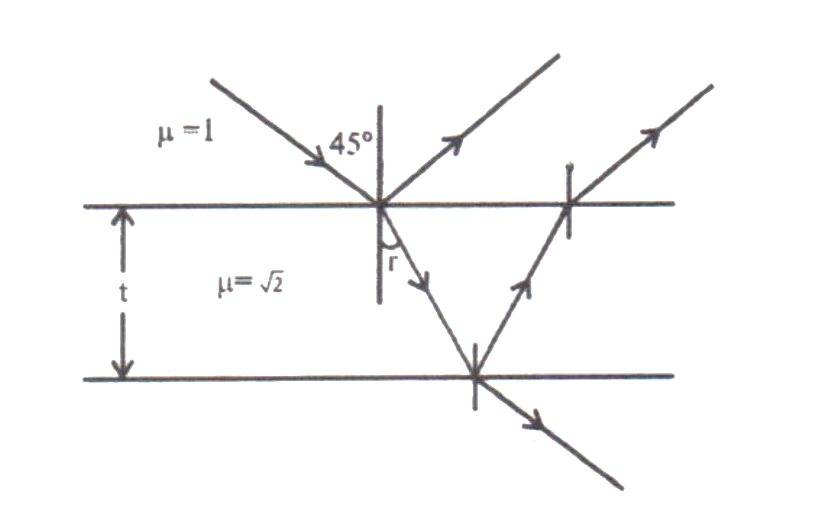
View SolutionA double - slit apparatus is immersed in a liquid of refractive index 1.33 it has slit separation of 1mm and distance between the plane of slits and screen 1.33 m the slits are illuminated by a parallel beam of light whose wavelength in air is 800 nm.
(i) Calculate the fringe width.
(ii) One of the slits of apparatus is covered by a thin glass sheet of refractive index 1.53 Find the smallest thickness of the sheet to bring the adjacent minima on the axis.
View Solution