Refractive index of a thin soap film of a uniform thickness is 1.34. Find the smallest thickness of the film that gives in interference maximum in the reflected light when light of wavelength 5360Å fall at normal incidence.
To find the smallest thickness of a thin soap film that gives an interference maximum in the reflected light, we can follow these steps:
Step 1: Understand the condition for constructive interference
For constructive interference in thin films, the condition is given by:
2μt=(n+12)λ
where:
- μ is the refractive index of the film,
- t is the thickness of the film,
- n is the order of interference (0, 1, 2, ...),
- λ is the wavelength of light in vacuum.
Step 2: Identify the parameters
From the problem, we have:
- Refractive index μ=1.34
- Wavelength λ=5360Å=5360×10−10m
Step 3: Find the smallest thickness for maximum interference
To find the smallest thickness t, we will use the lowest order of interference, which is n=0:
2μt=(0+12)λ
This simplifies to:
2μt=12λ
Step 4: Solve for thickness t
Rearranging the equation gives:
t=λ4μ
Step 5: Substitute the values
Now, substituting the values of λ and μ:
t=5360×10−104×1.34
Step 6: Calculate the thickness
Calculating the denominator:
4×1.34=5.36
Now substituting back:
t=5360×10−105.36
Calculating:
t≈53605.36×10−10≈1000×10−10m
t=1000Å
Final Answer
The smallest thickness of the soap film that gives an interference maximum in the reflected light is:
t=1000Å
---
For constructive interference in thin films, the condition is given by:
2μt=(n+12)λ
where:
- μ is the refractive index of the film,
- t is the thickness of the film,
- n is the order of interference (0, 1, 2, ...),
- λ is the wavelength of light in vacuum.
From the problem, we have:
- Refractive index μ=1.34
- Wavelength λ=5360Å=5360×10−10m
To find the smallest thickness t, we will use the lowest order of interference, which is n=0:
2μt=(0+12)λ
This simplifies to:
2μt=12λ
Rearranging the equation gives:
t=λ4μ
Now, substituting the values of λ and μ:
t=5360×10−104×1.34
Calculating the denominator:
4×1.34=5.36
Now substituting back:
t=5360×10−105.36
t≈53605.36×10−10≈1000×10−10m
t=1000Å
The smallest thickness of the soap film that gives an interference maximum in the reflected light is:
t=1000Å
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Exercise 2.2
22 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Subjective
11 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Solved Examples
10 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - single correct Ansewer type
12 Videos
Similar Questions
Refractive index of a thin soap film of a uniform thickness is 1.38. The smallest thickness of the film that gives an interference maxima in the reflected light when light of wavelength 5520 Å falls at normal incidence is
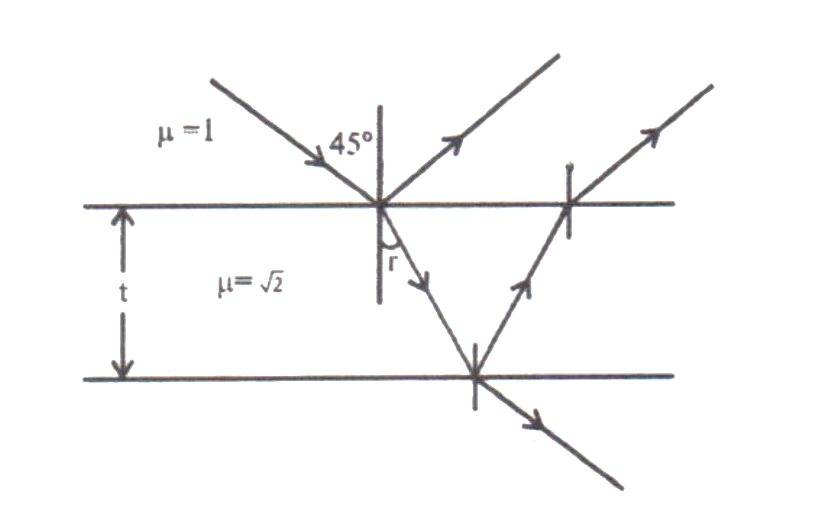
View SolutionA ray of light is incident at an angle of 45∘ (ralative to the normal) on a thin film of a liquid with an index of refraction of √2. Assuming that the medium of both sides of the film is air, find the minimum thickness of the film required for constructive interference in the reflected light for sodium light of wavelenght 600 nm.
View SolutionAn oil film covers the surface of a small pond. The refractive index of the oil is greater than that of water. At one point on the film, the film has the smallest non-zero thickness for which there will be destructive interference in the reflected light when infrared radiation with wavelength 800 nm is incident normal to the film. When this film is viewed at normal incidence at this same point, for what visible wavelengths, if any, will there be constructive interference? (Visible light has wavelengths between 400nm and 700 nm )
View SolutionA film with index of refraction 1.50 coats a glass lens with index of refraction 1.80. What is the minimum thickness of the thin film that will strongly reflect light with wavelength 600 nm?
View SolutionWhat is the minimum thickness of a thin film (μ=1.2) that results in constructive interference in the reflected light? If the film is illuminated with light whose wavelength in free space is λ=500nm ?
View SolutionA light of wavelength 5890Å falls normally on a thin air film. The minimum thickness of the film such that the film appears dark in reflected light is
View SolutionFind the minimum thickness of a film which will strongly reflect the light of wavelength 589 nm. The refractive index of the material of the film is 1.25.
View SolutionCalculate the minimum thickness of a soap bubble film (μ=1.33)that results in constructive interference in the reflected light if the film is illuminated with light whose wavelength in free space is λ=600nm.
View SolutionApproximate thickness of oil film to observe interference of light (due to which it looks coloured) is
View SolutionA thin film of thickness t and index of refractive 1.33 coats a glass with index of refraction 1.50.What is the least thickness t that will strong reflect light with wavelength 600nm incident normally?
View Solution
