A monochromatic light of λ=5000Å is incident on two identical slits separated by a distance of 5×10−4 m. The interference pattern is seen on a screen placed at a distance of 1 m from the plane of slits. A thin glass plate of thickness 1.5×10−6 m and refractive index μ=1.5 is placed between one of the slits and the screen . Find the intensity at the center of the screen.
The correct Answer is:0
To solve the problem step by step, we will follow the outlined process to find the intensity at the center of the screen when a thin glass plate is introduced in the path of one of the beams in a double-slit interference experiment.
Step 1: Identify Given Values
- Wavelength of light, λ=5000Å=5000×10−10m=5×10−7m
- Distance between the slits, d=5×10−4m
- Distance from the slits to the screen, D=1m
- Thickness of the glass plate, t=1.5×10−6m
- Refractive index of the glass plate, μ=1.5
Step 2: Calculate the Path Difference
When a glass plate is introduced in the path of one of the beams, the effective path difference Δx is given by:
Δx=(S2O−S1O)+(μ−1)t
Here, S2O is the path through the air, and S1O is the path through the glass. Since S1O does not pass through the glass, we can simplify this to:
Δx=0+(μ−1)t=(1.5−1)×1.5×10−6
Calculating this gives:
Δx=0.5×1.5×10−6=0.75×10−6m
Step 3: Calculate the Phase Difference
The phase difference ϕ corresponding to the path difference is given by:
ϕ=2πλΔx
Substituting the values:
ϕ=2π5×10−7×0.75×10−6
Calculating this gives:
ϕ=2π×0.755=1.5π5=0.3πradians
Step 4: Calculate the Resultant Intensity
The intensity at the center of the screen can be calculated using the formula:
I=Imaxcos2(ϕ2)
Since Imax for two coherent sources is typically 4I0 (where I0 is the intensity from one slit), we will use:
I=4I0cos2(0.3π2)
Calculating 0.3π2=0.15π:
cos(0.15π)≈cos(0.4712)≈0.891
Thus:
I=4I0(0.891)2≈4I0×0.792≈3.168I0
Step 5: Conclusion
The intensity at the center of the screen is:
I≈3.168I0
- Wavelength of light, λ=5000Å=5000×10−10m=5×10−7m
- Distance between the slits, d=5×10−4m
- Distance from the slits to the screen, D=1m
- Thickness of the glass plate, t=1.5×10−6m
- Refractive index of the glass plate, μ=1.5
When a glass plate is introduced in the path of one of the beams, the effective path difference Δx is given by:
Δx=(S2O−S1O)+(μ−1)t
Here, S2O is the path through the air, and S1O is the path through the glass. Since S1O does not pass through the glass, we can simplify this to:
Δx=0+(μ−1)t=(1.5−1)×1.5×10−6
Calculating this gives:
Δx=0.5×1.5×10−6=0.75×10−6m
The phase difference ϕ corresponding to the path difference is given by:
ϕ=2πλΔx
Substituting the values:
ϕ=2π5×10−7×0.75×10−6
Calculating this gives:
ϕ=2π×0.755=1.5π5=0.3πradians
The intensity at the center of the screen can be calculated using the formula:
I=Imaxcos2(ϕ2)
Since Imax for two coherent sources is typically 4I0 (where I0 is the intensity from one slit), we will use:
I=4I0cos2(0.3π2)
Calculating 0.3π2=0.15π:
cos(0.15π)≈cos(0.4712)≈0.891
Thus:
I=4I0(0.891)2≈4I0×0.792≈3.168I0
The intensity at the center of the screen is:
I≈3.168I0
Topper's Solved these Questions
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Integer
Explore 3 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Archives
Explore 4 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Assertion- Reasoning
Explore 13 Videos
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:single correct Ansewer type
Explore 12 Videos
Similar Questions
A monochromatic light of λ=500nm is incident on two identical slits separated by a distance of 5×10−4m. The interference pattern is seen on a screen placed at a distance of 1m from the plane of slits. A thin glass plate of thickness 1.5×10−6m and refractive index μ=1.5 is placed between one of the slits and the screen. Find the intensity at the center of the screen if the intensity is I0 in the absence of the plate. Also find the lateral shift of the central maxima and number of fringes crossed through center.
View SolutionLight of wavelength 5000A0 is incident on a slit. The first minimum of the diffraction pattern is observed to lie at a distance of 5 mm from the central maximum on a screen placed at a distance of 3 m from the slit. Then the width of the slit is
View SolutionTwo slits in YDSE are placed 1 mm from each other. Interference pattern is observed on a screen placed 1m from the plane of slits. What is the angular fringe width for a light of wavelength 400 nm
View SolutionTwo slits in YDSE are placed 2 millimeter from each other. Interference pattern is observed on a screen placed 2 m from the plane of slits. What is the fringe width for a light of wavelength 400 nm?
View SolutionIn Yonung's double-slit experiment, two slits which are separated by 1.2 mm are illuminated with a monochromatic light of wavelength 6000Å The interference pattern is observed on a screen placed at a distance of 1 m from the slits. Find the number of bright fringes formed over 1 cm width on the screen.
View SolutionA young's double slit apporatus is immersed in a liquid of refractive index 1.33.It has slit separation of 1 mm and interference pattern is observed on the screen at a distance 1.33 m from plane of slits.The wavelength in air is 6300Å
Calculate the fringe width.
View SolutionTwo slits at a distance of 1mm are illuminated by a light of wavelength 6.5×10−7m. The interference fringes are observed on a screen placed at a distance of 1m. The distance between third dark fringe and fifth bright fringe will be
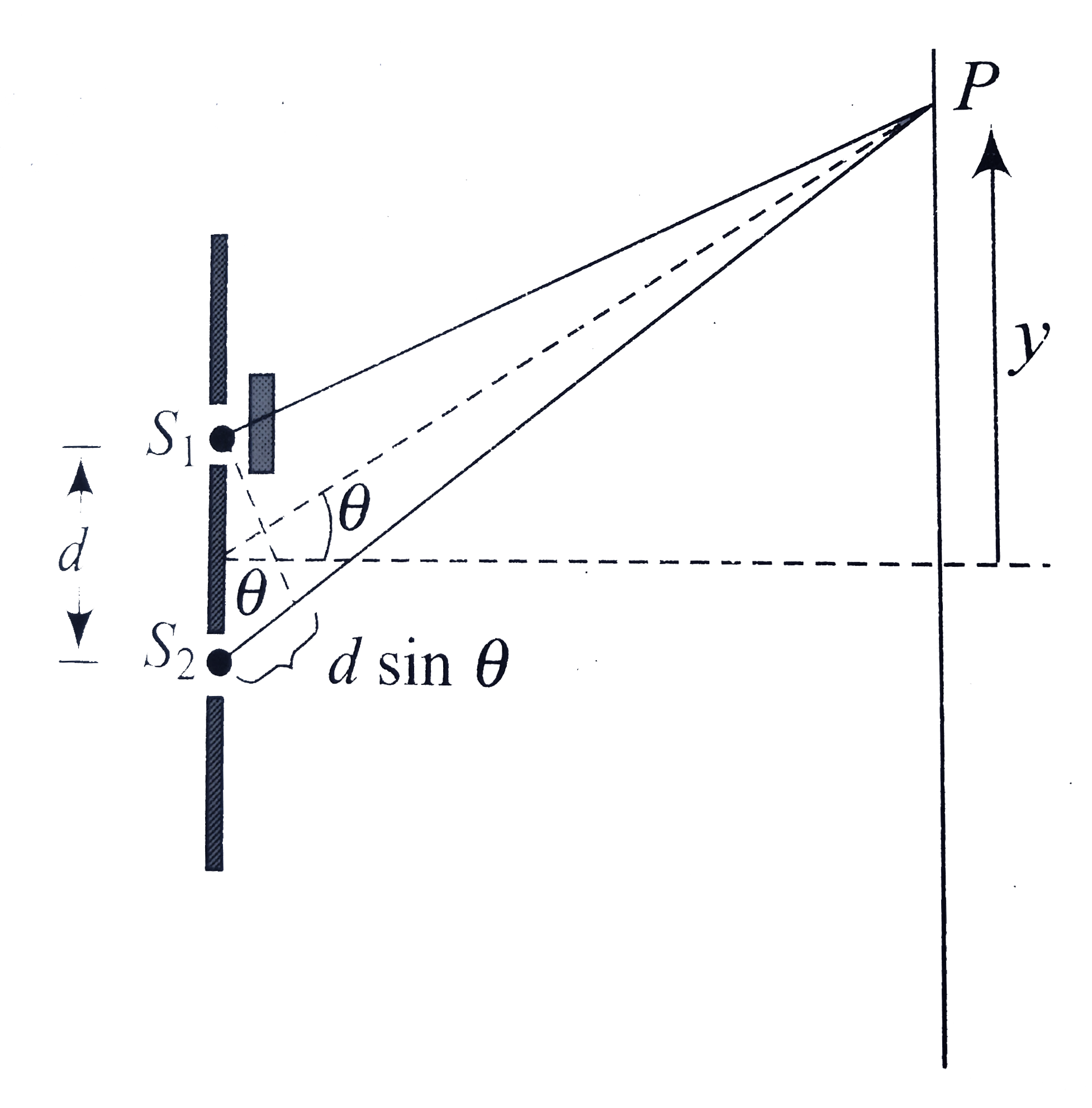
View SolutionIn YDSE, light of wavelength λ=5000Å is used, which emerges in phase from two a slits distance d=3×10−7m apart. A transparent sheet of thickness t=1.5×10(−7)m, refractive indes n=1.17, is placed over one of the slits. Where does the central maxima of the interference now appear from the center of the screen? (Find the value of y?)
View SolutionA plane monochromatic light wave falls normally on a diaphragm with two narrow slits separated by 2.5mm. The fringe pattern is formed on a screen 100 cm behind the diaphragm. By what distance will these fringes be displaced, when one of the slits is covered by a glass plate (μ=1.5) of thickness 10μm?
View SolutionFigure shows a YDSE setup having identical slits S1 and S2 with d =5 mm and D = 1 m. A monochromatic light of wavelength λ=6000Å is incident on the plane of slit due to which at screen centre O, an intensity I0 is produced with fringe pattern on both sides Now a thin transparent film of 11μm thickness and refractive index μ=2.1 is placed in front of slit S1 and now interference patten is observed again on screen.
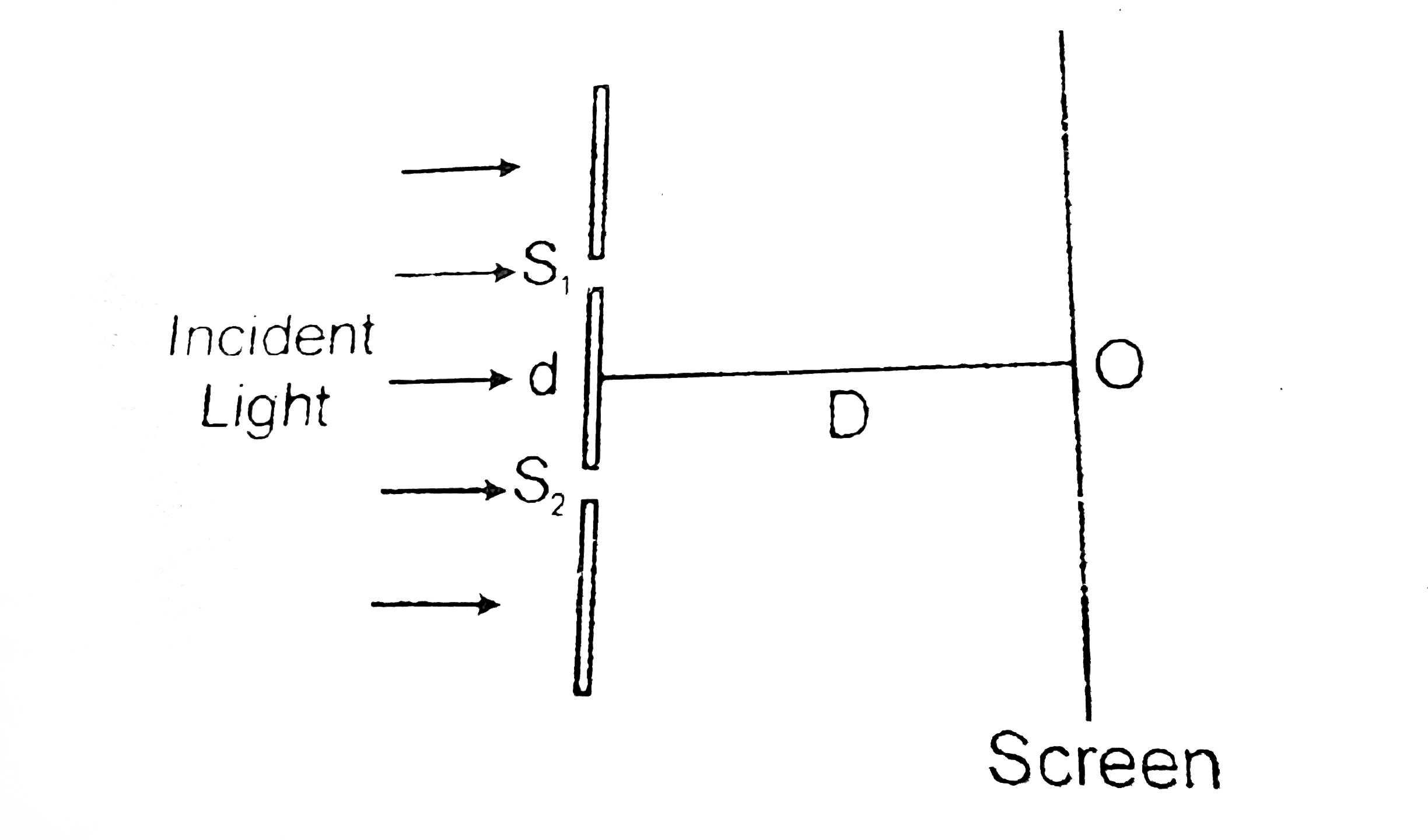
After placing the film of slit S1, the intensity at point O screen is :
View Solution
