A beaker containing water is placed on the platform of a spring balance. The balance reads 1.5kg. A stone of mass 0.5kg and density 104kg/m3 is immersed in water without touching the walls of the beaker. What will be the balance reading now?
2kg
2.5kg
1kg
3kg
The correct Answer is:A
To solve the problem step by step, we will analyze the situation before and after immersing the stone in the water.
Step 1: Understand the initial conditions
- The initial reading of the spring balance is given as 1.5 kg. This reading represents the weight of the beaker filled with water.
Step 2: Determine the properties of the stone
- The mass of the stone is given as 0.5 kg.
- The density of the stone is given as 104kg/m3.
Step 3: Calculate the volume of the stone
- The volume V of the stone can be calculated using the formula:
V=massdensity=0.5kg104kg/m3=5×10−5m3
Step 4: Calculate the buoyant force acting on the stone
- The buoyant force Fb acting on the stone when it is immersed in water can be calculated using Archimedes' principle:
Fb=density of water×V×g
where the density of water is approximately 103kg/m3 and g (acceleration due to gravity) is approximately 10m/s2.
Substituting the values:
Fb=103kg/m3×5×10−5m3×10m/s2=0.5N
Step 5: Calculate the new reading on the spring balance
- When the stone is immersed in the water, the spring balance will read the weight of the beaker with water plus the weight of the stone minus the buoyant force acting on the stone.
- The initial weight (reading) on the balance is:
W1=1.5kg
- The new reading N can be expressed as:
N=W1+weight of the stone−Fb
- The weight of the stone is:
Weight of the stone=mass×g=0.5kg×10m/s2=5N
- Since we are working in kg, we need to convert the buoyant force into kg equivalent:
Fb=0.5N10m/s2=0.05kg
- Now substituting the values:
N=1.5kg+0.5kg−0.05kg=1.5+0.5−0.05=1.95kg
Final Answer
The new reading on the spring balance after immersing the stone in water will be 1.95 kg.
- The initial reading of the spring balance is given as 1.5 kg. This reading represents the weight of the beaker filled with water.
- The mass of the stone is given as 0.5 kg.
- The density of the stone is given as 104kg/m3.
- The volume V of the stone can be calculated using the formula:
V=massdensity=0.5kg104kg/m3=5×10−5m3
- The buoyant force Fb acting on the stone when it is immersed in water can be calculated using Archimedes' principle:
Fb=density of water×V×g
where the density of water is approximately 103kg/m3 and g (acceleration due to gravity) is approximately 10m/s2.
Substituting the values:
Fb=103kg/m3×5×10−5m3×10m/s2=0.5N
- When the stone is immersed in the water, the spring balance will read the weight of the beaker with water plus the weight of the stone minus the buoyant force acting on the stone.
- The initial weight (reading) on the balance is:
W1=1.5kg
- The new reading N can be expressed as:
N=W1+weight of the stone−Fb
- The weight of the stone is:
Weight of the stone=mass×g=0.5kg×10m/s2=5N
- Since we are working in kg, we need to convert the buoyant force into kg equivalent:
Fb=0.5N10m/s2=0.05kg
N=1.5kg+0.5kg−0.05kg=1.5+0.5−0.05=1.95kg
The new reading on the spring balance after immersing the stone in water will be 1.95 kg.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Multipe Correct
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Assertion-Reasoning
8 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective
25 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
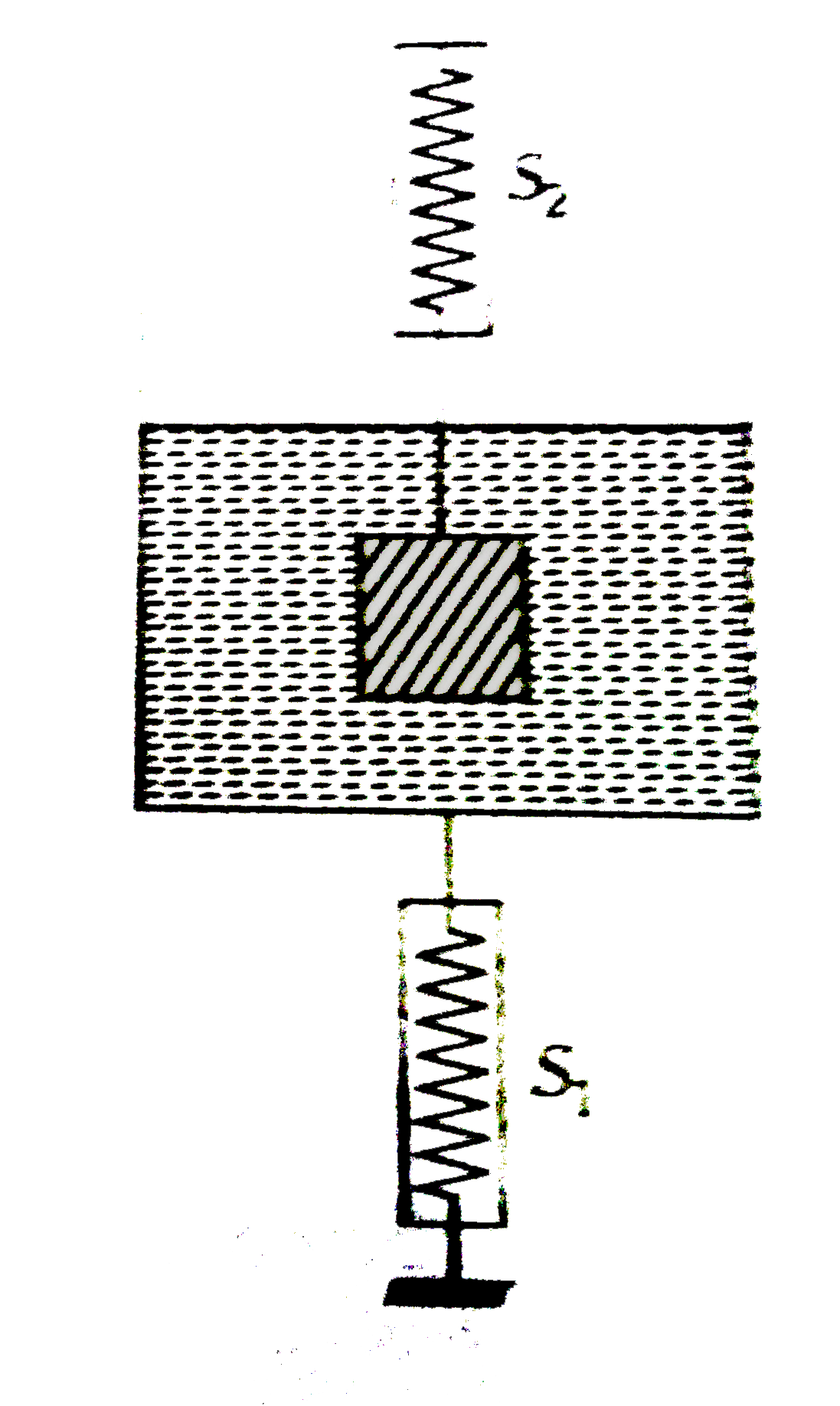
A beaker containing water is kept on a spring scale. The mass of water and beaker is 5 kg . A block of mass 2 kg and specific gravity 10 is suspended by means of thread from a spring balance as shown. The readings of scales S1 and S2 are respectively Take, g=10ms−2
View SolutionThe reading of a spring balance when a mass is weighed on it in air is 40 gm. When the mass is immersed in water, its reading is 20 gm. The specific gravity of the mass is
View SolutionA block hangs from a spring balance. The balance reads 40 N in air, 35 N when block is immersed in water and 25 N when block is immersed in another liquid. Find the density of block and other liquid.
View SolutionA beaker containing water is placed on the pan of a balance which shows a reading of M. A lump of sugar of mass m ad volume v is now suspend by a thread (from an independent support) in such a way that it is completely immersed in water without touching the beaker and without any overflow of water. How will the reading change as time passes on?
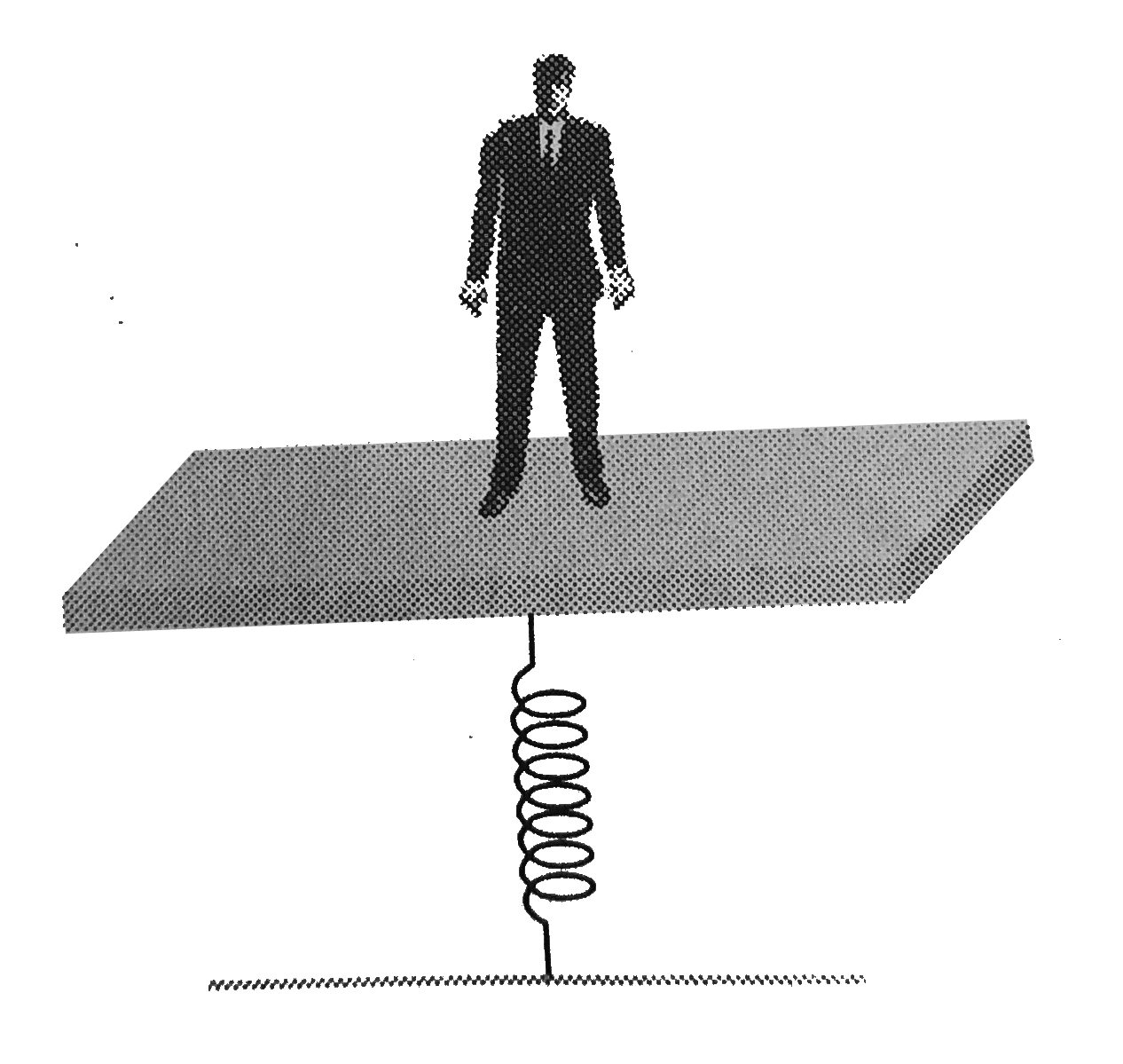
View SolutionA man weighing 60kg stands on the horizontal platform of a spring balance. The platform starts executing simple harmonic motion of amplitude 0.1m and frequency 2π. its which of the following statement is correct ?
View SolutionA 20N metal block is suspended by a spring balance. A beaker containing some water is placed on a weighing machine which reads 40N. The spring balance is now lowered so that the block gets immersed in the water. The spring balance now reads 16N. The reading of the weighing machine will be.
View SolutionThe mass of 1 litre of water is 1 kg. Find the density of water in kgm−3
View SolutionThe mass of 5 litre of water is 5 kg. Find the density of water in g cm−3
View SolutionA spring balance reads 10kg when a bucket of water is suspened from it . What will be the reading of the balance when an iron piece of mass 7.2kg suspended by a string is immersed with half its volume inside the water in the bucket? Relative density of iron is 7.2 a) 10 kg b) 10.5 kg c) 13.6 kg d) 17.2 kg
View SolutionAn iron block is suspended from a string and is then completely immersed in a container of water. The mass of iron is 1 kg and its density is 7200kg/m3 . What is the tension in the string before and after the iron block is immersed ?
View Solution
