A sphere of solid material of specific gravity 8 has a concentric spherical cavity and just sinks in water. The ratio of radius of cavity to that of outer radius of the sphere must be
71/32
51/32
91/32
31/32
The correct Answer is:A
To solve the problem, we need to find the ratio of the radius of the cavity (r) to the outer radius of the sphere (R) given that the sphere just sinks in water.
1. Understanding the Problem:
- We have a sphere with a specific gravity of 8, which means its density is 8 times that of water.
- The sphere has a concentric cavity and just sinks in water, indicating that the weight of the sphere equals the buoyant force acting on it.
2. Defining Variables:
- Let the radius of the cavity be r.
- Let the outer radius of the sphere be R.
3. Buoyant Force Calculation:
- The buoyant force Fb acting on the sphere is given by:
Fb=Density of water×Volume of the outer sphere×g
- The volume of the outer sphere is:
Vouter=43πR3
- Therefore, the buoyant force is:
Fb=ρwater×43πR3×g
- Since the specific gravity of water is 1, we can denote ρwater=1.
4. Weight of the Sphere Calculation:
- The weight W of the sphere can be expressed as:
W=Density of the sphere×Volume of the solid part×g
- The density of the sphere is ρsphere=8×ρwater=8.
- The volume of the solid part of the sphere is:
Vsolid=Vouter−Vcavity=43πR3−43πr3=43π(R3−r3)
- Therefore, the weight of the sphere is:
W=8×43π(R3−r3)×g
5. Setting Up the Equation:
- Since the sphere just sinks, we have:
W=Fb
- Substituting the expressions for W and Fb:
8×43π(R3−r3)×g=43πR3×g
6. Simplifying the Equation:
- Cancel out common terms 43πg:
8(R3−r3)=R3
- Rearranging gives:
8R3−8r3=R3
- This simplifies to:
7R3=8r3
7. Finding the Ratio:
- Dividing both sides by R3:
r3R3=78
- Taking the cube root of both sides:
rR=(78)1/3
Final Answer:
The ratio of the radius of the cavity to that of the outer radius of the sphere is:
rR=71/32
- We have a sphere with a specific gravity of 8, which means its density is 8 times that of water.
- The sphere has a concentric cavity and just sinks in water, indicating that the weight of the sphere equals the buoyant force acting on it.
- Let the radius of the cavity be r.
- Let the outer radius of the sphere be R.
- The buoyant force Fb acting on the sphere is given by:
Fb=Density of water×Volume of the outer sphere×g
- The volume of the outer sphere is:
Vouter=43πR3
- Therefore, the buoyant force is:
Fb=ρwater×43πR3×g
- Since the specific gravity of water is 1, we can denote ρwater=1.
- The weight W of the sphere can be expressed as:
W=Density of the sphere×Volume of the solid part×g
- The density of the sphere is ρsphere=8×ρwater=8.
- The volume of the solid part of the sphere is:
Vsolid=Vouter−Vcavity=43πR3−43πr3=43π(R3−r3)
- Therefore, the weight of the sphere is:
W=8×43π(R3−r3)×g
- Since the sphere just sinks, we have:
W=Fb
- Substituting the expressions for W and Fb:
8×43π(R3−r3)×g=43πR3×g
- Cancel out common terms 43πg:
8(R3−r3)=R3
- Rearranging gives:
8R3−8r3=R3
- This simplifies to:
7R3=8r3
- Dividing both sides by R3:
r3R3=78
- Taking the cube root of both sides:
rR=(78)1/3
The ratio of the radius of the cavity to that of the outer radius of the sphere is:
rR=71/32
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Multipe Correct
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Assertion-Reasoning
8 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective
25 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A solid ball of metal has a concentric spherical cavity within it. If the ball is heated, the volume of the cavity will
View SolutionA sphere of radius R and made of material of relative density σ has a concentric cavity of radius r. It just floats when placed in a tank full of water. The value of the ratio R/r will be
View SolutionA solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distanace R/4 from the centre of sphere. The gravitational force on mass m is
View SolutionA solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distance R/4 from the centre of sphere. The gravitational force on mass m is
View SolutionA hemi-spherical cavity is created in solid sphere (of radius 2R) as shown in the figure. Then

View SolutionA solid sphere of radius R has a spherical cavity of radius r as shown in the figure. If the volume charge density σ is uniformly distributed over the whole volume of the sphere, then electric field strength at the centre of the sphere will be
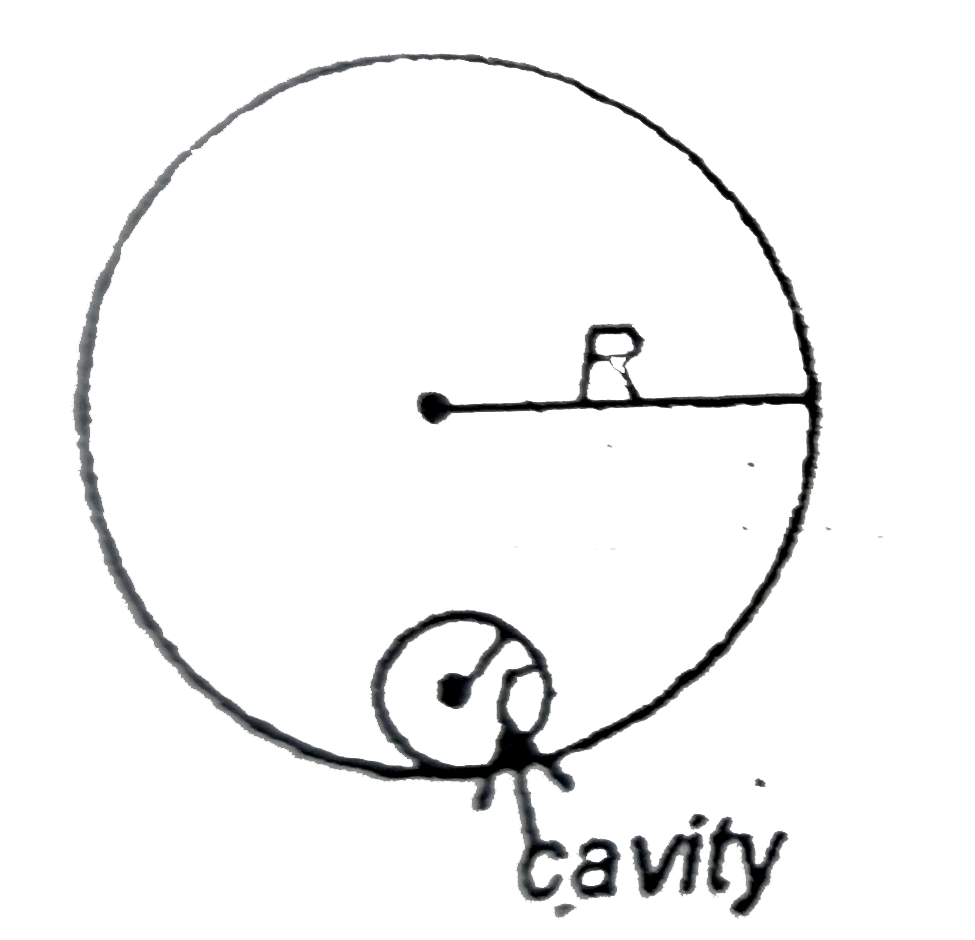
View SolutionA sphere of radius 2R and mas M has a spherical cavity of radius R as shown in the figure. Find the value of gravitational field at a point P at a distance of 6R from centre of the sphere.

View SolutionA solid spherical conductor of radius R has a spherical cavity of radius at its centre. A charge is kept at the centre. The charge at the inner surface, outer surface are respectively.
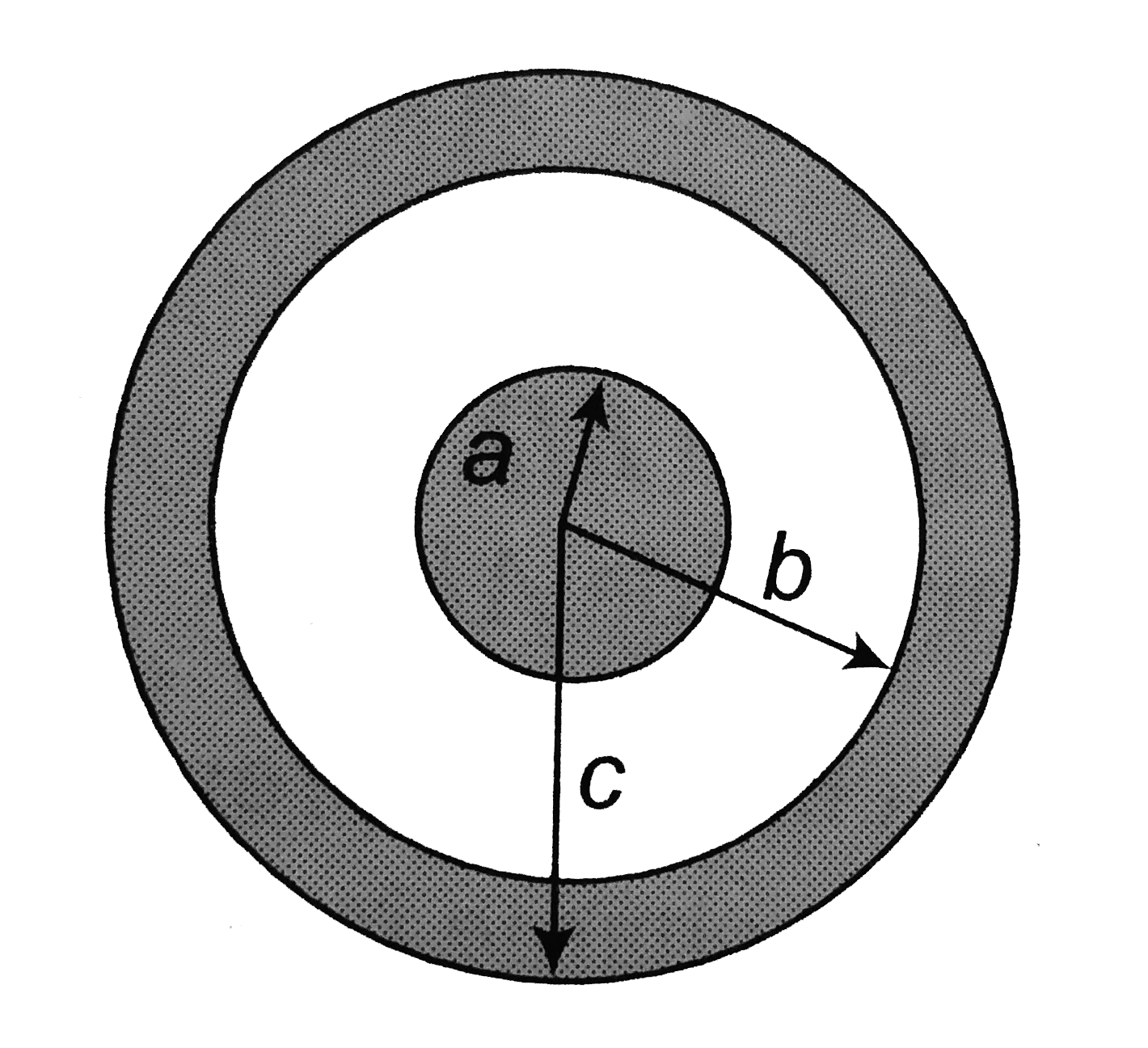
View SolutionA solid conducting sphere of radius a has a net positive charge 2Q. A conducting spherical shell of inner radius b and outer radius c is concentric with the solid sphere and has a net charge −Q. The surface charge density on the inner and outer surfaces of the spherical shell will be
View SolutionA sphere of radius R has a uniform volume density ρ. A spherical cavity of radius b, whose center lies at →a, is removed from the sphere.
i. Find the electric field at any point inside the spherical cavity.
ii. Find the electric field outside the cavity
(a) at points inside the large sphere but outside the cavity and
(b) at points outside the large sphere.

View Solution
